Article contents
Investigation of species-mass diffusion in binary-species boundary layers at high pressure using direct numerical simulations
Published online by Cambridge University Press: 06 October 2021
Abstract
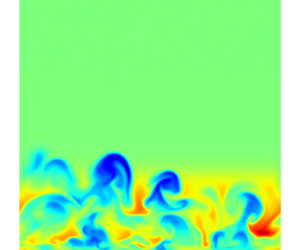
Direct numerical simulations of single-species and binary-species temporal boundary layers at high pressure are performed with special attention to species-mass diffusion. The working fluids are nitrogen or a mixture of nitrogen and methane. Mean profiles and turbulent fluctuations of mass fraction show that their qualitative characteristics are different from those of streamwise velocity and temperature, due to the different boundary conditions. In a wall-parallel plane near the wall, the streamwise velocity and temperature have streaky patterns and the fields are similar. However, the mass fraction field at the same location is different from the streamwise velocity and temperature fields indicating that species-mass diffusion is not similar to the momentum and thermal diffusion. In contrast, at the centre and near the edge of the boundary layer, the mass fraction and temperature fields have almost the same pattern, indicating that the similarity between thermal and species-mass diffusion holds away from the wall. The lack of similarity near the wall is traced to the Soret effect that induces a temperature-gradient-dependent species-mass flux. As a result, a new phenomenon has been identified for a non-isothermal binary-species system – uphill diffusion, which in its classical isothermal definition can only occur for three or more species. A quadrant analysis for the turbulent mass flux reveals that near the wall the Soret effect enhances the negative contributions of the quadrants. Due to the enhancement of the negative contributions, small species-concentration fluid tends to be trapped near the wall.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 8
- Cited by



