Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-05T02:51:32.217Z Has data issue: false hasContentIssue false
  • English
  • Français

Interactions between aquatic plants and turbulent flow: a field study using stereoscopic PIV

Published online by Cambridge University Press:  05 September 2013

S. M. Cameron ,
V. I. Nikora ,
I. Albayrak ,
O. Miler ,
M. Stewart  and
F. Siniscalchi
S. M. Cameron*
Affiliation:
School of Engineering, University of Aberdeen, Kings College, Aberdeen AB24 3UE, UK
V. I. Nikora
Affiliation:
School of Engineering, University of Aberdeen, Kings College, Aberdeen AB24 3UE, UK
I. Albayrak
Affiliation:
School of Engineering, University of Aberdeen, Kings College, Aberdeen AB24 3UE, UK
O. Miler
Affiliation:
School of Engineering, University of Aberdeen, Kings College, Aberdeen AB24 3UE, UK
M. Stewart
Affiliation:
School of Engineering, University of Aberdeen, Kings College, Aberdeen AB24 3UE, UK
F. Siniscalchi
Affiliation:
School of Engineering, University of Aberdeen, Kings College, Aberdeen AB24 3UE, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A stereoscopic particle image velocimetry (PIV) system for use in shallow (${\sim }$0.5 m deep) rivers was developed and deployed in the Urie River, Scotland, to study the interactions between turbulent flow and a Ranunculus penicillatus plant patch in its native environment. Statistical moments of the velocity field were calculated utilizing a new method of reducing the contribution of measurement noise, based on the measurement redundancy inherent in the stereoscopic PIV method. Reynolds normal and shear stresses, their budget terms, and higher-order moments of the velocity probability distribution in the wake of the plant patch were found to be dominated by the presence of a free shear layer induced by the plant drag. Plant motion, estimated from the PIV images, was characterized by travelling waves that propagate along the plant with a velocity similar to the eddy convection velocity, suggesting a direct coupling between turbulence and the plant motion. The characteristic frequency of the plant velocity fluctuations (${\sim }$1 Hz) may suggest that the plant motion is dominated by large eddies with scale similar to the flow depth or plant length. Plant and fluid velocity fluctuations were, in contrast, found to be strongly correlated only over a narrow (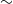${\sim }$30 mm) elevation range above the top of the plant, supporting a contribution of the shear layer turbulence to the plant motion. Many aspects of flow–aquatic plant interactions remain to be clarified, and the newly developed stereoscopic field PIV system should prove valuable in future studies.

JFM classification

Geophysical and Geological Flows: River dynamics Turbulent Flows: Shear layer turbulence
Type
Papers
Information
Journal of Fluid Mechanics , Volume 732 , 10 October 2013 , pp. 345 - 372
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Albayrak, I., Nikora, V., Miler, O. & O’Hare, M. 2012 Flow–plant interactions at a leaf scale: effects of leaf shape, serration, roughness and flexural rigidity. Aquat. Sci. 74 (2), 267–286.CrossRefGoogle Scholar
Astarita, T. 2007 Analysis of weighting windows for image deformation methods in PIV. Exp. Fluids 43 (6), 859–872.CrossRefGoogle Scholar
Balachandar, R., Blakely, D., Tachie, M. & Putz, G. 2001 A study on turbulent boundary layers on a smooth flat plate in an open channel. Trans. ASME: J. Fluids Engng 123 (2), 394–400.Google Scholar
Bornette, G. & Puijalon, S. 2011 Response of aquatic plants to abiotic factors: a review. Aquat. Sci. 73 (1), 1–14.Google Scholar
Calluaud, D. & David, L. 2004 Stereoscopic particle image velocimetry measurements of the flow around a surface-mounted block. Exp. Fluids 36 (1), 53–61.Google Scholar
Cameron, S. M. 2011 PIV algorithms for open-channel turbulence research: accuracy, resolution and limitations. J. Hydro-environ. Res. 5 (4), 247–262.CrossRefGoogle Scholar
Cantwell, B. & Coles, D. 1983 Experimental study of entrainment and transport in the turbulent near wake of a circular cylinder. J. Fluid Mech. 136, 321–374.CrossRefGoogle Scholar
Davidson, P. A. 2004 Turbulence: An Introduction for Scientists and Engineers. Oxford University Press.Google Scholar
Folkard, A. M. 2005 Hydrodynamics of model Posidonia oceanica patches in shallow water. Limnol. Oceanogr. 50 (5), 1592–1600.CrossRefGoogle Scholar
Folkard, A. M. 2011a Flow regimes in gaps within stands of flexible vegetation: laboratory flume simulations. Environ. Fluid Mech. 11 (3), 289–306.CrossRefGoogle Scholar
Folkard, A. M. 2011b Vegetated flows in their environmental context: a review. Proc. Inst. Civil Engrs: Engng Comput. Mech. 164 (1), 3–24.Google Scholar
Garcia, C. M., Jackson, P. R. & Garcia, M. H. 2006 Confidence intervals in the determination of turbulence parameters. Exp. Fluids 40 (4), 514–522.CrossRefGoogle Scholar
Ghisalberti, M. & Nepf, H. M. 2002 Mixing layers and coherent structures in vegetated aquatic flows. J. Geophys. Res. C: Oceans 107 (2), 3–1.Google Scholar
Graff, K. F. 1991 Wave Motion in Elastic Solids. Dover.Google Scholar
Green, J. C. 2005 Velocity and turbulence distribution around lotic macrophytes. Aquat. Ecol. 39 (1-2), 1–10.CrossRefGoogle Scholar
Hurther, D. & Lemmin, U. 2001 A correction method for turbulence measurements with a 3D acoustic doppler velocity profiler. J. Atmos. Ocean. Technol. 18 (3), 446–458.2.0.CO;2>CrossRefGoogle Scholar
Hussein, H. J. & Martinuzzi, R. J. 1996 Energy balance for turbulent flow around a surface mounted cube placed in a channel. Phys. Fluids 8 (3), 764–780.CrossRefGoogle Scholar
Katija, K. & Dabiri, J. O. 2008 In situ field measurements of aquatic animal–fluid interactions using a self-contained underwater velocimetry apparatus (SCUVA). Limnol. Oceanogr.: Meth. 6 (April), 162–171.Google Scholar
Koehl, M. A. R. & Alberte, R. S. 1988 Flow, flapping, and photosynthesis of nereocystis leutkeana: a functional comparison of undulate and flat blade morphologies. Mar. Biol. 99 (3), 435–444.Google Scholar
de Langre, E. 2008 Effects of wind on plants. Annu. Rev. Fluid Mech. 40, 141–168.Google Scholar
de Langre, E., Païdoussis, M. P., Doaré, O. & Modarres-Sadeghi, Y. 2007 Flutter of long flexible cylinders in axial flow. J. Fluid Mech. 571, 371–389.CrossRefGoogle Scholar
Liao, Q., Bootsma, H. A., Xiao, J., Klump, J. V., Hume, A., Long, M. H. & Berg, P. 2009 Development of an in situ underwater particle image velocimetry (UWPIV) system. Limnol. Oceanogr.: Meth. 7 (February), 169–184.Google Scholar
Lomb, N. R. 1976 Least-squares frequency analysis of unequally spaced data. Astrophys. Space Sci. 39 (2), 447–462.CrossRefGoogle Scholar
Maas, H. G. 1996 Contributions of digital photogrammetry to 3D PTV. In Three-dimensional Velocity and Vorticity Measuring and Image Analysis Techniques (ed. Dracos, T.), pp. 191–208. Kluwer.CrossRefGoogle Scholar
Mansour, N. N., Kim, J. & Moin, P. 1988 Reynolds-stress and dissipation-rate budgets in a turbulent channel flow. J. Fluid Mech. 194, 15–44.CrossRefGoogle Scholar
Naden, P., Rameshwaran, P., Mountford, O. & Robertson, C. 2006 The influence of macrophyte growth, typical of eutrophic conditions, on river flow velocities and turbulence production. Hydrol. Process. 20 (18), 3915–3938.CrossRefGoogle Scholar
Naudascher, E. & Rockwell, D. 1994 Flow-Induced Vibrations: An Engineering Guide. Dover.Google Scholar
Nepf, H. M. 2012 Hydrodynamics of vegetated channels. J. Hydraul. Res. 50 (3), 262–279.CrossRefGoogle Scholar
Nezu, I. & Nakagawa, H. 1993 Turbulence in Open Channel Flows. Balkema.Google Scholar
Nezu, I. & Sanjou, M. 2008 Turburence structure and coherent motion in vegetated canopy open-channel flows. J. Hydro-environ. Res. 2 (2), 62–90.Google Scholar
Nikora, V. 2010 Hydrodynamics of aquatic ecosystems: an interface between ecology, biomechanics and environmental fluid mechanics. River Res. Appl. 26 (4), 367–384.CrossRefGoogle Scholar
Nikora, V., Cameron, S., Albayrak, I., Miler, O., Nikora, N., Siniscalchi, F., Stewart, M. & O’Hare, M. 2012 Flow-biota interactions in aquatic systems: scales, mechanisms, and challenges. In Environmental Fluid Mecahanics: Memorial Volume in Honour of Prof. Gerhard H. Jirka (ed. Rodi, W. & Uhlmann, M.), pp. 217–235. CRC Press.Google Scholar
Nikora, V. & Goring, D. G. 2000a Eddy convection velocity and Taylor’s hypothesis of ‘frozen’ turbulence in a rough-bed open-channel flow. J. Hydrosci. Hydraul. Engng 18 (2), 75–91.Google Scholar
Nikora, V. & Goring, D. 2000b Flow turbulence over fixed and weakly mobile gravel beds. J. Hydraul. Engng 126 (9), 679–690.CrossRefGoogle Scholar
Nikora, N. & Nikora, V 2007 A viscous drag concept for flow resistance in vegetated channels. In Harmonizing the Demands of Art and Nature in Hydraulics: Proceedings of 32nd Congress of IAHR, Venice, Italy (CD-ROM).Google Scholar
Nimmo Smith, W. A. M., Atsavapranee, P., Katz, J. & Osborn, T. R. 2002 PIV measurements in the bottom boundary layer of the coastal ocean. Exp. Fluids 33 (6), 962–971.CrossRefGoogle Scholar
Nobach, H. & Bodenschatz, E. 2009 Limitations of accuracy in PIV due to individual variations of particle image intensities. Exp. Fluids 47 (1), 27–38.CrossRefGoogle Scholar
O’Hare, M. T., Hutchinson, K. A. & Clarke, R. T. 2007 The drag and reconfiguration experienced by five macrophytes from a lowland river. Aquat. Bot. 86 (3), 253–259.CrossRefGoogle Scholar
Païdoussis, M. P. 2004 Fluid-Structure Interactions: Slender Structures and Axial Flow, vol. 2. Elsevier.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.CrossRefGoogle Scholar
Prasad, A. K. 2000 Stereoscopic particle image velocimetry. Exp. Fluids 29 (2), 103–116.Google Scholar
Raffel, M., Willert, C. E., Wereley, S. T. & Kompenhans, J. 2007 Particle Image Velocimetry: A Practical Guide. Springer.Google Scholar
Raupach, M. R., Finnigan, J. J. & Brunet, Y. 1996 Coherent eddies and turbulence in vegetation canopies: the mixing-layer analogy. Boundary Layer Meteorol. 78 (3-4), 351–382.Google Scholar
Sand-Jensen, K. 2003 Drag and reconfiguration of freshwater macrophytes. Freshwat. Biol. 48 (2), 271–283.CrossRefGoogle Scholar
Sand-Jensen, K. & Mebus, J. R. 1996 Fine-scale patterns of water velocity within macrophyte patches in streams. Oikos 76 (1), 169–180.Google Scholar
Scargle, J. D. 1982 Studies in astronomical time series analysis. II. Statistical aspects of spectral analysis of unevenly spaced data. Astrophys. J. 263 (2), 835–853.Google Scholar
Shaw, R. H., Brunet, Y., Finnigan, J. J. & Raupach, M. R. 1995 A wind tunnel study of air flow in waving wheat: two-point velocity statistics. Boundary Layer Meteorol. 76 (4), 349–376.CrossRefGoogle Scholar
Siniscalchi, F. & Nikora, V. I. 2012 Flow–plant interactions in open-channel flows: a comparative analysis of five freshwater plant species. Water Resour. Res. 48 (5).CrossRefGoogle Scholar
Siniscalchi, F. & Nikora, V. I. 2013 Dynamic reconfiguration of aquatic plants and its interrelations with upstream turbulence and drag forces. J. Hydraul. Res. 51 (1).CrossRefGoogle Scholar
Siniscalchi, F., Nikora, V. I. & Aberle, J. 2012 Plant patch hydrodynamics in streams: mean flow, turbulence, and drag forces. Water Resour. Res. 48 (1).Google Scholar
Sukhodolova, T. A. & Sukhodolov, A. N. 2012 Vegetated mixing layer around a finite-size patch of submerged plants: 1. Theory and field experiments. Water Resour. Res. 48 (10).CrossRefGoogle Scholar
Tritico, H. M., Cotel, A. J. & Clarke, J. N. 2007 Development, testing and demonstration of a portable submersible miniature particle imaging velocimetry device. Meas. Sci. Technol. 18 (8), 2555–2562.CrossRefGoogle Scholar
Uberoi, M. S. & Freymuth, P. 1970 Turbulent energy balance and spectra of the axisymmetric wake. Phys. Fluids 13 (9), 2205–2210.CrossRefGoogle Scholar
Vogel, S. 1994 Life in Moving Fluids: The Physical Biology of Flow. Princeton University Press.Google Scholar
Wernet, M. P. 2005 Symmetric phase only filtering: a new paradigm for DPIV data processing. Meas. Sci. Technol. 16 (3), 601–618.Google Scholar
Wieneke, B. 2005 Stereo-PIV using self-calibration on particle images. Exp. Fluids 39 (2), 267–280.Google Scholar
Willert, C. 1997 Stereoscopic digital particle image velocimetry for application in wind tunnel flows. Meas. Sci. Technol. 8 (12), 1465–1479.Google Scholar
Wygnanski, I. & Fiedler, H. E. 1970 Two-dimensional mixing region. J. Fluid Mech. 41 (2), 327–361.Google Scholar
Zhu, W., Van Hout, R., Luznik, L., Kang, H. S., Katz, J. & Meneveau, C. 2006 A comparison of PIV measurements of canopy turbulence performed in the field and in a wind tunnel model. Exp. Fluids 41 (2), 309–318.CrossRefGoogle Scholar

Cameron et al. supplementary movie

Flow and plant velocity fluctuations measured around a Ranunculus penicillatus plant patch in the Urie river using stereoscopic PIV.

Download Cameron et al. supplementary movie(Video)
Video 9.4 MB