Article contents
Inertial versus baroclinic instability of the Bickley jet in continuously stratified rotating fluid
Published online by Cambridge University Press: 04 March 2014
Abstract
The paper contains a detailed study of the inertial instability of a barotropic Bickley jet on the 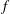 $f$-plane in the continuously stratified primitive equations model, and a comparison of this essentially ageostrophic instability with the classical baroclinic one. Analytical and numerical investigation of the linear stability of the jet in the long-wave sector is performed for a range of Rossby and Burger numbers. The major results are that: (1) the standard symmetric inertial instability, appearing at high enough Rossby numbers, turns out to be the infinite-wavelength limit of an asymmetric inertial instability, this latter having the highest growth rate for a large range of vertical wavenumbers; (2) inertial instability coexists with the standard baroclinic instability, which becomes dominant at small Rossby numbers. Nonlinear saturation of the inertial instability of the jet with a superimposed random small-amplitude perturbation is then studied, using the Weather Research and Forecast model. It is shown that at first stages the inertial instability dominates. It is localized near the maximum of the anticyclonic shear and is associated with the highest attainable value of the vertical wavenumber. The saturation of the inertial instability leads to the homogenization of the geostrophic momentum in the unstable region. At later stages, another baroclinic instability develops, characterized by lower values of the vertical wavenumber. This instability saturates by forming large-scale vortices downstream. It is identified as the leading instability of a marginally inertially stable jet resulting from the initial one through homogenization of the geostrophic momentum. The rough scenario of the evolution of essentially ageostrophic jets is, thus, as follows: the inertial instability rapidly saturates and baroclinic instability takes over. It is shown that reorganization of the flow due to developing instabilities is an efficient source of inertia-gravity waves.
$f$-plane in the continuously stratified primitive equations model, and a comparison of this essentially ageostrophic instability with the classical baroclinic one. Analytical and numerical investigation of the linear stability of the jet in the long-wave sector is performed for a range of Rossby and Burger numbers. The major results are that: (1) the standard symmetric inertial instability, appearing at high enough Rossby numbers, turns out to be the infinite-wavelength limit of an asymmetric inertial instability, this latter having the highest growth rate for a large range of vertical wavenumbers; (2) inertial instability coexists with the standard baroclinic instability, which becomes dominant at small Rossby numbers. Nonlinear saturation of the inertial instability of the jet with a superimposed random small-amplitude perturbation is then studied, using the Weather Research and Forecast model. It is shown that at first stages the inertial instability dominates. It is localized near the maximum of the anticyclonic shear and is associated with the highest attainable value of the vertical wavenumber. The saturation of the inertial instability leads to the homogenization of the geostrophic momentum in the unstable region. At later stages, another baroclinic instability develops, characterized by lower values of the vertical wavenumber. This instability saturates by forming large-scale vortices downstream. It is identified as the leading instability of a marginally inertially stable jet resulting from the initial one through homogenization of the geostrophic momentum. The rough scenario of the evolution of essentially ageostrophic jets is, thus, as follows: the inertial instability rapidly saturates and baroclinic instability takes over. It is shown that reorganization of the flow due to developing instabilities is an efficient source of inertia-gravity waves.
- Type
- Papers
- Information
- Copyright
- © 2014 Cambridge University Press
References
- 17
- Cited by


