Article contents
Inertial focusing of non-neutrally buoyant spherical particles in curved microfluidic ducts
Published online by Cambridge University Press: 04 September 2020
Abstract
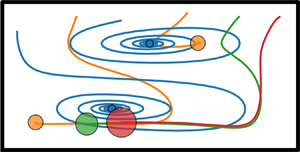
We examine the effect of gravity and (rotational) inertia on the inertial focusing of spherical non-neutrally buoyant particles suspended in flow through curved microfluidic ducts. In the neutrally buoyant case, examined in Harding et al. (J. Fluid Mech., vol. 875, 2019, pp. 1–43), the gravitational contribution to the force on the particle is exactly zero and the net effect of centrifugal and centripetal forces (due to the motion around the curved duct) is negligible. Inertial lift force and drag from the secondary fluid flow vortices interact and lead to focusing behaviour which is sensitive to the bend radius of the device and the particle size (each measured relative to the height of the cross-section). In the case of non-neutrally buoyant particles the behaviour becomes more complex with the two additional perturbing forces. The gravitational force, relative to the inertial lift force, scales with the inverse square of the flow velocity, making it a potentially important factor for devices operating at low flow rates with a suspension of non-neutrally buoyant particles. In contrast, the net centripetal/centrifugal force scales with the inverse of the bend radius, similar to the drag force from the secondary flow. We examine how these forces perturb the stable equilibria within the cross-sectional plane to which neutrally buoyant particles ultimately migrate.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
REFERENCES
- 9
- Cited by



