Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Liu, Y.
and
Guan, Y. K.
2021.
Large eddy simulation of the turbulent mixing at an oblique interface induced by non-classical planar shock waves.
AIP Advances,
Vol. 11,
Issue. 4,
Hopkins, Keill J.
Porat, Hadas
McIntyre, Timothy J.
Wheatley, Vincent
and
Veeraragavan, Ananthanarayanan
2021.
Measurements and analysis of hypersonic tripped boundary layer turbulence.
Experiments in Fluids,
Vol. 62,
Issue. 8,
Exposito, D.
Gai, S.L.
and
Neely, A.J.
2021.
Wall temperature and bluntness effects on hypersonic laminar separation at a compression corner.
Journal of Fluid Mechanics,
Vol. 922,
Issue. ,
Helm, Clara M.
and
Martín, M. P.
2022.
Large eddy simulation of two separated hypersonic shock/turbulent boundary layer interactions.
Physical Review Fluids,
Vol. 7,
Issue. 7,
Chang, Eric Won Keun
Chan, Wilson Y.K.
McIntyre, Timothy J.
and
Veeraragavan, Ananthanarayanan
2022.
Hypersonic shock impingement studies on a flat plate: flow separation of laminar boundary layers.
Journal of Fluid Mechanics,
Vol. 951,
Issue. ,
Chiapparino, Giuseppe
and
Stemmer, Christian
2022.
Numerical investigation of a Mach 6 hypersonic laminar flow on two-dimensional cold-wall compression corners with controlled surface roughness.
International Journal of Heat and Fluid Flow,
Vol. 94,
Issue. ,
p.
108937.
Diwakar, Anant
and
Bodi, Kowsik
2022.
Numerical and Empirical Studies of Heat Transfer in High-Enthalpy Argon–Air Mixtures.
Journal of Thermophysics and Heat Transfer,
Vol. 36,
Issue. 3,
p.
694.
Zhang, Bo
Yi, Shihe
Zhao, Yuxin
Yang, Rui
He, Lin
and
Lu, Xiaoge
2023.
Hypervelocity imperfect gas nozzle design with shared wave-elimination contour.
Physics of Fluids,
Vol. 35,
Issue. 8,
Xue, Longsheng
Jiao, Yun
Wang, Chengpeng
and
Cheng, Keming
2023.
Pressure plateau of separation induced by shock impingement in a Mach 5 flow.
Journal of Fluid Mechanics,
Vol. 972,
Issue. ,
Chang, Eric Won Keun
Joglekar, Chinmay
McGilvray, Matthew
and
Hermann, Tobias
2023.
Integration of Arc-jet in Impulse Facility for Hypervelocity Aerothermal Testing with Ablation.
Dai, Chunliang
Sun, Bo
Zhao, Dan
Zhou, Shengbing
Zhou, Changsheng
and
Man, Yanjin
2023.
High temperature non-equilibrium flow characteristics of impinging shock/flat-plate turbulent boundary layer interaction at Mach 8.42.
Physics of Fluids,
Vol. 35,
Issue. 9,
Capra, Bianca R.
and
Attwood, Lily G.
2023.
Energy Deposition on Analogous Vehicle Panel: Thermal–Structural Response.
Journal of Spacecraft and Rockets,
Vol. 60,
Issue. 6,
p.
1916.
Zhou, Yong-yi
Zhao, Yi-long
Zhao, Yu-xin
Zhong, Jia-cheng
and
He, Gang
2024.
Effects of the wall temperature on separation flowfield of swept shock wave/boundary layer interactions.
Physics of Fluids,
Vol. 36,
Issue. 6,
Yu, J. N.
Fan, D. W.
and
Zhou, Y.
2024.
Fluid-Structure-Sound Interactions and Control.
p.
247.
Hopkins, Keill J.
Ananthapadmanaban, Ramprakash
McIntyre, Timothy J.
Mee, David J.
Wheatley, Vincent
and
Veeraragavan, Ananthanarayanan
2024.
Experimental Verification of a Modified Cylindrical Focused Laser Differential Interferometer.
Journal of Spacecraft and Rockets,
Vol. 61,
Issue. 5,
p.
1194.
Dai, Chunliang
Sun, Bo
Zhao, Dan
Zhou, Shengbing
Zhou, Changsheng
and
Man, Yanjin
2024.
Numerical investigations on flow and heat transfer characteristics of a high-enthalpy double-cone in thermal and chemical non-equilibrium for hypersonic propulsion.
International Communications in Heat and Mass Transfer,
Vol. 155,
Issue. ,
p.
107522.
Rathi, Harsha
and
Sinha, Krishnendu
2024.
Simulation of Hypersonic Shock-Boundary Layer Interaction Using Shock-Strength Dependent Turbulence Model.
AIAA Journal,
Vol. 62,
Issue. 9,
p.
3244.
Neely, Andrew J.
Dooner, Dylan
and
Giannelis, Nicholas F.
2025.
The Challenges for Multi-Physics Validation Experiments for Trajectory Simulations of High-Speed Vehicles.
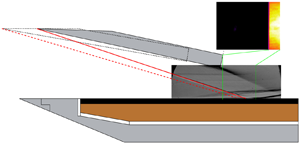
 $T_w=298\ \textrm {K}$ to
$T_w=298\ \textrm {K}$ to  $T_w\approx 675\ \textrm {K}$ during an experimental run. An oblique shock, generated by a plate that was inclined at
$T_w\approx 675\ \textrm {K}$ during an experimental run. An oblique shock, generated by a plate that was inclined at  $10^{\circ }$ or
$10^{\circ }$ or  $12^{\circ }$ to the free stream, was impinged on the heated flat plate to induce boundary layer separation. The primary flow condition produced Mach 7 flight-equivalent nozzle-supply enthalpy with a unit Reynolds number of
$12^{\circ }$ to the free stream, was impinged on the heated flat plate to induce boundary layer separation. The primary flow condition produced Mach 7 flight-equivalent nozzle-supply enthalpy with a unit Reynolds number of  $4.93\times 10^6\ \textrm {m}^{-1}$. More flow conditions with lower unit Reynolds numbers and flow enthalpies were considered to examine flow separation characteristics. Schlieren and infrared thermography captured the flow field and the wall temperature distribution, respectively. The results showed that the size of the flow separation grew with a higher
$4.93\times 10^6\ \textrm {m}^{-1}$. More flow conditions with lower unit Reynolds numbers and flow enthalpies were considered to examine flow separation characteristics. Schlieren and infrared thermography captured the flow field and the wall temperature distribution, respectively. The results showed that the size of the flow separation grew with a higher  $T_w$ and a lower unit Reynolds number. Moreover, the scaled separation of the present data showed a high discrepancy with existing separation correlations developed from a supersonic impinging shock and a hypersonic compression ramp, mainly due to the higher shock strength. Instead, the present data followed a scaling law that includes the pressure ratio across the impinging shock with a slight dependence on the wall temperature ratio.
$T_w$ and a lower unit Reynolds number. Moreover, the scaled separation of the present data showed a high discrepancy with existing separation correlations developed from a supersonic impinging shock and a hypersonic compression ramp, mainly due to the higher shock strength. Instead, the present data followed a scaling law that includes the pressure ratio across the impinging shock with a slight dependence on the wall temperature ratio.