Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-26T20:28:23.088Z Has data issue: false hasContentIssue false
  • English
  • Français

Hydrodynamic impulse enhancement of a vortex ring interacting with an axisymmetric co-axial aperture

Published online by Cambridge University Press:  28 April 2021

JiaCheng Hu  and
Sean D. Peterson Open the ORCID record for Sean D. Peterson [Opens in a new window]
JiaCheng Hu
Affiliation:
Department of Mechanical and Mechatronics Engineering, University of Waterloo, Waterloo, ONN2L 3G1, Canada
Sean D. Peterson*
Affiliation:
Department of Mechanical and Mechatronics Engineering, University of Waterloo, Waterloo, ONN2L 3G1, Canada
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

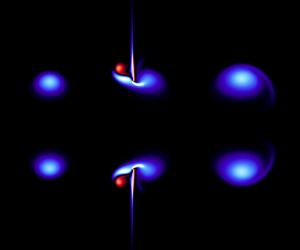
The dynamics of a vortex ring advecting towards and interacting with a solid wall with a coaxial aperture is governed by the aperture-to-ring radius ratio, $R_a/R_i$, which is parametrically explored herein through a series of numerical simulations for ring Reynolds numbers ranging from $Re=1000$ to $3000$. For $R_a/R_i \lessapprox 0.9$, the interaction largely resembles that of a vortex ring impacting a solid wall (impact regime), whereas, for $R_a/R_i \gtrapprox 1.3$, the ring passes through the aperture, with the influence of the interaction diminishing as $R_a/R_i$ increases (slip-through regime). When the aperture radius is approximately equal to that of the ring, however, an interesting phenomenon is observed, wherein the hydrodynamic impulse of the vortex ring is enhanced up to an additional 11 % at the highest considered Reynolds number when comparing with a free vortex ring that experiences no collision (herein termed the ‘vortex nozzle’ effect). Detailed investigation of the ‘vortex nozzle’ illustrates that the impulse enhancement is a consequence of two complementary effects: (i) fluid originating along the impact side of the wall is entrained into the ring, increasing its radius and volume; and (ii) the circulation loss during the interaction with the aperture tip is minimized due to the vortex core enveloping the aperture tip. In addition to ring impulse enhancement, the ‘vortex nozzle’ regime also exhibits the greatest volumetric flow rate through the nozzle and the highest loading on the structure, which may have practical engineering applications in smart material-based energy harvester designs.

JFM classification

Vortex Flows: Vortex dynamics Vortex Flows: Vortex interactions
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 917 , 25 June 2021 , A34
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Alben, S. 2012 The attraction between a flexible filament and a point vortex. J. Fluid Mech. 697, 481–503.CrossRefGoogle Scholar
Allen, J.J., Jouanne, Y. & Shashikanth, B.N. 2007 Vortex interaction with a moving sphere. J. Fluid Mech. 587, 337–346.CrossRefGoogle Scholar
Archer, P.J., Thomas, T.G. & Coleman, G.N. 2010 The instability of a vortex ring impinging on a free surface. J. Fluid Mech. 642, 79–94.CrossRefGoogle Scholar
Cantwell, B.J. 1986 Viscous starting jets. J. Fluid Mech. 173, 159–189.CrossRefGoogle Scholar
Cheng, M., Lou, J. & Luo, L.S. 2010 Numerical study of a vortex ring impacting a flat wall. J. Fluid Mech. 660, 430–455.Google Scholar
Chu, C.C., Wang, C.T. & Chang, C.C. 1995 a A vortex ring impinging on a solid plane surface – vortex structure and surface force. Phys. Fluids 7, 1391–1401.CrossRefGoogle Scholar
Chu, C.C., Wang, C.T., Chang, C.C., Chang, R.Y. & Chang, W.T. 1995 b Head-on collision of two coaxial vortex rings: experiment and computation. J. Fluid Mech. 296, 39–71.CrossRefGoogle Scholar
Dabiri, J.O. 2006 Note on the induced Lagrangian drift and added-mass of a vortex. J. Fluid Mech. 547, 105–113.CrossRefGoogle Scholar
Dabiri, J.O. & Gharib, M. 2004 Fluid entrainment by isolated vortex rings. J. Fluid Mech. 511, 311–331.CrossRefGoogle Scholar
Didden, N. 1979 On the formation of vortex rings: rolling-up and production of circulation. J. Appl. Maths Phys. 30, 101–116.Google Scholar
Fraenkel, L.E. 1972 Examples of steady vortex rings of small cross-section in an ideal fluid. J. Fluid Mech. 51, 119–135.CrossRefGoogle Scholar
Geuzaine, C. & Remacle, J.-F. 2009 Gmsh: A 3-D finite element mesh generator with built-in pre- and post-processing facilities. Intl J. Numer. Meth. Engng 79, 1309–1331.CrossRefGoogle Scholar
Gharib, M., Rambod, E. & Shariff, K. 1998 A universal time scale for vortex ring formation. J. Fluid Mech. 360, 121–140.Google Scholar
Heeg, R.S. & Riley, N. 1997 Simulations of the formation of an axisymmetric vortex ring. J. Fluid Mech. 339, 199–211.Google Scholar
Hu, J., Cha, Y., Porfiri, M. & Peterson, S.D. 2014 Energy harvesting from a vortex ring impinging on an annular ionic polymer metal composite. Smart Mater. Struct. 23, 074014.CrossRefGoogle Scholar
Hu, J. & Peterson, S.D. 2018 Vortex ring impingement on a wall with a coaxial aperture. Phys. Rev. Fluids 3, 084701.Google Scholar
Kim, D. & Gharib, M. 2011 Characteristics of vortex formation and thrust performance in drag-based paddling propulsion. J. Expl Biol. 214, 2283–2291.CrossRefGoogle ScholarPubMed
Masuda, N., Yoshida, J., Ito, B., Furuya, T. & Sano, O. 2012 Collision of a vortex ring on granular material. Part I. Interaction of the vortex ring with the granular layer. Fluid Dyn. Res. 44, 015501.CrossRefGoogle Scholar
Mohseni, K., Ran, H. & Colonius, T. 2001 Numerical experiments on vortex ring formation. J. Fluid Mech. 430, 267–282.CrossRefGoogle Scholar
Morton, B.R. 1984 The generation and decay of vorticity. Geophys. Astrophys. Fluid Dyn. 28, 277–308.CrossRefGoogle Scholar
Naaktgeboren, C., Krueger, P.S. & Lage, J.L. 2012 Interaction of a laminar vortex ring with a thin permeable screen. J. Fluid Mech. 707, 260–286.CrossRefGoogle Scholar
New, T.H., Long, J., Zang, B. & Shi, S. 2020 Collision of vortex rings upon V-walls. J. Fluid Mech. 899, A2.CrossRefGoogle Scholar
New, T.H. & Zang, B. 2017 Head-on collisions of vortex rings upon round cylinders. J. Fluid Mech. 833, 648–676.CrossRefGoogle Scholar
Nitsche, M. 2017 Deflection and trapping of a counter-rotating vortex pair by a flat plate. Phys. Rev. Fluids 2, 1–22.Google Scholar
Olsthoorn, J. & Dalziel, S.B. 2017 Three-dimensional visualization of the interaction of a vortex ring with a stratified interface. J. Fluid Mech. 820, 549–579.CrossRefGoogle Scholar
Olsthoorn, J. & Dalziel, S.B. 2018 Vortex-ring-induced stratified mixing: mixing model. J. Fluid Mech. 837, 129–146.CrossRefGoogle Scholar
Orlandi, P. 1990 Vortex dipole rebound from a wall. Phys. Fluids A 2, 1429–1436.Google Scholar
Orlandi, P. & Verzicco, R. 1993 Vortex rings impinging on walls: axisymmetric and three-dimensional simulations. J. Fluid Mech. 256, 615–646.CrossRefGoogle Scholar
Peterson, S.D. & Porfiri, M. 2012 a Energy exchange between a vortex ring and an ionic polymer metal composite. Appl. Phys. Lett. 100, 114102.CrossRefGoogle Scholar
Peterson, S.D. & Porfiri, M. 2012 b Interaction of a vortex pair with a flexible plate in an ideal quiescent fluid. J. Intell. Mater. Syst. Struct. 23, 1485–1504.CrossRefGoogle Scholar
Peterson, S.D. & Porfiri, M. 2013 Impact of a vortex dipole with a semi-infinite rigid plate. Phys. Fluids 25, 093103.CrossRefGoogle Scholar
Pirnia, A., Hu, J., Peterson, S.D. & Erath, B.D. 2017 Vortex dynamics and flow-induced vibrations arising from a vortex ring passing tangentially over a flexible plate. J. Appl. Phys. 122, 164901.CrossRefGoogle Scholar
Raffel, M., Willert, C.E., Wereley, S.T. & Kompenhans, J. 2007 Particle Image Velocimetry: A Practical Guide, 2nd edn. Springer.CrossRefGoogle Scholar
Saffman, P.G. 1993 Vortex Dynamics. Cambridge University Press.CrossRefGoogle Scholar
Sullivan, I.S., Niemela, J.J., Hershberger, R.E., Bolster, D. & Donnelly, R.J. 2008 Dynamics of thin vortex rings. J. Fluid Mech. 609, 319–347.CrossRefGoogle Scholar
Swearingen, J.D., Crouch, J.D. & Handler, R.A. 1995 Dynamics and stability of a vortex ring impacting a solid boundary. J. Fluid Mech. 297, 1–28.CrossRefGoogle Scholar
Tinaikar, A., Advaith, S. & Basu, S. 2018 Understanding evolution of vortex rings in viscous fluids. J. Fluid Mech. 836, 873–909.CrossRefGoogle Scholar
Verzicco, R. & Orlandi, P. 1994 Normal and oblique collisions of a vortex ring with a wall. Meccanica 29, 383–391.Google Scholar
Walker, J.D.A., Smith, C.R., Cerra, A.W. & Doligalski, T.L. 1987 The impact of a vortex ring on a wall. J. Fluid Mech. 181, 99–140.CrossRefGoogle Scholar
Wu, J.Z., Ma, H.Y. & Zhou, M.D. 2006 Vorticity and Vortex Dynamics. Springer.Google Scholar
Wu, J.Z., Ma, H.Y. & Zhou, M.D. 2015 Vortical Flows. Springer.CrossRefGoogle Scholar
Xu, Y., Wang, J.J., Feng, L.H., He, G.S. & Wang, Z.Y. 2018 Laminar vortex rings impinging onto porous walls with a constant porosity. J. Fluid Mech. 837, 729–764.CrossRefGoogle Scholar
Zivkov, E., Yarusevych, S., Porfiri, M. & Peterson, S.D. 2015 Numerical investigation of the interaction of a vortex dipole with a deformable plate. J. Fluids Struct. 58, 203–215.CrossRefGoogle Scholar

Hu and Peterson movie 1

Vorticity evolution for R_a/R_i=0.6 at Re=2000.

Download Hu and Peterson movie 1(Video)
Video 474.2 KB

Hu and Peterson movie 2

Vorticity evolution for R_a/R_i=1.0 at Re=2000.

Download Hu and Peterson movie 2(Video)
Video 297.7 KB

Hu and Peterson movie 3

Vorticity evolution for R_a/R_i=1.4 at Re=2000.

Download Hu and Peterson movie 3(Video)
Video 281.7 KB