Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-26T01:04:45.920Z Has data issue: false hasContentIssue false
  • English
  • Français

Heat or mass transport from drops in shearing flows. Part 2. Inertial effects on transport

Published online by Cambridge University Press:  06 July 2018

Deepak Krishnamurthy  and
Ganesh Subramanian Open the ORCID record for Ganesh Subramanian [Opens in a new window]
Deepak Krishnamurthy
Affiliation:
Bioengineering Department, Stanford University, Shriram Center, Room 064, 443 Via Ortega, Stanford, CA 94305-4125, USA
Ganesh Subramanian*
Affiliation:
Engineering Mechanics Unit, Jawaharlal Nehru Centre for Advanced Scientific Research, Bangalore, Karnataka 560064, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We analyse the singular effects of weak inertia on the heat (or equivalently mass) transport problem from drops in linear shearing flows. For small spherical drops embedded in hyperbolic planar linear flows, which constitute a one-parameter family (the parameter being $\unicode[STIX]{x1D6FC}$ with 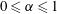$0\leqslant \unicode[STIX]{x1D6FC}\leqslant 1$, and whose extremal members are simple shear (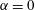$\unicode[STIX]{x1D6FC}=0$) and planar extension (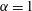$\unicode[STIX]{x1D6FC}=1$)), there are two distinct regimes for scalar (heat or mass) transport at large Péclet numbers (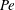$Pe$) depending on the exterior streamline topology (Krishnamurthy & Subramanian, J. Fluid Mech., vol. 850, 2018, pp. 439–483). When the drop-to-medium viscosity ratio (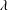$\unicode[STIX]{x1D706}$) is larger than a critical value, 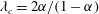$\unicode[STIX]{x1D706}_{c}=2\unicode[STIX]{x1D6FC}/(1-\unicode[STIX]{x1D6FC})$, the drop is surrounded by a region of closed streamlines in the inertialess limit (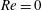$Re=0$, $Re$ being the drop Reynolds number). Convection is incapable of transporting heat away on account of the near-field closed streamline topology, and the transport remains diffusion limited even for 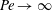$Pe\rightarrow \infty$. However, weak inertia breaks open the closed streamline region, giving way to finite-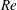$Re$ spiralling streamlines and convectively enhanced transport. For 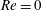$Re=0$ the closed streamlines on the drop surface, for $\unicode[STIX]{x1D706}>\unicode[STIX]{x1D706}_{c}$, are Jeffery orbits, a terminology originally used to describe the trajectories of an axisymmetric rigid particle in a simple shear flow. Based on this identification, a novel boundary layer analysis that employs a surface-flow-aligned non-orthogonal coordinate system, is used to solve the transport problem in the dual asymptotic limit 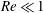$Re\ll 1$, $RePe\gg 1$, corresponding to the regime where inertial convection balances diffusion in an $O(RePe)^{-1/2}$ boundary layer. Further, the separation of time scales in the aforementioned limit, between rapid convection due to the Stokesian velocity field and the slower convection by the 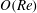$O(Re)$ inertial velocity field, allows one to average the convection–diffusion equation over the phase of the Stokesian surface streamlines (Jeffery orbits), allowing a simplification of the original three-dimensional non-axisymmetric transport problem to a form resembling a much simpler axisymmetric one. A self-similar ansatz then leads to the boundary layer temperature field, and the resulting Nusselt number is given by $Nu={\mathcal{H}}(\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D706})(RePe)^{1/2}$ with 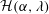${\mathcal{H}}(\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D706})$ given in terms of a one-dimensional integral; the prefactor 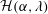${\mathcal{H}}(\unicode[STIX]{x1D6FC},\unicode[STIX]{x1D706})$ diverges for 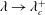$\unicode[STIX]{x1D706}\rightarrow \unicode[STIX]{x1D706}_{c}^{+}$ due to assumptions underlying the Jeffery-orbit-averaged analysis breaking down. Although the separation of time scales necessary for the validity of the analysis no longer exists in the transition regime ($\unicode[STIX]{x1D706}$ in the neighbourhood of 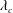$\unicode[STIX]{x1D706}_{c}$), scaling arguments nevertheless highlight the manner in which the Nusselt number function connects smoothly across the open and closed streamline regimes for any finite 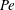$Pe$.

JFM classification

Drops and Bubbles: Drops and Bubbles Low-Reynolds-number flows: Low-Reynolds-number flows Multiphase and Particle-laden Flows: Multiphase and Particle-laden Flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 850 , 10 September 2018 , pp. 484 - 524
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Acrivos, A. 1971 Heat transfer at high Peclet number from a small sphere freely rotating in a simple shear field. J. Fluid Mech. 46 (02), 233–240.Google Scholar
Aris, R. 2012 Vectors, Tensors and the Basic Equations of Fluid Mechanics. Courier Dover Publications.Google Scholar
Barthes-Biesel, D. & Acrivos, A. 1973 Deformation and burst of a liquid droplet freely suspended in a linear shear field. J. Fluid Mech. 61, 1–21.Google Scholar
Bassom, A. R. & Gilbert, A. D. 1998 The spiral wind-up of vorticity in an inviscid planar vortex. J. Fluid Mech. 371, 109–140.Google Scholar
Bassom, A. R. & Gilbert, A. D. 1999 The spiral wind-up and dissipation of vorticity and passive scalar in a strained planar vortex. J. Fluid Mech. 398, 245–270.Google Scholar
Cox, R. G., Zia, I. Y. Z. & Mason, S. G. 1968 Particle motions in sheared suspensions xxv. streamlines around cylinders and spheres. J. Colloid Interface Sci. 27 (1), 7–18.Google Scholar
Dabade, V., Marath, N. K. & Subramanian, G. 2016 The effect of inertia on the orientation dynamics of anisotropic particles in simple shear flow. J. Fluid Mech. 791, 631–703.Google Scholar
Frankel, N. A. & Acrivos, A. 1968 Heat and mass transfer from small spheres and cylinders freely suspended in shear flow. Phys. Fluids 11, 1913–1918.Google Scholar
Greco, F. 2002 Second-order theory for the deformation of a newtonian drop in a stationary flow field. Phys. Fluids 14 (3), 946–954.Google Scholar
Jeffery, G. B. 1922 The motion of ellipsoidal particles immersed in a viscous fluid. Proc. R. Soc. Lond. A 102 (715), 161–179.Google Scholar
Kennedy, M. R., Pozrikidis, C. & Skalak, R. 1994 Motion and deformation of liquid drops, and the rheology of dilute emulsions in simple shear flow. Comput. Fluids 23 (2), 251–278.Google Scholar
Kossack, C. A. & Acrivos, A. 1974 Steady simple shear flow past a circular cylinder at moderate Reynolds numbers: a numerical solution. J. Fluid Mech. 66 (2), 353–376.Google Scholar
Krishnamurthy, D. & Subramanian, G. 2018 Heat or mass transport from drops in shearing flows. Part 1. The open streamline regime. J. Fluid Mech. 850, 439–483.Google Scholar
Leal, L. G. & Hinch, E. J. 1971 The effect of weak Brownian rotations on particles in shear flow. J. Fluid Mech. 46 (04), 685–703.Google Scholar
Leal, L. G. 2007 Advanced Transport Phenomena: Fluid Mechanics and Convective Transport Processes, vol. 7. Cambridge University Press.Google Scholar
Marath, N. K., Dwivedi, R. & Subramanian, G. 2017 An orientational order transition in a sheared suspension of anisotropic particles. J. Fluid Mech. 811, R3.Google Scholar
Marath, N. K. & Subramanian, G. 2018 The inertial orientation dynamics of anisotropic particles in planar linear flows. J. Fluid Mech. 844, 357–402.Google Scholar
Mikulencak, D. R. & Morris, J. F. 2004 Stationary shear flow around fixed and free bodies at finiite Reynolds number. J. Fluid Mech. 520, 215–242.Google Scholar
Pigeonneau, F., Perrodin, M. & Climent, E. 2014 Mass-transfer enhancement by a reversible chemical reaction across the interface of a bubble rising under stokes flow. AIChE J. 60 (9), 3376–3388.Google Scholar
Poe, G. G. & Acrivos, A. 1975 Closed-streamline flows past rotating single cylinders and spheres: inertia effects. J. Fluid Mech. 72 (04), 605–623.Google Scholar
Poe, G. G. & Acrivos, A. 1976 Closed streamline flows past small rotating particles: heat transfer at high Péclet numbers. Intl J. Multiphase Flow 2 (4), 365–377.Google Scholar
Powell, R. L. 1983 External and internal streamlines and deformation of drops in linear two-dimensional flows. J. Colloid Interface Sci. 95 (1), 148–162.Google Scholar
Raja, R. V., Subramanian, G. & Koch, D. L. 2010 Inertial effects on the rheology of a dilute emulsion. J. Fluid Mech. 646, 255–296.Google Scholar
Rhines, P. B. & Young, W. R. 1983 How rapidly is a passive scalar mixed within closed streamlines? J. Fluid Mech. 133, 133–145.Google Scholar
Robertson, C. R. & Acrivos, A. 1970 Low Reynolds number shear flow past a rotating circular cylinder. Part 1. Momentum transfer. J. Fluid Mech. 40 (04), 685–703.Google Scholar
Singh, R. K. & Sarkar, K. 2011 Inertial effects on the dynamics, streamline topology and interfacial stresses due to a drop in shear. J. Fluid Mech. 683, 149–171.Google Scholar
Subramanian, G. & Brady, J. F. 2006 Trajectory analysis for non-Brownian inertial suspensions in simple shear flow. J. Fluid Mech. 559, 151–2006.Google Scholar
Subramanian, G. & Koch, D. L. 2005 Inertial effects on fiber motion in simple shear flow. J. Fluid Mech. 535, 383–414.Google Scholar
Subramanian, G. & Koch, D. L. 2006a Centrifugal forces alter streamline topology and greatly enhance the rate of heat and mass transfer from neutrally buoyant particles to a shear flow. Phys. Rev. Lett. 96 (13), 134503.Google Scholar
Subramanian, G. & Koch, D. L. 2006b Inertial effects on the transfer of heat or mass from neutrally buoyant spheres in a steady linear velocity field. Phys. Fluids 18 (7), 073302.Google Scholar
Subramanian, G. & Koch, D. L. 2007 Heat transfer from a neutrally buoyant sphere in a second-order fluid. J. Non-Newtonian Fluid Mech. 144, 49–57.Google Scholar
Subramanian, G., Koch, D. L., Zhang, J. & Yang, C. 2011a The influence of the inertially dominated outer region on the rheology of a dilute dispersion of low-Reynolds-number drops or rigid particles. J. Fluid Mech. 674, 307–358.Google Scholar
Subramanian, G., Koch, D. L., Zhang, J. & Yang, C. 2011b The influence of the inertially dominated outer region on the rheology of a dilute dispersion of low-Reynolds-number drops or rigid particles. J. Fluid Mech. 674, 307–358.Google Scholar
Torza, S., Henry, C. P., Cox, R. G. & Mason, S. G. 1971 Particle motions in sheared suspensions. xxvi. Streamlines in and around liquid drops. J. Colloid Interface Sci. 35 (4), 529–543.Google Scholar
Vlahovska, P. M., Blawzdziewicz, J. & Loewenberg, M. 2009 Small-deformation theory for a surfactant-covered drop in linear flows. J. Fluid Mech. 624, 293–337.Google Scholar
Wolfram Research Inc. 2010 Mathematica 8.0.Google Scholar
Yang, C., Zhang, J., Koch, D. L. & Yin, X. 2011 Mass/heat transfer from a neutrally buoyant sphere in simple shear flow at finite Reynolds and Peclet numbers. AIChE J. 57 (6), 1419–1433.Google Scholar
Yu-Fang, P. & Acrivos, A. 1968 Heat transfer at high Peclet number in regions of closed streamlines. Intl J. Heat Mass Transfer 11 (3), 439–444.Google Scholar