Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-26T17:41:44.797Z Has data issue: false hasContentIssue false
  • English
  • Français

Global modes of viscous heated jets with real gas effects

Published online by Cambridge University Press:  07 February 2022

S. Demange Open the ORCID record for S. Demange [Opens in a new window] ,
U.A. Qadri Open the ORCID record for U.A. Qadri [Opens in a new window] ,
M.P. Juniper Open the ORCID record for M.P. Juniper [Opens in a new window]  and
F. Pinna
S. Demange*
Affiliation:
Department of Aeronautics and Aerospace, von Kármán Institute, Rhode-Saint-Genese 1640, Belgium
U.A. Qadri
Affiliation:
Department of Engineering, University of Cambridge, Cambridge CB2 1PZ, UK
M.P. Juniper
Affiliation:
Department of Engineering, University of Cambridge, Cambridge CB2 1PZ, UK
F. Pinna
Affiliation:
Department of Aeronautics and Aerospace, von Kármán Institute, Rhode-Saint-Genese 1640, Belgium
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This study investigates the stability features of spatially spreading heated jets in the viscous regime with real gas effects, using both unsteady two-dimensional axisymmetric simulations and linear analyses of the steady state and time-averaged states. At a Reynolds number of $400$, the heated jets are found to undergo a subcritical Hopf bifurcation, marking the start of self-sustained oscillations when decreasing the temperature ratio $S=T_{\infty }/T_{{c}}$, which highly depends on the thermodynamic and transport property assumptions imposed in the simulations. Once the flow enters a limit cycle past the Hopf bifurcation, the linear analyses over the steady base state are unable to capture the oscillation's frequency. 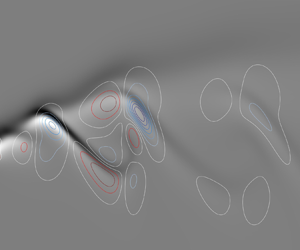Nevertheless, this study confirms that including real gas effects in the stability equations has a strong effect on the growth rate of the global mode once the centreline temperature of the jet reaches that of the dissociation reaction onset, which is $T=2800$ K for air at $p_s=100$ mbar. The linear global analyses over the time-averaged states lead to a satisfying prediction of the oscillation's frequency for the cases studied, and the baroclinic torque obtained from the resulting global mode matches well with that of the simulations.

JFM classification

Wakes/Jets: Jets Nonlinear Dynamical Systems: Bifurcation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 936 , 10 April 2022 , A7
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Åkervik, E., Brandt, L., Henningson, D.S., Hæpffner, J., Marxen, O. & Schlatter, P. 2006 Steady solutions of the Navier–Stokes equations by selective frequency damping. Phys. Fluids 18 (6), 068102.CrossRefGoogle Scholar
Anderson, J.D. 2006 Hypersonic and High-Temperature Gas Dynamics, 3rd edn. American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Balestra, G. 2013 Spatio-temporal linear stability analysis for heated coaxial jet flows. Master's thesis, ETH Zurich.Google Scholar
Balestra, G., Gloor, M. & Kleiser, L. 2015 Absolute and convective instabilities of heated coaxial jet flow. Phys. Fluids 27 (5), 054101.CrossRefGoogle Scholar
Bers, A. 1984 Space-time evolution of plasma instabilities-absolute and convective. In Basic Plasma Physics, p. 451. Cambridge University Press.Google Scholar
Bharadwaj, K.K. & Das, D. 2017 Global instability analysis and experiments on buoyant plumes. J. Fluid Mech. 832, 97–145.CrossRefGoogle Scholar
Bharadwaj, K.K. & Das, D. 2019 Puffing in planar buoyant plumes: BiGlobal instability analysis and experiments. J. Fluid Mech. 863, 817–849.CrossRefGoogle Scholar
Briggs, R.J. 1964 Electron-Stream Interaction with Plasmas. MIT Press.CrossRefGoogle Scholar
Chakravarthy, R.V.K., Lesshafft, L. & Huerre, P. 2018 Global stability of buoyant jets and plumes. J. Fluid Mech. 835, 654–673.CrossRefGoogle Scholar
Chandler, G.J., Juniper, M.P., Nichols, J.W. & Schmid, P.J. 2012 Adjoint algorithms for the Navier–Stokes equations in the low Mach number limit. J. Comput. Phys. 231 (4), 1900–1916.CrossRefGoogle Scholar
Chomaz, J. 2005 Global instabilities in spatially developing flows: non-normality and nonlinearity. Annu. Rev. Fluid Mech. 37, 357–392.CrossRefGoogle Scholar
Chomaz, J.M., Huerre, P. & Redekopp, L.G. 1988 Bifurcations to local and global modes in spatially developing flows. Phys. Rev. Lett. 60 (1), 25–28.CrossRefGoogle ScholarPubMed
Cipullo, A. 2010 Plasma flow characterisation by means of optical emission diagnostics. Project Rep. 2010-05. von Kármán Institute, Rhode Saint Genèse, Belgium.Google Scholar
Cipullo, A., Helber, B., Panerai, F., Zeni, F. & Chazot, O. 2014 Investigation of freestream plasma flow produced by inductively coupled plasma wind tunnel. J. Thermophys. Heat Transfer 28 (3), 381–393.CrossRefGoogle Scholar
Coenen, W., Lesshafft, L., Garnaud, X. & Secilla, A. 2017 Global instability of low-density jets. J. Fluid Mech. 820, 187–2017.CrossRefGoogle Scholar
Coenen, W. & Sevilla, A. 2012 The structure of the absolutely unstable regions in the near field of low-density jets. J. Fluid Mech. 713, 123–149.CrossRefGoogle Scholar
Coenen, W., Sevilla, A. & Sanchez, A.L. 2008 Absolute instability of light jets emerging from circular injector tubes. Phys. Fluids 20, 074104.CrossRefGoogle Scholar
Couairon, A. & Chomaz, J.-M. 1999 Fully nonlinear global modes in slowly varying flows. Phys. Fluids 11 (12), 3688–3703.CrossRefGoogle Scholar
Demange, S., Chazot, O. & Pinna, F. 2020 Local analysis of absolute instability in plasma jets. J. Fluid Mech. 903, 124104.CrossRefGoogle Scholar
Demange, S. & Pinna, F. 2020 On the role of thermo-transport properties in the convective/absolute transition of heated round jets. Phys. Fluids 32 (12), 124104.CrossRefGoogle Scholar
Demange, S., Qadri, U.A. & Pinna, F. 2019 Linear impulse response of a plasma jet. In Proceedings of the IUTAM Symposium on Laminar Turbulent Transition (ed. S. Sherwin, P. Schmid & X. Wu). Springer.Google Scholar
Hallberg, M.P. & Strykowski, P.J. 2006 On the universality of global modes in low-density axisymmetric jets. J. Fluid Mech. 569, 493–507.CrossRefGoogle Scholar
Hermanns, M. & Hernández, J.A. 2008 Stable high-order finite-difference methods based on non-uniform grid point distributions. Intl J. Numer. Meth. Fluids 56 (3), 233–255.CrossRefGoogle Scholar
Huerre, P. 2000 Open shear flow instabilities. In Perspectives in Fluid Dynamics (ed. G.K. Batchelor, H.K. Moffatt & M.G. Worster), pp. 159–229. Cambridge University Press.Google Scholar
Huerre, P. & Monkewitz, P.A. 1985 Absolute and convective instabilities in free shear layers. J. Fluid Mech. 159, 151–168.CrossRefGoogle Scholar
Huerre, P. & Monkewitz, P.A. 1990 Local and global instabilities in spatially developing flows. Annu. Rev. Fluid Mech. 22 (1), 473–537.CrossRefGoogle Scholar
Jendoubi, S. & Strykowski, P.J. 1994 Absolute and convective instability of axisymmetric jets with external flow. Phys. Fluids 6 (9), 3000–3009.CrossRefGoogle Scholar
Juniper, M.J. 2006 The effect of confinement on the stability of two-dimensional shear flows. J. Fluid Mech. 565, 171–195.CrossRefGoogle Scholar
Juniper, M.J. 2008 The effect of confinement on the stability of non-swirling round jet/wake flows. J. Fluid Mech. 605, 227–252.CrossRefGoogle Scholar
Kolb, J.F., Mohamed, A.-A.H., Price, R.O., Swanson, R.J., Bowman, A., Chiavarini, R.L., Stacey, M. & Schoenbach, K.H. 2008 Cold atmospheric pressure air plasma jet for medical applications. Appl. Phys. Lett. 92 (24), 241501.CrossRefGoogle Scholar
Kyle, D.M. & Sreenivasan, K.R. 1993 The instability and breakdown of a round variable-density jet. J. Fluid Mech. 249 (1), 619–664.CrossRefGoogle Scholar
Leontini, J.S., Thompson, M.C. & Hourigan, K. 2010 A numerical study of global frequency selection in the time-mean wake of a circular cylinder. J. Fluid Mech. 645, 435–446.CrossRefGoogle Scholar
Lesshafft, L. 2018 Artificial eigenmodes in truncated flow domains. Theor. Comput. Fluid Dyn. 32 (3), 245–262.CrossRefGoogle Scholar
Lesshafft, L., Coenen, W., Garnaud, X. & Sevilla, A. 2015 Modal instability analysis of light jets. Proc. IUTAM 14, 137–140.CrossRefGoogle Scholar
Lesshafft, L. & Huerre, P. 2007 Linear impulse response in hot round jets. Phys. Fluids 19 (2), 024102.CrossRefGoogle Scholar
Lesshafft, L., Huerre, P. & Sagaut, P. 2007 Frequency selection in globally unstable round jets. Phys. Fluids 19, 054108.CrossRefGoogle Scholar
Lesshafft, L., Huerre, P., Sagaut, P. & Terracol, M. 2005 Global modes in hot jets, absolute/convective instabilities and acoustic feedback. In 11th AIAA/CEAS Aeroacoustics Conference. American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Lesshafft, L. & Marquet, O. 2010 Optimal velocity and density profiles for the onset of absolute instability in jets. J. Fluid Mech. 662, 398–408.CrossRefGoogle Scholar
Malik, M.R. & Anderson, E.C. 1991 Real gas effects on hypersonic boundary-layer stability. Phys. Fluids 3, 803–821.CrossRefGoogle Scholar
Marieua, V., Reynierb, P., Marraffab, L., Vennemannb, D., Filippisc, F.D. & Caristiac, S. 2007 Evaluation of scirocco plasma wind-tunnel capabilities for entry simulations in co2 atmospheres. Acta Astronaut. 61, 604–616.Google Scholar
Michalke, A. 1984 Survey on jet instability theory. Prog. Aerosp. Sci. 21, 159–199.CrossRefGoogle Scholar
Miró Miró, F. 2020 Numerical investigation of hypersonic boundary-layer stability and transition in the presence of ablation phenomena. PhD Thesis, Universite Libre de Bruxelles/von Kármán Institute for Fluid Dynamics, Brussels, Belgium.Google Scholar
Miró Miró, F., Beyak, E.S., Pinna, F. & Reed, H.L. 2019 High-enthalpy models for boundary-layer stability and transition. Phys. Fluids 31 (4), 044101.CrossRefGoogle Scholar
Monkewitz, P., Bechert, D., Barsikow, B. & Lehmann, B. 1990 Self-excited oscillations and mixing in a heated round jet. J. Fluid Mech. 213, 611–639.CrossRefGoogle Scholar
Monkewitz, P. & Sohn, K. 1998 Absolute instability in hot jets. AIAA J. 26, 911–916.Google Scholar
Nichols, J. 2005 Simulation and stability analysis of jet diffusion flames. PhD thesis, University of Washington.Google Scholar
Nichols, J.W., Schmid, P. & Riley, J.J. 2007 Self-sustained oscillations in variable-density round jets. J. Fluid Mech. 582, 341–376.CrossRefGoogle Scholar
Oberleithner, K., Rukes, L. & Soria, J. 2014 Mean flow stability analysis of oscillating jet experiments. J. Fluid Mech. 757, 1–32.CrossRefGoogle Scholar
Park, C. 1993 Review of chemical-kinetic problems of future NASA missions. I - Earth entries. J. Thermophys. Heat Transfer 7 (3), 385–398.CrossRefGoogle Scholar
Pinna, F. 2012 Numerical study of stability for flows from low to high mach number. PhD thesis, Universita ‘La Sapienza’ di Roma/von Kármán Institute.Google Scholar
Pinna, F. 2013 VESTA toolkit: a software to compute transition and stability of boundary layers. In 43rd Fluid Dynamics Conference. American Institute of Aeronautics and Astronautics.CrossRefGoogle Scholar
Qadri, U.A., Chandler, G.J. & Juniper, M.P. 2015 Self-sustained hydrodynamic oscillations in lifted jet diffusion flames: origin and control. J. Fluid Mech. 775, 201–222.CrossRefGoogle Scholar
Qadri, U.A., Chandler, G.J. & Juniper, M.P. 2018 Passive control of global instability in low-density jets. Eur. J. Mech. B/Fluids 72, 311–319.CrossRefGoogle Scholar
Sevilla, A., Gordillo, J.M. & Martínnez-Bazan, C. 2002 The effect of the diameter ratio on the absolute and convective instability of free coflowing jets. Phys. Fluids 14 (9), 3028–3038.CrossRefGoogle Scholar
Sipp, D. & Lebedev, A. 2007 Global stability of base and mean flows: a general approach and its applications to cylinder and open cavity flows. J. Fluid Mech. 593, 333–358.CrossRefGoogle Scholar
Spores, R. & Pfender, E. 1989 Flow structure of a turbulent thermal plasma jet. Surf. Coat. Technol. 37 (3), 251–270.CrossRefGoogle Scholar
Sreenivasan, K.R., Raghu, S. & Kyle, D. 1989 Absolute instability in variable density round jets. Exp. Fluids 7 (5), 309–317.CrossRefGoogle Scholar
Sutherland, W. 1893 The viscosity of gases and molecular force. Lond. Edinb. Dub. Phil. Mag. J. Sci. 36 (223), 507–531.CrossRefGoogle Scholar
Tammisola, O. & Juniper, M.P. 2016 Coherent structures in a swirl injector at $Re = 4800$ by nonlinear simulations and linear global modes. J. Fluid Mech. 792, 620–657.CrossRefGoogle Scholar
Theofilis, V. 2003 Advances in global linear instability analysis of nonparallel and three-dimensional flows. Prog. Aerosp. Sci. 39 (4), 249–315.CrossRefGoogle Scholar
Turton, S.E., Tuckerman, L.S. & Barkley, D. 2015 Prediction of frequencies in thermosolutal convection from mean flows. Phys. Rev. E 91 (4), 043009.CrossRefGoogle ScholarPubMed
Zhu, Y., Gupta, V. & Li, L.K.B. 2017 Onset of global instability in low-density jets. J. Fluid Mech. 828, R1.CrossRefGoogle Scholar

Demange et al. supplementary movie 1

See pdf file for movie caption
Download Demange et al. supplementary movie 1(Video)
Video 1.9 MB

Demange et al. supplementary movie 2

See pdf file for movie caption

Download Demange et al. supplementary movie 2(Video)
Video 9.4 MB

Demange et al. supplementary movie 3

See pdf file for movie caption

Download Demange et al. supplementary movie 3(Video)
Video 10 MB

Demange et al. supplementary movie 4

See pdf file for movie caption

Download Demange et al. supplementary movie 4(Video)
Video 11.4 MB

Demange et al. supplementary movie 5

See pdf file for movie caption

Download Demange et al. supplementary movie 5(Video)
Video 14.7 MB

Demange et al. supplementary movie 6

See pdf file for movie caption

Download Demange et al. supplementary movie 6(Video)
Video 12.4 MB

Demange et al. supplementary movie 7

See pdf file for movie caption

Download Demange et al. supplementary movie 7(Video)
Video 15.4 MB
Supplementary material: PDF

Demange et al. supplementary material

Captions for movies 1-7

Download Demange et al. supplementary material(PDF)
PDF 13.4 KB
Supplementary material: PDF

Demange et al. supplementary material

Supplementary data

Download Demange et al. supplementary material(PDF)
PDF 138.9 KB