Hostname: page-component-78c5997874-fbnjt Total loading time: 0 Render date: 2024-11-19T08:24:36.623Z Has data issue: false hasContentIssue false
  • English
  • Français

Experiments on gravity currents propagating on different bottom slopes

Published online by Cambridge University Press:  14 August 2013

Albert Dai
Albert Dai*
Affiliation:
Department of Water Resources and Environmental Engineering, Tamkang University, Taiwan 251-37, Taiwan
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Experiments for gravity currents generated from an instantaneous buoyancy source propagating on an inclined boundary in the slope angle range ${0}^{\circ } \leq \theta \leq {9}^{\circ } $ are reported. While the flow patterns for gravity currents on 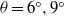$\theta = {6}^{\circ } , {9}^{\circ } $ are qualitatively different from those on 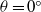$\theta = {0}^{\circ } $, similarities are observed in the acceleration phase for the flow patterns between 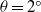$\theta = {2}^{\circ } $ and 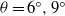$\theta = {6}^{\circ } , {9}^{\circ } $ and in the deceleration phase, the patterns for gravity currents on 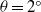$\theta = {2}^{\circ } $ are found similar to those on $\theta = {0}^{\circ } $. Previously, it was known that the front location history in the deceleration phase obeys a power-relationship, which is essentially an asymptotic form of the solution to thermal theory. We showed that this power-relationship applies only in the early stage of the deceleration phase, and when gravity currents propagate into the later stage of the deceleration phase, viscous effects become more important and the front location data deviate from this relationship. When the power-relationship applies, it is found that at 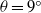$\theta = {9}^{\circ } $, 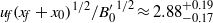${u}_{f} {({x}_{f} + {x}_{0} )}^{1/ 2} / {{ B}_{0}^{\prime } }^{1/ 2} \approx 2. 8{ 8}_{- 0. 17}^{+ 0. 19} $, which changes to $2. 8{ 6}_{- 0. 13}^{+ 0. 13} $ at 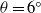$\theta = {6}^{\circ } $, $2. 5{ 4}_{- 0. 07}^{+ 0. 08} $ at 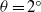$\theta = {2}^{\circ } $, and $1. 5{ 1}_{- 0. 07}^{+ 0. 07} $ on a horizontal boundary, where ${u}_{f} $ is the front velocity, $({x}_{f} + {x}_{0} )$ is the front location measured from the virtual origin, and ${ B}_{0}^{\prime } $ is the released buoyancy. Our results indicate that in the slope angle range 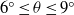${6}^{\circ } \leq \theta \leq {9}^{\circ } $, the asymptotic relationship between the front velocity and front location in the deceleration phase is not sensitive to the variation of slope angle. In the late deceleration phase when the front location data deviate from the power-relationship, we found that the flow patterns for 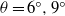$\theta = {6}^{\circ } , {9}^{\circ } $ are dramatically different from those for $\theta = {0}^{\circ } , {2}^{\circ } $. For high slope angles, i.e. $\theta = {6}^{\circ } , {9}^{\circ } $, the edge of the gravity current head experiences a large upheaval and enrolment by ambient fluid towards the end of the deceleration phase, while for low slope angles, i.e. $\theta = {0}^{\circ } , {2}^{\circ } $, the gravity current head maintains a more streamlined shape without violent mixing with ambient fluid throughout the course of gravity current propagation. Our findings indicate two plausible routes to the finale of a gravity current event.

JFM classification

Geophysical and Geological Flows: Geophysical and Geological Flows Geophysical and Geological Flows: Gravity currents
Type
Papers
Information
Journal of Fluid Mechanics , Volume 731 , 25 September 2013 , pp. 117 - 141
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adduce, C., Sciortino, G. & Proietti, S. 2012 Gravity currents produced by lock-exchanges: experiments and simulations with a two layer shallow-water model with entrainment. J. Hydraul. Engng 138 (2), 111–121.Google Scholar
Baines, P. G. 2001 Mixing in flows down gentle slopes into stratified environments. J. Fluid Mech. 443, 237–270.Google Scholar
Baines, P. G. 2005 Mixing regimes for the flow of dense fluid down slopes into stratified environments. J. Fluid Mech. 538, 245–267.Google Scholar
Batchelor, G. K. 1967 An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
Beghin, P., Hopfinger, E. J. & Britter, R. E. 1981 Gravitational convection from instantaneous sources on inclined boundaries. J. Fluid Mech. 107, 407–422.Google Scholar
Birman, V. K., Battandier, B. A., Meiburg, E. & Linden, P. F. 2007 Lock-exchange flows in sloping channels. J. Fluid Mech. 577, 53–77.Google Scholar
Britter, R. E. & Linden, P. F. 1980 The motion of the front of a gravity current travelling down an incline. J. Fluid Mech. 99, 531–543.Google Scholar
Cantero, M., Lee, J., Balachandar, S. & Garcia, M. 2007 On the front velocity of gravity currents. J. Fluid Mech. 586, 1–39.Google Scholar
Dade, W. B., Lister, J. R. & Huppert, H. E. 1994 Fine-sediment deposition from gravity surges on uniform slopes. J. Sedim. Res. 64, 423–432.Google Scholar
Dalziel, S. B. 2012 DigiFlow User Guide. Dalziel Research Partners.Google Scholar
Ellison, T. H. & Turner, J. S. 1959 Turbulent entrainment in stratified flows. J. Fluid Mech. 6, 423–448.Google Scholar
Fannelop, T. K. 1994 Fluid Mechanics for Industrial Safety and Environmental Protection. Elsevier.Google Scholar
Hopfinger, E. J. 1983 Snow avalanche motion and related phenomena. Annu. Rev. Fluid Mech. 15, 47–76.Google Scholar
Hoult, D. P. 1972 Oil spreading on the sea. Annu. Rev. Fluid Mech. 4, 341–368.Google Scholar
Huppert, H. 1982 The propagation of two-dimensional and axisymmetric viscous gravity currents over a rigid horizontal boundary surface. J. Fluid Mech. 121, 43–58.Google Scholar
Huppert, H. & Simpson, J. 1980 The slumping of gravity currents. J. Fluid Mech. 99, 785–799.Google Scholar
La Rocca, M., Adduce, C., Lombardi, V., Sciortino, G. & Hinkermann, R. 2012a Development of a lattice Boltzmann method for two-layered shallow-water flow. Intl J. Numer. Meth. Fluids 70 (8), 1048–1072.Google Scholar
La Rocca, M., Adduce, C., Sciortino, G., Bateman, P. A. & Boniforti, M. A. 2012b A two-layer shallow water model for 3d gravity currents. J. Hydraul Res. 50 (2), 208–217.Google Scholar
La Rocca, M., Adduce, C., Sciortino, G. & Pinzon, A. B. 2008 Experimental and numerical simulation of three-dimensional gravity currents on smooth and rough bottom. Phys. Fluids 20 (10), 106603.Google Scholar
Marino, B., Thomas, L. & Linden, P. 2005 The front condition for gravity currents. J. Fluid Mech. 536, 49–78.Google Scholar
Maxworthy, T. 2010 Experiments on gravity currents propagating down slopes. Part 2. The evolution of a fixed volume of fluid released from closed locks into a long, open channel. J. Fluid Mech. 647, 27–51.Google Scholar
Maxworthy, T. & Nokes, R. I. 2007 Experiments on gravity currents propagating down slopes. Part 1. The release of a fixed volume of heavy fluid from an enclosed lock into an open channel. J. Fluid Mech. 584, 433–453.Google Scholar
Monaghan, J. J., Cas, R. A. F., Kos, A. M. & Hallworth, M. 1999 Gravity currents descending a ramp in a stratified tank. J. Fluid Mech. 379, 39–69.Google Scholar
Nogueira, H. I. S., Adduce, C., Alves, E. & Franca, M. J. 2013 Image analysis technique applied to lock-exchange gravity currents. Meas. Sci. Technol. 24, 047001.Google Scholar
Rastello, M. & Hopfinger, E. J. 2004 Sediment-entraining suspension clouds: a model of powder-snow avalanches. J. Fluid Mech. 509, 181–206.Google Scholar
Ross, A. N., Linden, P. F. & Dalziel, S. B. 2002 A study of three-dimensional gravity currents on a uniform slope. J. Fluid Mech. 453, 239–261.Google Scholar
Seon, T., Znaien, J., Perrin, B., Hinch, E. J., Salin, D. & Hulin, J. P. 2007 Front dynamics and macroscopic diffusion in buoyant mixing in tilted tubes. Phys. Fluids 19, 125105.Google Scholar
Shin, J., Dalziel, S. & Linden, P. 2004 Gravity currents produced by lock exchange. J. Fluid Mech. 521, 1–34.Google Scholar
Simpson, J. 1997 Gravity Currents, 2nd edn. Cambridge University Press.Google Scholar
Tickle, G. 1996 A model of the motion and dilution of a heavy gas cloud released on a uniform slope in calm conditions. J. Hazard. Mater. 49, 29–47.Google Scholar
Webber, D., Jones, S. & Martin, D. 1993 A model of the motion of a heavy gas cloud released on a uniform slope. J. Hazard. Mater. 33, 101–122.Google Scholar