Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-09T15:01:20.150Z Has data issue: false hasContentIssue false
  • English
  • Français

Expansion wave diffraction over a 90 degree corner

Published online by Cambridge University Press:  25 September 2014

Irshaad Mahomed  and
Beric W. Skews
Irshaad Mahomed
Affiliation:
Flow Research Unit, School of Mechanical, Industrial and Aeronautical Engineering, University of the Witwatersrand, Johannesburg, 2050, South Africa
Beric W. Skews*
Affiliation:
Flow Research Unit, School of Mechanical, Industrial and Aeronautical Engineering, University of the Witwatersrand, Johannesburg, 2050, South Africa
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The diffraction of an initially one-dimensional plane expansion wave over a 90° corner was explored using experiment and numerical simulation. Unlike studies of shock diffraction, expansion wave diffraction has hardly been documented previously. The planar expansion wave was produced in a shock tube by bursting a diaphragm. Two independent parameters were identified for study: (i) the initial diaphragm shock tube pressure ratio, which determines the strength (pressure ratio) of the expansion, and (ii) the position of the diaphragm from the apex of the 90° corner, which determines the width of the wave. The experimentation only considered variation in the shock tube pressure ratio whereas the simulation varied both parameters. A Navier–Stokes solver with Menter’s shear stress transport 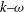$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}k\mbox{--}\omega $ turbulence model was found to adequately model the overall flow field. A number of major flow features were identified occurring in the vicinity of the corner. These were: a shear layer that originated by flow separation at the apex of the corner; a vortex within a separation bubble that remained attached to the wall, in sharp contrast to what happens in the shock wave diffraction case, where the vortex convects downstream; and a reflected compression wave arising from perturbation signals generated by the diffraction. For a narrow-width expansion wave existing prior to diffraction, the reflected compression wave steepens into an outwardly propagating, weak cylindrical shock wave. Regions of supersonic flow are identified surrounding the bubble and can extend downstream depending on the pressure ratio. Another major flow feature identified in some cases was an oblique shock located near the separation bubble. A large wake region is evident immediately downstream of the bubble and appears to consist of two distinct layers. The experimental results showed large-scale turbulent structures within the separation bubble, and shear layer instability and vortex shedding from the separation bubble were also evident.

JFM classification

Compressible Flows: Compressible Flows Compressible Flows: Gas dynamics Compressible Flows: Shock waves
Type
Papers
Information
Journal of Fluid Mechanics , Volume 757 , 25 October 2014 , pp. 649 - 664
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anderson, W. M. 1967 Diffraction of an incomplete expansion wave by a corner. Proc. Camb. Phil. Soc. 63, 909–921.CrossRefGoogle Scholar
Anderson, J. 2003 Modern Compressible Flow with Historical Perspective. McGraw-Hill.Google Scholar
Billington, I. J. 1956 An experimental study of one-dimensional refraction of a rarefaction wave at a contact surface. J. Aeronaut. Sci. 23, 997–1006.Google Scholar
Glass, I. I. & Sislean, J. P. 1994 Nonstationary Flows and Shock Waves. Oxford University Press.CrossRefGoogle Scholar
Kleine, H., Ritzerfeld, E. & Grönig, H. 2003 Shock wave diffraction at a ninety degree corner. Comput. Fluid Dyn. J. 12, 142–158.Google Scholar
Powell, J. B. L. 1957 The diffraction of a rarefaction wave by a corner. J. Fluid Mech. 3, 243–254.CrossRefGoogle Scholar
Skews, B. W. 1967 The perturbed region behind a diffracting shock wave. J. Fluid Mech. 29, 705–719.Google Scholar
Skews, B., Law, C., Muritala, A. & Bode, S. 2012 Shear layer behaviour resulting from shock wave diffraction. Exp. Fluids 52, 417–424.CrossRefGoogle Scholar