Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-23T11:48:32.193Z Has data issue: false hasContentIssue false
  • English
  • Français

Erosion–deposition waves in shallow granular free-surface flows

Published online by Cambridge University Press:  02 December 2014

A. N. Edwards  and
J. M. N. T. Gray
A. N. Edwards
Affiliation:
School of Mathematics and Manchester Centre for Nonlinear Dynamics, University of Manchester, Manchester M13 9PL, UK
J. M. N. T. Gray*
Affiliation:
School of Mathematics and Manchester Centre for Nonlinear Dynamics, University of Manchester, Manchester M13 9PL, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Debris flows can spontaneously develop regular large-amplitude surge waves that are interspersed by periods in which the channel fill is completely stationary. These are important because each individual surge is much more destructive than a steady uniform flow with the same mass flux. In this paper small-scale experiments that exhibit similar behaviour are described. The flow consists of carborundum particles that flow down a rough inclined chute covered with a static erodible layer of the same grains. For inflow conditions close to the minimum depth required for steady uniform flows to exist, small disturbances are unstable, creating waves that rapidly coarsen and grow in size. As the waves become sufficiently large, the troughs between the wave crests drop below a critical thickness and come to rest. A series of steadily travelling waves develop which erode the static layer of particles in front of them and deposit grains behind them, to form a layer that is again stationary. This is, in turn, re-eroded and deposited by the next wave. We term these waves granular erosion–deposition waves. Although erosion and deposition problems are notoriously difficult, a simple model is developed which uses a depth-averaged version of the 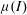${\it\mu}(I)$-rheology and Pouliquen and Forterre’s extended friction law. The viscous dissipation combines with dynamic, intermediate and static friction regimes to generate finite-length waves with static and mobile regions. The existence of stationary layers fundamentally distinguishes erosion–deposition waves from granular roll waves, which form in slightly deeper flows and are always completely mobilized. Numerical simulations show that the system of equations is able to model both erosion–deposition waves and granular roll waves. Moreover, the computed wave amplitude, wavespeed and coarsening dynamics are in good quantitative agreement with experiments.

JFM classification

Granular media: Granular media Non-Newtonian Flows: Rheology Waves/Free-surface Flows: Waves/Free-surface Flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 762 , 10 January 2015 , pp. 35 - 67
Check for updates
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aranson, I. S. & Tsimring, L. S. 2001 Continuum description of avalanches in granular media. Phys. Rev. E 64, 020301(R).Google Scholar
Aranson, I. S. & Tsimring, L. S. 2002 Continuum theory of partially fluidized granular flows. Phys. Rev. E 65, 061303.CrossRefGoogle ScholarPubMed
Balmforth, N. J. & Liu, J. 2004 Roll waves in mud. J. Fluid Mech. 519, 33–54.CrossRefGoogle Scholar
Balmforth, N. J. & Mandre, S. 2004 Dynamics of roll waves. J. Fluid Mech. 514, 1–33.Google Scholar
Barker, T., Schaeffer, D. G., Bohórquez, P. & Gray, J. M. N. T. 2014 Well-posed and ill-posed behaviour of the ${\it\mu}(I)$ -rheology for granular flows. J. Fluid Mech. (submitted).Google Scholar
Börzsönyi, T., Halsey, T. C. & Ecke, R. E. 2005 Two scenarios for avalanche dynamics in inclined granular layers. Phys. Rev. Lett. 94, 208001.Google Scholar
Börzsönyi, T., Halsey, T. C. & Ecke, R. E. 2008 Avalanche dynamics on a rough inclined plane. Phys. Rev. E 78, 011306.CrossRefGoogle ScholarPubMed
Bouchaud, J. P., Cates, M. E., Prakash, J. R. & Edwards, S. F. 1994 A model for the dynamics of sandpile surfaces. J. Phys. I France 4, 1383–1410.CrossRefGoogle Scholar
Clement, E., Malloggi, F., Andreotti, B. & Aranson, I. S. 2007 Erosive granular avalanches: a cross-confrontation between experiment and theory. Granul. Matt. 10, 3–11.CrossRefGoogle Scholar
Cornish, V. 1910 Ocean Waves and Kindred Geophysical Phenomena. Cambridge University Press.Google Scholar
Cui, X. & Gray, J. M. N. T. 2013 Gravity-driven granular free-surface flow around a circular cylinder. J. Fluid Mech. 720, 314–337.CrossRefGoogle Scholar
Daerr, A. 2001 Dynamical equilibrium of avalanches on a rough plane. Phys. Fluids 13, 2115–2124.CrossRefGoogle Scholar
Daerr, A. & Douady, S. 1999 Two types of avalanche behaviour in granular media. Nature 399, 241–243.CrossRefGoogle Scholar
Davies, T. R. H. 1986 Large debris flows: a macro-viscous phenomenon. Acta Mechanica 63, 161–178.CrossRefGoogle Scholar
Davies, T. R. H. 1990 Debris-flow surges – experimental simulation. J. Hydrol. (NZ) 29, 18–46.Google Scholar
Douady, S., Andreotti, B. & Daerr, A. 1999 On granular surface flow equations. Eur. Phys. J. B 11, 131–142.Google Scholar
Doyle, E. E., Huppert, H. E., Lube, G., Mader, H. M. & Sparks, R. S. J. 2007 Static and flowing regions in granular collapses down channels: insights from a sedimenting shallow water model. Phys. Fluids 19, 106601.Google Scholar
Dressler, R. F. 1949 Mathematical solution of the problem of roll-waves in inclined open channels. Commun. Pure Appl. Maths 2, 149–194.CrossRefGoogle Scholar
Forterre, Y. 2006 Kapiza waves as a test for three-dimensional granular flow rheology. J. Fluid Mech. 563, 123–132.Google Scholar
Forterre, Y. & Pouliquen, O. 2003 Long-surface-wave instability dense granular flows. J. Fluid Mech. 486, 21–50.Google Scholar
GDR-MiDi 2004 On dense granular flows. Eur. Phys. J. E 14, 341–365.CrossRefGoogle Scholar
Gray, J. M. N. T. 2001 Granular flow in partially filled slowly rotating drums. J. Fluid Mech. 441, 1–29.Google Scholar
Gray, J. M. N. T. & Ancey, C. 2009 Segregation, recirculation and deposition of coarse particles near two-dimensional avalanche fronts. J. Fluid Mech. 629, 387–423.CrossRefGoogle Scholar
Gray, J. M. N. T. & Edwards, A. N. 2014 A depth-averaged ${\it\mu}(I)$ -rheology for shallow granular free-surface flows. J. Fluid Mech. 755, 503–534.CrossRefGoogle Scholar
Gray, J. M. N. T., Tai, Y. C. & Noelle, S. 2003 Shock waves, dead-zones and particle-free regions in rapid granular free-surface flows. J. Fluid Mech. 491, 161–181.CrossRefGoogle Scholar
Gray, J. M. N. T., Wieland, M. & Hutter, K. 1999 Free surface flow of cohesionless granular avalanches over complex basal topography. Proc. R. Soc. A 455, 1841–1874.CrossRefGoogle Scholar
Grigorian, S. S., Eglit, M. E. & Iakimov, I. L. 1967 Novaya postanovka i reshenie zadachi o dvizhenii snezhnoi laviny (A new formulation and solution of the problem of snow avalanche motion). Tr. Vysokogornogo Geofizich Inst. 12, 104–113.Google Scholar
Iverson, R. M. 2012 Elementary theory of bed-sediment entrainment by debris flows and avalanches. J. Geophys. Res. 117, F03006.Google Scholar
Iverson, R. M., Logan, M., LaHusen, R. G. & Berti, M. 2010 The perfect debris flow? Aggregated results from 28 large-scale experiments J. Geophys. Res. 115, F03005.Google Scholar
Johnson, C. G. & Gray, J. M. N. T. 2011 Granular jets and hydraulic jumps on an inclined plane. J. Fluid Mech. 675, 87–116.Google Scholar
Jop, P., Forterre, Y. & Pouliquen, O. 2005 Crucial role of sidewalls in granular surface flows: consequences for the rheology. J. Fluid Mech. 541, 167–192.Google Scholar
Jop, P., Forterre, Y. & Pouliquen, O. 2006 A constitutive relation for dense granular flows. Nature 44, 727–730.CrossRefGoogle Scholar
Kranenburg, C. 1992 On the evolution of roll waves. J. Fluid Mech. 245, 249–261.Google Scholar
Kurganov, A. & Tadmor, E. 2000 New high-resolution central schemes for nonlinear conservationl laws and convection–diffusion equations. J. Comput. Phys. 160, 241–282.Google Scholar
Lagrée, P.-Y., Staron, L. & Popinet, S. 2011 The granular column collapse as a continuum: validity of a two-dimensional Navier–Stokes model with a ${\it\mu}(I)$ -rheology. J. Fluid Mech. 686, 378–408.CrossRefGoogle Scholar
Li, J., Jianmo, J. Y., Bi, C. & Luo, D. 1983 The main features of the mudflow in Jiang-Jia Ravine. Z. Geomorphol. 27, 325–341.Google Scholar
Marchi, L., Arattano, M. & Deganutti, A. M. 2002 Ten years of debris-flow monitoring in the Moscardo torrent, Italian Alps. Geomorphology 46, 1–17.Google Scholar
McArdell, B. W., Zanuttigh, B., Lamberti, A. & Rickenmann, D. 2003 Systematic comparison of debris flow laws at the Illgraben torrent, Switzerland. In Debris-Flow Hazards Mitigation: Mechanics, Prediction, and Assessment: Proceedings of the Third International Conference on Debris-Flow Hazards Mitigation, Davos, Switzerland, September 10–12, 2003 (ed. Rickenmann, D. & Chen, C.-L.), pp. 647–658. Millpress.Google Scholar
Medovikov, A. A. 1998 High order explicit methods for parabolic equations. BIT 38 (2), 372–390.Google Scholar
Needham, D. J. & Merkin, J. H. 1984 On roll waves down an open inclined channel. Proc. R. Soc. Lond. A 394, 259–278.Google Scholar
Nessyahu, H. & Tadmor, E. 1990 Non-oscillatory central differencing for hyperbolic conservation laws. J. Comput. Phys. 87, 408–463.Google Scholar
Pouliquen, O. 1999a Scaling laws in granular flows down rough inclined planes. Phys. Fluids 11 (3), 542–548.Google Scholar
Pouliquen, O. 1999b On the shape of granular fronts down rough inclined planes. Phys. Fluids 11 (7), 1956–1958.Google Scholar
Pouliquen, O. & Forterre, Y. 2002 Friction law for dense granular flows: application to the motion of a mass down a rough inclined plane. J. Fluid Mech. 453, 133–151.Google Scholar
Pouliquen, O. & Renaut, N. 1996 Onset of granular flows on an inclined rough surface: dilatancy effects. J. Phys. II France 6, 923–935.Google Scholar
Savage, S. B. & Hutter, K. 1989 The motion of a finite mass of granular material down a rough incline. J. Fluid Mech. 199, 177–215.Google Scholar
Staron, L., Lagrée, P.-Y. & Popinet, S. 2012 The granular silo as a continuum plastic flow: the hour-glass versus the clepsydra. Phys. Fluids 24, 103301.Google Scholar
Tai, Y. C. & Kuo, C. Y. 2008 A new model of granular flows over general topography with erosion and deposition. Acta Mech. 199, 71–96.CrossRefGoogle Scholar
Tai, Y. C. & Kuo, C. Y. 2012 Modelling shallow debris flows of the Coulomb-mixture type over temporally varying topography. Nat. Hazards Earth Syst. Sci. 12, 269–280.Google Scholar
Takagi, D., McElwaine, J. N. & Huppert, H. H. 2011 Shallow granular flows. Phys. Rev. E 88, 031306.Google Scholar
Vallance, J. W.1994 Experimental and field studies related to the behaviour of granular mass flows and the characteristics of their deposits. PhD thesis, Michigan Technological University.Google Scholar
Weiyan, T. 1992 Shallow Water Hydrodynamics. Elsevier.Google Scholar
Whitham, G. B. 1974 Linear and Nonlinear Waves. John Wiley.Google Scholar
Zanuttigh, B. & Lamberti, A. 2007 Instability and surge development in debris flows. Rev. Geophys. 45, RG3006.Google Scholar

Edwards and Gray supplementary movie

An oblique view of erosion-deposition waves propagating from top to bottom in a flow of carborundum particles, on a chute inclined at 35.1 degrees to the horizontal with a rough bed of spherical glass beads. The chute has glass sidewalls on its 7.8cm width and a length of 3.29m from the inflow gate to the outflow end, with a visible region here that is approximately 50cm in length and near to the midpoint of the downslope $x$-direction. Regions of stationary material between successive waves and the merging of waves propagating at different speeds can be observed.

Download Edwards and Gray supplementary movie(Video)
Video 6.2 MB

Edwards and Gray supplementary movie

An overhead view of erosion-deposition waves propagating from top to bottom in a flow of carborundum particles, on a chute inclined at 35.1 degrees to the horizontal with a rough bed of spherical glass beads. The chute has glass sidewalls on its 7.8cm width and a length of 3.29m from the inflow gate to the outflow end, with a visible region here that is approximately 0.2m in length and near to the midpoint of the downslope $x$-direction. Regions of stationary material between successive waves and the merging of waves propagating at different speeds can be observed.

Download Edwards and Gray supplementary movie(Video)
Video 6.5 MB

Edwards and Gray supplementary movie

A flow of carborundum particles, from left to right, on a chute inclined at 35.1 degrees to the horizontal viewed through the glass sidewalls, with a visible region here that is approximately 16cm in length and near to the midpoint of the downslope $x$-direction, in alignment with the chute to give the flow thickness profile on the vertical axis. The positions of the spherical glass beads which form the rough bed of the chute are visible on the right-hand side as white circles. It is observed that the waves erode at material below the thickness of the initial static uniform layer, but not all the way down to the bed.

Download Edwards and Gray supplementary movie(Video)
Video 10.8 MB

Edwards and Gray supplementary movie

Flow thickness $h$ varying with downslope position $x$, obtained from numerical simulations on a periodic domain with travelling-wave solution, shown in figure~\ref{fig9}(a), as the initial conditions and a 2nd-order Runge-Kutta time-stepper. The flow thickness profile is observed to remain unchanged, aside from small variations in the constant thickness $h_{+}$ of the stationary layer, after the given period of time has elapsed. These variations are greater than when a 3rd-order adaptive Runge-Kutta time-stepper is used (movie 3).

Download Edwards and Gray supplementary movie(Video)
Video 13.3 MB

Edwards and Gray supplementary movie

Flow thickness $h$ varying with downslope position $x$, obtained from numerical simulations on a periodic domain with travelling-wave solution, shown in figure~\ref{fig9}(a), as the initial conditions and a 3rd-order adaptive Runge-Kutta time-stepper. The flow thickness profile is observed to remain unchanged, aside from small variations in the constant thickness $h_{+}$ of the stationary layer, after the given period of time has elapsed. These variations are smaller than when a 2nd-order Runge-Kutta time-stepper is used (movie 4).

Download Edwards and Gray supplementary movie(Video)
Video 10.7 MB

Edwards and Gray supplementary movie

The flow thickness $h$ obtained by a numerical simulation in a periodic domain with initial conditions $h(x,t=0)=h_{\mathrm{stop}} + 10^{-4}H(x)$, where $H(x)\in[-1,1]$ is a zero-mean pseudo-random perturbation to the thickness $h_{\mathrm{stop}}$ of a steady uniform flow. The roll wave instability causes the perturbation to grow and eventually coarsen to form one solitary, steady travelling erosion-deposition wave, along with a region of stationary material, which fills the length of the domain.

Download Edwards and Gray supplementary movie(Video)
Video 13.9 MB

Edwards and Gray supplementary movie

Results of a numerical simulation showing the flow thickness $h$ and the depth-averaged velocity $\bar{u}$ with downslope position $x$(m) (solid lines). At the inflow boundary, a flow thickness $h(x=0,t)=h_0 + 10^{-4}H(t)$ is prescribed, where $H(t)=\sin{(2\pi f t)}$ is a sinusoidal perturbation, with $f=0.47$Hz, to a steady uniform flow, of thickness $h_0=h_{\mathrm{stop}}$ and corresponding depth-averaged velocity $\bar{u}_0=\bar{u}_{\mathrm{stop}}$, imposed as an initial condition (dashed lines). Erosion-deposition waves of the inflow frequency, $f=0.47$Hz, grow downslope of the inflow gate and the important experimental flow feature of stationary regions between waves, where $\bar{u}=0$, is captured numerically.

Download Edwards and Gray supplementary movie(Video)
Video 9.2 MB

Edwards and Gray supplementary movie

Results of a numerical simulation showing the flow thickness $h$ and the depth-averaged velocity $\bar{u}$ with downslope position $x$(m) (solid lines). At the inflow boundary, a flow thickness $h(x=0,t)=h_0 + 10^{-4}H(t)$ is prescribed, where $H(t)=\sin{(2\pi f t)}$ is a sinusoidal perturbation, with $f=0.47$Hz, to a steady uniform flow, of thickness $h_0=1.2 h_{\mathrm{stop}}$ and corresponding depth-averaged velocity $\bar{u}_0 =(1.2)^{3/2}\bar{u}_{\mathrm{stop}}$, imposed as an initial condition (dashed-dotted lines). The minimum flow thickness and velocity for which a steady uniform flow is possible, $h_{\mathrm{stop}}$ and $\bar{u}_{\mathrm{stop}}$ respectively, are also shown (dashed lines). Continuous roll waves of the inflow frequency, $f=0.47$Hz, grow downslope of the inflow gate.

Download Edwards and Gray supplementary movie(Video)
Video 9.8 MB

Edwards and Gray supplementary movie

Results of a numerical simulation showing the flow thickness $h$ and the depth-averaged velocity $\bar{u}$ with downslope position $x$(m) (solid lines). At the inflow boundary, a flow thickness $h(x=0,t)=h_0 + 10^{-4}H(t)$ is prescribed, where $H(t)=\sin{(2\pi f t)}$ is a sinusoidal perturbation, with $f=0.47$Hz, to a steady uniform flow, of thickness $h_0=1.2 h_{\mathrm{stop}}$ and corresponding depth-averaged velocity $\bar{u}_0 =(1.2)^{3/2}\bar{u}_{\mathrm{stop}}$, imposed as an initial condition (dashed-dotted lines). The minimum flow thickness and velocity for which a steady uniform flow is possible, $h_{\mathrm{stop}}$ and $\bar{u}_{\mathrm{stop}}$ respectively, are also shown (dashed lines). Continuous roll waves of the inflow frequency, $f=0.47$Hz, grow downslope of the inflow gate.

Download Edwards and Gray supplementary movie(Video)
Video 9.1 MB

Edwards and Gray supplementary movie

Results of a numerical simulation showing the flow thickness $h$ and the depth-averaged velocity $\bar{u}$ with downslope position $x$(m) (solid lines). At the inflow boundary, a flow thickness $h(x=0,t)=h_0 + 10^{-4}H(t)$ is prescribed, where $H(t) \in [-1,1]$ is a zero-mean, pseudo-random perturbation to a steady uniform flow, of thickness $h_0=1.2 h_{\mathrm{stop}}$ and corresponding depth-averaged velocity $\bar{u}_0 = (1.2)^{3/2}\bar{u}_{\mathrm{stop}}$, imposed as an initial condition (dashed-dotted lines). The minimum flow thickness and velocity for which a steady uniform flow is possible, $h_{\mathrm{stop}}$ and $\bar{u}_{\mathrm{stop}}$ respectively, are also shown (dashed lines). Continuous roll waves of a range of frequencies grow downslope of the inflow gate and there are mergers of waves with different characteristics.

Download Edwards and Gray supplementary movie(Video)
Video 7.5 MB