Article contents
Enumeration, orthogonality and completeness of the incompressible Coriolis modes in a sphere
Published online by Cambridge University Press: 04 February 2015
Abstract
We consider incompressible flows in the rapid-rotation limit of small Rossby number and vanishing Ekman number, in a bounded volume with a rigid impenetrable rotating boundary. Physically the flows are inviscid, almost rigid rotations. We interpret the Coriolis force, modified by a pressure gradient, as a linear operator acting on smooth inviscid incompressible flows in the volume. The eigenfunctions of the Coriolis operator
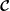 $\boldsymbol{{\mathcal{C}}}$
so defined are the inertial modes (including any Rossby modes) and geostrophic modes of the rotating volume. We show
$\boldsymbol{{\mathcal{C}}}$
so defined are the inertial modes (including any Rossby modes) and geostrophic modes of the rotating volume. We show
 $\boldsymbol{{\mathcal{C}}}$
is a bounded operator and that
$\boldsymbol{{\mathcal{C}}}$
is a bounded operator and that
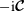 $-\text{i}\boldsymbol{{\mathcal{C}}}$
is symmetric, so that the Coriolis modes of different frequencies are orthogonal. We prove that the space of incompressible polynomial flows of degree
$-\text{i}\boldsymbol{{\mathcal{C}}}$
is symmetric, so that the Coriolis modes of different frequencies are orthogonal. We prove that the space of incompressible polynomial flows of degree
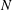 $N$
or less in a sphere is invariant under
$N$
or less in a sphere is invariant under
 $\boldsymbol{{\mathcal{C}}}$
. The symmetry of
$\boldsymbol{{\mathcal{C}}}$
. The symmetry of
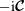 $-\text{i}\boldsymbol{{\mathcal{C}}}$
thus implies the Coriolis operator is non-defective on the finite-dimensional space of spherical polynomial flows. This enables us to enumerate the Coriolis modes, and to establish their completeness using the Weierstrass polynomial approximation theorem. The fundamental tool, which is required to establish invariance of spherical polynomial flows under
$-\text{i}\boldsymbol{{\mathcal{C}}}$
thus implies the Coriolis operator is non-defective on the finite-dimensional space of spherical polynomial flows. This enables us to enumerate the Coriolis modes, and to establish their completeness using the Weierstrass polynomial approximation theorem. The fundamental tool, which is required to establish invariance of spherical polynomial flows under
 $\boldsymbol{{\mathcal{C}}}$
and completeness, is that the solution of the polynomial Poisson–Neumann problem, i.e. Poisson’s equation with a Neumann boundary condition and polynomial data, in a sphere is a polynomial. We also enumerate the Coriolis modes in a sphere, with careful consideration of the geostrophic modes, directly from the known analytic solutions.
$\boldsymbol{{\mathcal{C}}}$
and completeness, is that the solution of the polynomial Poisson–Neumann problem, i.e. Poisson’s equation with a Neumann boundary condition and polynomial data, in a sphere is a polynomial. We also enumerate the Coriolis modes in a sphere, with careful consideration of the geostrophic modes, directly from the known analytic solutions.
- Type
- Papers
- Information
- Copyright
- © 2015 Cambridge University Press
References
- 23
- Cited by



