Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-26T06:12:47.608Z Has data issue: false hasContentIssue false
  • English
  • Français

Entrainment by turbulent fountains

Published online by Cambridge University Press:  04 February 2016

H. C. Burridge  and
G. R. Hunt
H. C. Burridge
Affiliation:
Department of Applied Mathematics and Theoretical Physics, University of Cambridge, Centre for Mathematical Sciences, Wilberforce Road, Cambridge CB3 0WA, UK
G. R. Hunt*
Affiliation:
Department of Engineering, University of Cambridge, Trumpington Street, Cambridge CB2 1PZ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

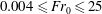Experimental measurements of entrainment by turbulent fountains from circular sources in quiescent uniform environments are presented. Our results span almost four orders of magnitude in the source Froude number ($0.004\leqslant \mathit{Fr}_{0}\leqslant 25$) and thereby encompass the entrainment across all classes of fountain behaviour identified to date. We identify scalings for the entrained volume flux 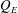$Q_{E}$, in terms of 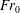$\mathit{Fr}_{0}$ and the source volume flux 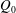$Q_{0}$, within a number of distinct Froude-number bands corresponding to each class of fountain. Additionally we identify a distinct class of new behaviour, as yet unreported, for 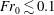$\mathit{Fr}_{0}\lesssim 0.1$.

JFM classification

Wakes/Jets: Jets Convection: Plumes/thermals Turbulent Flows: Turbulent convection
Type
Papers
Information
Journal of Fluid Mechanics , Volume 790 , 10 March 2016 , pp. 407 - 418
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Baines, W. D. 1975 Entrainment by a plume or jet at a density interface. J. Fluid Mech. 68 (2), 309–320.CrossRefGoogle Scholar
Baines, W. D. 1983 A technique for the direct measurement of volume flux of a plume. J. Fluid Mech. 132, 247–256.CrossRefGoogle Scholar
Baines, W. D., Corriveau, A. F. & Reedman, T. J. 1993 Turbulent fountains in a closed chamber. J. Fluid Mech. 255, 621–646.CrossRefGoogle Scholar
Baines, W. D., Turner, J. S. & Campbell, I. H. 1990 Turbulent fountains in an open chamber. J. Fluid Mech. 212, 557–592.CrossRefGoogle Scholar
Bloomfield, L. J. & Kerr, R. C. 1998 Turbulent fountains in a stratified fluid. J. Fluid Mech. 358, 335–356.Google Scholar
Burridge, H. C. & Hunt, G. R. 2013 The rhythm of fountains: the length and time scales of rise height fluctuations at low and high Froude numbers. J. Fluid Mech. 728, 91–119.Google Scholar
Burridge, H. C. & Hunt, G. R. 2014 Scaling arguments for the fluxes in turbulent miscible fountains. J. Fluid Mech. 744, 273–285.CrossRefGoogle Scholar
Burridge, H. C., Mistry, A. & Hunt, G. R. 2015 The effect of source Reynolds number on the rise height of a fountain. Phys. Fluids 27 (4), 047101.Google Scholar
Carazzo, G., Kaminski, E. & Tait, S. 2010 The rise and fall of turbulent fountains: a new model for improved quantitative predictions. J. Fluid Mech. 657, 265–284.Google Scholar
Cardoso, S. S. S. & Woods, A. W. 1993 Mixing by a turbulent plume in a confined stratified region. J. Fluid Mech. 250, 277–305.CrossRefGoogle Scholar
Cresswell, R. W. & Szczepura, R. T. 1993 Experimental investigation into a turbulent jet with negative buoyancy. Phys. Fluids 11, 2865–2878.Google Scholar
Ezzamel, A., Salizzoni, P. & Hunt, G. R. 2015 Dynamical variability of axisymmetric buoyant plumes. J. Fluid Mech. 765, 576–611.Google Scholar
Hunt, G. R. & Burridge, H. C. 2015 Fountains in industry and nature. Annu. Rev. Fluid Mech. 47, 195–220.CrossRefGoogle Scholar
Kaye, N. B. & Hunt, G. R. 2006 Weak fountains. J. Fluid Mech. 558, 319–328.Google Scholar
Kumagai, M. 1984 Turbulent buoyant convection from a source in a confined two-layered region. J. Fluid Mech. 147, 105–131.Google Scholar
Lin, W. E. & Armfield, S. W. 2000 Very weak fountains in a homogeneous fluid. Numer. Heat Transfer A 38, 377–396.Google Scholar
Lin, Y. J. P. & Linden, P. F. 2005 The entrainment due to a turbulent fountain at a density interface. J. Fluid Mech. 542, 25–52.Google Scholar
Morton, B. R. 1959 Forced plumes. J. Fluid Mech. 5, 151–163.CrossRefGoogle Scholar
Morton, B. R., Taylor, G. & Turner, J. S. 1956 Turbulent gravitational convection from maintained and instantaneous sources. Proc. R. Soc. Lond. A 234, 1–23.Google Scholar
Shrinivas, A. B. & Hunt, G. R. 2014 Unconfined turbulent entrainment across density interfaces. J. Fluid Mech. 757, 573–598.CrossRefGoogle Scholar
Sutherland, B. R. 2010 Internal Gravity Waves. Cambridge University Press.CrossRefGoogle Scholar
Williamson, N., Armfield, S. W. & Lin, W. 2011 Forced turbulent fountain flow behaviour. J. Fluid Mech. 671, 535–558.CrossRefGoogle Scholar