Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-08T08:19:53.227Z Has data issue: false hasContentIssue false
  • English
  • Français

The enstrophy cascade in forced two-dimensional turbulence

Published online by Cambridge University Press:  31 January 2011

ANDREAS VALLGREN  and
ERIK LINDBORG
ANDREAS VALLGREN*
Affiliation:
Linné FLOW Centre, KTH Mechanics, SE-100 44 Stockholm, Sweden
ERIK LINDBORG
Affiliation:
Linné FLOW Centre, KTH Mechanics, SE-100 44 Stockholm, Sweden
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

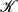We carry out direct numerical simulations of two-dimensional turbulence with forcing at different wavenumbers and resolutions up to 327682 grid points. In the absence of large-scale drag, a state is reached where enstrophy is quasi-stationary while energy is growing. In the enstrophy cascade range the energy spectrum has the form E(k) = εω2/3k−3, without any logarithmic correction, where εω is the enstrophy dissipation and 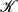is of the order of unity. However, 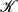is varying between different simulations and is thus not a perfect constant. This variation can be understood as a consequence of large-scale dissipation intermittency, following the argument by Landau (Landau & Lifshitz, Fluid Mechanics, 1959, Pergamon). In the presence of a large-scale drag, we obtain a slightly steeper spectrum. When forcing is applied at a scale which is somewhat smaller than the computational domain, no vortices are formed, and the statistics remain close to Gaussian in the enstrophy cascade range. When forcing is applied at a smaller scale, long-lived coherent vortices form at larger scales than the forcing scale, and intermittency measures become very large at all scales, including the scales of the enstrophy cascade. We conclude that the enstrophy cascade with a k−3-spectrum is a robust feature of the two-dimensional Navier–Stokes equations. However, there is a complete lack of universality of higher-order statistics of vorticity increments in the enstrophy cascade range.

JFM classification

Turbulent Flows: Homogeneous turbulence Turbulent Flows: Turbulence simulation Turbulent Flows: Turbulence theory
Type
Papers
Information
Journal of Fluid Mechanics , Volume 671 , 25 March 2011 , pp. 168 - 183
Copyright
Copyright © Cambridge University Press 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Alvelius, K. 1999 Random forcing of three-dimensional homogeneous turbulence. Phys. Fluids 11, 1880.CrossRefGoogle Scholar
Boffetta, G. 2007 Energy and enstrophy fluxes in the double cascade of two-dimensional turbulence. J. Fluid Mech. 589, 253.CrossRefGoogle Scholar
Boffetta, G., Celani, A., Musacchio, S. & Vergassola, M. 2002 Intermittency in two-dimensional Ekman–Navier–Stokes turbulence. Phys. Rev. E 66, 026304.CrossRefGoogle ScholarPubMed
Borue, V. 1993 Spectral exponents of enstrophy cascade in stationary two-dimensional homogeneous turbulence. Phys. Rev. Lett. 71, 3967.CrossRefGoogle ScholarPubMed
Borue, V. 1994 Inverse energy cascade in stationary two-dimensional homogeneous turbulence. Phys. Rev. Lett. 72, 1475.CrossRefGoogle ScholarPubMed
Bracco, A. & McWilliams, J. 2010 Reynolds-number dependency in homogeneous, stationary two-dimensional turbulence. J. Fluid Mech. 646, 517.CrossRefGoogle Scholar
Canuto, C., Hussaini, M. Y., Quarteroni, A. & Zang, T. A. 1988 Spectral Methods in Fluid Dynamics. Springer.CrossRefGoogle Scholar
Chertkov, M., Connaughton, C., Kolokolov, I. & Lebedev, V. 2008 Dynamics of energy condensation in two-dimensional turbulence. Phys. Rev. Lett. 99, 084501.CrossRefGoogle Scholar
Danilov, S. & Gurarie, D. 2001 Forced two-dimensional turbulence in spectral and physical space. Phys. Rev. E 63, 061208.CrossRefGoogle ScholarPubMed
Eyink, G. L. 1996 Exact results on stationary turbulence in 2D: consequences of vorticity conservation. Physica D 91, 97.CrossRefGoogle Scholar
Falkovich, G. & Lebedev, V. 1994 Universal direct cascade in two-dimensional turbulence. Phys. Rev. E 50, 3883.CrossRefGoogle ScholarPubMed
Ishihara, T. & Kaneda, Y. 2001 Energy spectrum in the enstrophy transfer range of two-dimensional forced turbulence. Phys. Fluids 13, 544.CrossRefGoogle Scholar
Kaneda, Y. 1987 Inertial range of two-dimensional turbulence in a Lagrangian renormalized approximation. Phys. Fluids 30, 2672.CrossRefGoogle Scholar
Kraichnan, R. H. 1967 Inertial ranges in two-dimensional turbulence. Phys. Fluids 10, 1417.CrossRefGoogle Scholar
Kraichnan, R. H. 1971 Inertial-range transfer in two- and three-dimensional turbulence. J. Fluid Mech. 47, 525.CrossRefGoogle Scholar
Kraichnan, R. H. 1974 On Kolmogorov's inertial-range theories. J. Fluid Mech. 62, 305.CrossRefGoogle Scholar
Landau, L. D. & Lifshitz, E. M. 1959 Fluid Mechanics, 1st edn. Pergamon.Google Scholar
Legras, B., Santangelo, P. & Benzi, R. 1988 High-resolution numerical experiments for forced two-dimensional turbulence. Europhys. Lett. 5, 37.CrossRefGoogle Scholar
Lindborg, E. 1999 Can the atmospheric kinetic energy spectrum be explained by two-dimensional turbulence? J. Fluid Mech. 388, 259.CrossRefGoogle Scholar
Lindborg, E. & Alvelius, K. 2000 The kinetic energy spectrum of the two-dimensional enstrophy turbulence cascade. Phys. Fluids 12, 945.CrossRefGoogle Scholar
Lindborg, E. & Brethouwer, G. 2007 Stratified turbulence forced in rotational and divergent modes. J. Fluid Mech. 586, 83.CrossRefGoogle Scholar
Maltrud, M. E. & Vallis, G. K. 1991 Energy spectra and coherent structures in forced two-dimensional and beta-plane turbulence. J. Fluid Mech. 228, 321.Google Scholar
McWilliams, J. 1989 Statistical properties of decaying geostrophic turbulence. J. Fluid Mech. 198, 199.CrossRefGoogle Scholar
McWilliams, J. 1990 The vortices of two-dimensional turbulence. J. Fluid Mech. 219, 361.CrossRefGoogle Scholar
Nam, K., Ott, E., Antonsen, T. M. & Guzdar, P. N. 2000 Lagrangian chaos and the effect of drag on the enstrophy cascade in two-dimensional turbulence. Phys. Rev. Lett. 84, 5134.CrossRefGoogle ScholarPubMed
Ohkitani, K. 1991 Wave number space dynamics of enstrophy cascade in a forced two-dimensional turbulence. Phys. Fluids A 3, 1598.CrossRefGoogle Scholar
Pasquero, C. & Falkovich, G. 2002 Stationary spectrum of vorticity cascade in two-dimensional turbulence. Phys. Rev. E 65, 056305.CrossRefGoogle ScholarPubMed
Scott, R. K. 2007 Nonrobustness of the two-dimensional turbulent inverse cascade. Phys. Rev. E 75, 046301.CrossRefGoogle ScholarPubMed
Tran, C. V. 2007 Constraints on inertial range scaling laws in forced two-dimensional Navier–Stokes turbulence. Phys. Fluids 19, 108109.CrossRefGoogle Scholar
Tran, C. V. & Bowman, J. C. 2004 Robustness of the inverse cascade in two-dimensional turbulence. Phys. Rev. E 69, 036303.CrossRefGoogle ScholarPubMed
Vallgren, A. 2010 Infrared Reynolds number dependency of the two-dimensional inverse energy cascade. J. Fluid Mech. 667, 465–475.Google Scholar