Published online by Cambridge University Press: 12 February 2015
Hydrodynamic interactions between two identical elastic dumbbells settling under gravity in a viscous fluid at low Reynolds number are investigated using the point-particle model. The evolution of a benchmark initial configuration is studied, in which the dumbbells are vertical and their centres are aligned horizontally. Rigid dumbbells and pairs of separate beads starting from the same positions tumble periodically while settling. We find that elasticity (which breaks the time-reversal symmetry of the motion) significantly affects the system dynamics. This is remarkable when taking into account that elastic forces are always much smaller than gravity. We observe oscillating motion of the elastic dumbbells, which tumble and change their length non-periodically. Independently of the value of the spring constant, a horizontal hydrodynamic repulsion appears between the dumbbells: their centres of mass move apart from each other horizontally. This motion is fast for moderate values of the spring constant  $k$, and slows down when
$k$, and slows down when 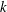 $k$ tends to zero or to infinity; in these limiting cases we recover the periodic dynamics reported in the literature. For moderate values of the spring constant, and different initial configurations, we observe the existence of a universal time-dependent solution to which the system converges after an initial relaxation phase. The tumbling time and the width of the trajectories in the centre-of-mass frame increase with time. In addition to its fundamental significance, the benchmark solution presented here is important to understanding general features of systems with a larger number of elastic particles, in regular and random configurations.
$k$ tends to zero or to infinity; in these limiting cases we recover the periodic dynamics reported in the literature. For moderate values of the spring constant, and different initial configurations, we observe the existence of a universal time-dependent solution to which the system converges after an initial relaxation phase. The tumbling time and the width of the trajectories in the centre-of-mass frame increase with time. In addition to its fundamental significance, the benchmark solution presented here is important to understanding general features of systems with a larger number of elastic particles, in regular and random configurations.
Two elastic dumbbells with the spring constant k=0.01, settling under gravity in a viscous fluid. Movie, based on the point-particle model, is taken in the centre-of-mass reference frame, for two initial configurations with the aspect ratios Cin=1 and Cin=1.8. Initially, the spring length L=1 (the equilibrium value). Hydrodynamic repulsion of the dumbbells and oscillations of their lengths are visible.
Two elastic dumbbells with the spring constant k=0.01, settling under gravity in a viscous fluid. Movie, based on the point-particle model, is taken in the centre-of-mass reference frame, for two initial configurations with the aspect ratios Cin=1 and Cin=1.8. Initially, the spring length L=1 (the equilibrium value). Hydrodynamic repulsion of the dumbbells and oscillations of their lengths are visible.
Two elastic dumbbells with the spring constant k=0.1, settling under gravity in a viscous fluid. Movie, based on the point-particle model, is taken in the centre-of-mass reference frame, for two initial configurations with the aspect ratios Cin=1 and Cin=1.8. Initially, the spring length L=1 (the equilibrium value). Hydrodynamic repulsion of the dumbbells and oscillations of their lengths are visible.
Two elastic dumbbells with the spring constant k=0.1, settling under gravity in a viscous fluid. Movie, based on the point-particle model, is taken in the centre-of-mass reference frame, for two initial configurations with the aspect ratios Cin=1 and Cin=1.8. Initially, the spring length L=1 (the equilibrium value). Hydrodynamic repulsion of the dumbbells and oscillations of their lengths are visible.