Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-24T01:06:05.606Z Has data issue: false hasContentIssue false
  • English
  • Français

The dynamics of an insulating plate over a thermally convecting fluid and its implication for continent movement over convective mantle

Published online by Cambridge University Press:  11 April 2019

Yadan Mao Open the ORCID record for Yadan Mao [Opens in a new window] ,
Jin-Qiang Zhong Open the ORCID record for Jin-Qiang Zhong [Opens in a new window]  and
Jun Zhang
Yadan Mao*
Affiliation:
Hubei Subsurface Multi-scale Imaging Key Laboratory, Institute of Geophysics and Geomatics, China University of Geosciences, Wuhan 430074, China
Jin-Qiang Zhong
Affiliation:
School of Physics Science and Engineering, Tongji University, Shanghai 200092, China
Jun Zhang
Affiliation:
Courant Institute, and Department of Physics, New York University, New York, NY 10012, USA NYU-ECNU Institute of Physics, NYU Shanghai, Shanghai 200062, China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Continents exert a thermal blanket effect to the mantle underneath by locally accumulating heat and modifying the flow structures, which in turn affects continent motion. This dynamic feedback is studied numerically with a simplified model of an insulating plate over a thermally convecting fluid with infinite Prandtl number at Rayleigh numbers of the order of 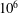$10^{6}$. Several plate–fluid coupling modes are revealed as the plate size varies. In particular, small plates show long durations of stagnancy over cold downwellings. Between long stagnancies, bursts of velocity are observed when the plate rides on a single convection cell. As plate size increases, the coupled system transitions to another type of short-lived stagnancy, in which case hot plumes develop underneath. For an even larger plate, a unidirectional moving mode emerges as the plate modifies impeding flow structures it encounters while maintaining a single convection cell underneath. These identified modes are reminiscent of some real cases of continent–mantle coupling. Results show that the capability of a plate to overcome impeding flow structures increases with plate size, Rayleigh number and intensity of internal heating. Compared to cases with a fixed plate, cases with a freely drifting plate are associated with higher Nusselt number and more convection cells within the flow domain.

JFM classification

Convection: Convection in cavities Geophysical and Geological Flows: Mantle convection
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 868 , 10 June 2019 , pp. 286 - 315
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503–537.Google Scholar
Bunge, H.-P. & Richards, M. A. 1996 The origin of large scale structure in mantle convection: effects of plate motions and viscosity stratification. Geophys. Res. Lett. 23, 2987–2990.Google Scholar
Bunge, H.-P., Richards, M. A. & Baumgardner, J.-R. 1996 Effect of depth-dependent viscosity on the planform of mantle convection. Nature 379, 436–438.Google Scholar
Bunge, H.-P., Richards, M. A. & Baumgardner, J. R. 1997 A sensitivity study of three-dimensional spherical mantle convection at 108 Rayleigh number: effects of depth-dependent viscosity, heating mode, and an endothermic phase change. J. Geophys. Res. 102, 11991–12007.Google Scholar
Burke, K. & Torsvik, T. H. 2004 Derivation of large igneous provinces of the past 200 million years from long-term heterogeneities in the deep mantle. Earth Planet. Sci. Lett. 227, 531–538.Google Scholar
Cande, S. C. & Stock, J. M. 2004 Pacific–Antarctic–Australia motion and the formation of the Macquarie plate. Geophys. J. Intl 157, 399–414.Google Scholar
Davies, G. F. 1988 Role of the lithosphere in mantle convection. J. Geophys. Res. 93, 10451–10466.Google Scholar
Ebinger, C. J. & Sleep, N. H. 1998 Cenozoic magmatism throughout east Africa resulting from impact of a single plume. Nature 395, 788–791.Google Scholar
Elder, J. 1967 Convective self-propulsion of continents. Nature 214, 657–750.Google Scholar
Gable, C. W., O’Connell, R. J. & Travis, B. J. 1991 Convection in three dimensions with surface plates: generation of toroidal flow. J. Geophys. Res 96, 8391–8405.Google Scholar
Grigné, C., Labrosse, S. & Tackley, P. J. 2005 Convective heat transfer as a function of wavelength: implications for the cooling of the Earth. J. Geophys. Res. 110, B03409.Google Scholar
Grigné, C., Labrosse, S. & Tackley, P. J. 2007 Convection under a lid of finite conductivity in wide aspect ratio models: effect of continents on the wavelength of mantle flow. J. Geophys. Res. 112, B08403.Google Scholar
Gurnis, M. 1988 Large-scale mantle convection and aggregation and dispersal of supercontinents. Nature 313, 541–546.Google Scholar
Heron, P. & Lowman, J. P. 2011 The effects of supercontinent size and thermal insulation on the formation of mantle plumes. Tectonophysics 510, 28–38.Google Scholar
Höink, T. & Lenardic, A. 2008 Three-dimensional mantle convection simulations with a low-viscosity asthenosphere and the relationship between heat flow and the horizontal length scale of convection. Geophys. Res. Lett. 35, L10304.Google Scholar
Honda, S., Yoshida, M., Ootorii, S. & Iwase, Y. 2000 The timescales of plume generation caused by continental aggregation. Earth Planet. Sci. Lett. 176, 31–43.Google Scholar
Howard, L. N., Malkus, W. V. R. & Whitehead, J. A. 1970 Self-convection of floating heat sources: a model for continental drift. Geophys. Fluid Dyn. 1, 123–142.Google Scholar
Jarvis, G. T. & Peltier, W. R. 1989 Convection models and geophysical observations. In Mantle Convection: Plate Tectonics and Global Dynamics (ed. Peltier, W. R.), pp. 479–594. Gordon and Breach.Google Scholar
King, S. D. 1995 The viscosity structure of the mantle. Rev. Geophys. 33, 11–17.Google Scholar
Lenardic, A., Moresi, L., Jellinek, A. M. & Manga, M. 2005 Continental insulation, mantle cooling, and the surface area of oceans and continents. Earth Planet. Sci. Lett. 234, 317–333.Google Scholar
Lenardic, A., Richards, M. A. & Busse, F. H. 2006 Depth-dependent rheology and the horizontal length scale of mantle convection. J. Geophys. Res. 111, B07404.Google Scholar
Leonard, B. P. 1979 A stable and accurate convective modelling procedure based on quadratic upstream interpolation. Comput. Meth. Appl. Mech. Engng 19, 59–98.Google Scholar
Lithgow-Bertelloni, C. & Silver, P. G. 1998 Dynamic topography, plate driving forces and the African superswell. Nature 395, 269–272.Google Scholar
Lowman, J. P. & Gable, C. W. 1999 Thermal evolution of the mantle following continental aggregation in 3D convection models. Geophys. Res. Lett. 26, 2649–2652.Google Scholar
Lowman, J. P. & Jarvis, G. T. 1993 Mantle convection flow reversals due to continental collisions. Geophys. Res. Lett. 20, 2087–2090.Google Scholar
Lowman, J. P. & Jarvis, G. T. 1995 Mantle convection models of continental collision and breakup incorporating finite thickness plates. Phys. Earth Planet. Inter. 88, 53–68.Google Scholar
Lowman, J. P. & Jarvis, G. T. 1999 Effects of mantle heat source distribution on supercontinent stability. J. Geophys. Res. 104, B6, 12733–12746.Google Scholar
Lowman, J., King, S. D. & Gable, C. W. 2001 The influence of tectonic plates on mantle convection patterns, temperature and heat flow. Geophys. J. Intl 146, 619–636.Google Scholar
Mao, Y., Lei, C. & Patterson, J. C. 2009 Unsteady natural convection in a triangular enclosure induced by absorption of radiation – a revisit by improved scaling analysis. J. Fluid Mech. 622, 75–102.Google Scholar
Mao, Y., Lei, C. & Patterson, J. C. 2010 Unsteady near-shore natural convection induced by surface cooling. J. Fluid Mech. 642, 213–233.Google Scholar
Mitrovica, J. X. 1996 Haskell [1935] revisited. J. Geophys. Res. 101, 555–569.Google Scholar
Monnereau, M. & Quéré, S. 2001 Spherical shell models of mantle convection with tectonic plates. Earth Planet. Sci. Lett. 184, 575–587.Google Scholar
Patankar, S. V. 1980 Numerical Heat Transfer and Fluid Flow. Taylor & Francis.Google Scholar
Phillips, B. R. & Bunge, H.-P. 2005 Heterogeneity and time dependence in 3D spherical mantle convection models with continental drift. Earth Planet. Sci. Lett. 233, 121–135.Google Scholar
Pollack, H. N., Hurter, S. J. & Johnson, J. R. 1993 Heat flow from the Earth’s interior: analysis of the global data set. Rev. Geophys. 31, 267–280.Google Scholar
Ritsema, J., van Heijst, H. J. & Woodhouse, J. H. 1999 Complex shear wave velocity structure imaged beneath Africa and Iceland. Science 286, 1925–1928.Google Scholar
Schubert, G., Turcotte, D. L. & Olson, P. 2001 Mantle Convection in the Earth and Planets. Cambridge University Press, 940 pp.Google Scholar
Suggate, R. P., Stevens, G. R. & Te Punga, M. T. 1978 The Geology of New Zealand. Department of Scientific and Industrial Research.Google Scholar
Sutherland, R. 1999 Basement geology and tectonic development of the greater New Zealand region: an interpretation from regional magnetic data. Tectonophysics 308, 341–362.Google Scholar
Tackley, P. J. 1996 On the ability of phase transitions and viscosity layering to induce long wavelength heterogeneity in the mantle. Geophys. Res. Lett. 23, 1985–1988.Google Scholar
Torsvik, T. H., Burke, K., Steinberger, B., Webb, S. J. & Ashwal, L. D. 2010 Diamonds sampled by plumes from the core–mantle boundary. Nature 466, 352–355.Google Scholar
Torsvik, T. H., Steinberger, B., Cocks, L. R. M. & Burke, K. 2008 Longitude: linking Earth’s ancient surface to its deep interior. Earth Planet. Sci. Lett. 276, 273–282.Google Scholar
Turcotte, D. & Schubert, G. 2002 Geodynamics, 2nd edn. Cambridge University Press.Google Scholar
Whitehead, J. A. 1972 Moving heaters as a model of continental drift. Phys. Earth Planet. Inter. 5, 199–212.Google Scholar
Whitehead, J. A. & Behn, M. D. 2015 The continental drift convection cell. Geophys. Res. Lett. 42, 4301–4308.Google Scholar
Whitehead, J. A., Shea, E. & Behn, M. D. 2011 Cellular convection in a chamber with a warm surface raft. Phys. Fluids 23, 104103.Google Scholar
Zhang, J. & Libchaber, A. 2000 Periodic boundary motion in thermal turbulence. Phys. Rev. Lett. 84, 4361–4364.Google Scholar
Zhong, J.-Q. & Zhang, J. 2005 Thermal convection with a freely moving top boundary. Phys. Fluids 17, 115105.Google Scholar
Zhong, J.-Q. & Zhang, J. 2007a Dynamical states of a mobile heat blanket on a thermally convecting fluid. Phys. Rev. E 75, 055301.Google Scholar
Zhong, J.-Q. & Zhang, J. 2007b Modeling the dynamics of a free boundary on turbulent thermal convection. Phys. Rev. E 76, 016307.Google Scholar
Zhong, S. & Gurnis, M. 1993 Dynamic feedback between a Continent like raft and thermal convection. J. Geophys. Res. 98, 12219–12232.Google Scholar
Zhong, S., Zuber, M. T., Moresi, L. & Gurnis, M. 2000 Role of temperature-dependent viscosity and surface plates in spherical shell models of mantle convection. J. Geophys. Res. 105, 11063–11082.Google Scholar

Mao et al. supplementary movie 1

The evolution of Stagnant Mode I (SM I) for L = 0.5

Download Mao et al. supplementary movie 1(Video)
Video 29.2 MB

Mao et al. supplementary movie 2

The evolution of stagnant mode II (SMI) for L = 1.5

Download Mao et al. supplementary movie 2(Video)
Video 22.7 MB

Mao et al. supplementary movie 3

The unidirectional moving mode (UMM) for L = 2.5

Download Mao et al. supplementary movie 3(Video)
Video 19.6 MB