Article contents
Dynamical similarity and universality of drop size and velocity spectra in sprays
Published online by Cambridge University Press: 07 December 2018
Abstract
Sprays are a class of multiphase flows which exhibit a wide range of drop size and velocity scales spanning several orders of magnitude. The objective of the current work is to experimentally investigate the prospect of dynamical similarity in these flows. We are also motivated to identify a choice of length and time scales which could lead towards a universal description of the drop size and velocity spectra. Towards this end, we have fabricated a cohort of geometrically similar pressure swirl atomizers using micro-electromechanical systems (MEMS) as well as additive manufacturing technology. We have characterized the dynamical characteristics of the sprays as well as the drop size and velocity spectra (in terms of probability density functions, p.d.f.s) over a wide range of Reynolds ( $Re$) and Weber numbers (
$Re$) and Weber numbers (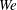 $We$) using high-speed imaging and phase Doppler interferometry, respectively. We show that the dimensionless Sauter mean diameter (
$We$) using high-speed imaging and phase Doppler interferometry, respectively. We show that the dimensionless Sauter mean diameter (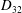 $D_{32}$) scaled to the boundary layer thickness in the liquid sheet at the nozzle exit (
$D_{32}$) scaled to the boundary layer thickness in the liquid sheet at the nozzle exit (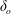 $\unicode[STIX]{x1D6FF}_{o}$) exhibits self-similarity in the core region of the spray, but not in the outer zone. In addition, we show that global drop size spectra in the sprays show two distinct characteristics. The spectra from varying
$\unicode[STIX]{x1D6FF}_{o}$) exhibits self-similarity in the core region of the spray, but not in the outer zone. In addition, we show that global drop size spectra in the sprays show two distinct characteristics. The spectra from varying 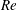 $Re$ and
$Re$ and  $We$ collapse onto a universal p.d.f. for drops of size
$We$ collapse onto a universal p.d.f. for drops of size  $x$ where
$x$ where 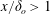 $x/\unicode[STIX]{x1D6FF}_{o}>1$. For
$x/\unicode[STIX]{x1D6FF}_{o}>1$. For 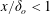 $x/\unicode[STIX]{x1D6FF}_{o}<1$, a residual effect of
$x/\unicode[STIX]{x1D6FF}_{o}<1$, a residual effect of 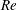 $Re$ and
$Re$ and 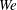 $We$ persists in the size spectra. We explain this characteristic by the fact that the physical mechanisms that cause large drops is different from that which is responsible for the small drops. Similarly, with the liquid sheet velocity at the nozzle exit (
$We$ persists in the size spectra. We explain this characteristic by the fact that the physical mechanisms that cause large drops is different from that which is responsible for the small drops. Similarly, with the liquid sheet velocity at the nozzle exit (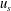 $u_{s}$) as the choice of velocity scale, we show that drops moving with a velocity
$u_{s}$) as the choice of velocity scale, we show that drops moving with a velocity  $u$ such that
$u$ such that 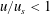 $u/u_{s}<1$ collapse onto a universal p.d.f., while drops with
$u/u_{s}<1$ collapse onto a universal p.d.f., while drops with 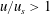 $u/u_{s}>1$ exhibit a residual effect of
$u/u_{s}>1$ exhibit a residual effect of 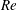 $Re$ and
$Re$ and 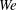 $We$. From these observations, we suggest that physically accurate models for drop size and velocity spectra should rely on piecewise descriptions of the p.d.f. rather than invoking a single mathematical form for the entire distribution. Finally, we show from a dynamical modal analysis that the conical liquid sheet flapping characteristics exhibit a sharp transition in Strouhal number (
$We$. From these observations, we suggest that physically accurate models for drop size and velocity spectra should rely on piecewise descriptions of the p.d.f. rather than invoking a single mathematical form for the entire distribution. Finally, we show from a dynamical modal analysis that the conical liquid sheet flapping characteristics exhibit a sharp transition in Strouhal number ( $St$) at a critical
$St$) at a critical 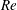 $Re$.
$Re$.
- Type
- JFM Papers
- Information
- Copyright
- © 2018 Cambridge University Press
References
- 32
- Cited by


