Hostname: page-component-669899f699-swprf Total loading time: 0 Render date: 2025-04-25T12:26:14.310Z Has data issue: false hasContentIssue false
  • English
  • Français

Direct numerical simulation of turbulent flow and structures in a circular pipe subjected to axial system rotation

Published online by Cambridge University Press:  25 November 2024

Zhao-Ping Zhang Open the ORCID record for Zhao-Ping Zhang [Opens in a new window]  and
Bing-Chen Wang Open the ORCID record for Bing-Chen Wang [Opens in a new window]
Zhao-Ping Zhang
Affiliation:
Department of Mechanical Engineering, University of Manitoba, Winnipeg, MB R3T 5V6, Canada
Bing-Chen Wang*
Affiliation:
Department of Mechanical Engineering, University of Manitoba, Winnipeg, MB R3T 5V6, Canada
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

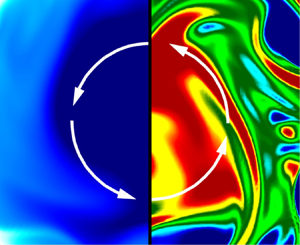
Turbulent circular pipe flows subjected to axial system rotation are studied using direct numerical simulations (DNS) for a wide range of rotation numbers of $Ro_b = 0\unicode{x2013}20$ at a fixed Reynolds number. To ensure that energetic turbulent eddy motions are captured at high rotation numbers, long pipes up to $L_z = 180{\rm \pi} R$ are used in DNS. Two types of energy-containing flow structures have been observed. The first type is hairpin structures that are characteristic of the turbulent boundary layer developing over the pipe wall for both non-rotating and axially rotating flows. The second type is Taylor columns forming at moderate and high rotation numbers. Based on the study of two-point autocorrelation coefficients, it is observed that Taylor columns exhibit quasi-periods in both axial and azimuthal directions. According to the premultiplied spectra, Taylor columns feature one single characteristic axial length scale at the moderate rotation numbers but two at high rotation numbers. It is discovered that the axial system rotation suppresses the sweep events systematically and impedes the formation of hairpin structures. As the rotation number is increased, the turbulence kinetic energy held by Taylor columns enhances rapidly associated with significant increases in their axial length scales.

JFM classification

Turbulent Flows: Pipe flow Turbulent Flows: Rotating turbulence Turbulent Flows: Turbulence simulation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 1000 , 10 December 2024 , A1
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Adrian, R.J. 2007 Hairpin vortex organization in wall turbulence. Phys. Fluids 19 (4), 041301.CrossRefGoogle Scholar
Avsarkisov, V., Hoyas, S., Oberlack, M. & García-Galache, J.P. 2014 Turbulent plane Couette flow at moderately high Reynolds number. J. Fluid Mech. 751, R1.CrossRefGoogle Scholar
Bagheri, E., Wang, B.-C. & Yang, Z. 2020 Influence of domain size on direct numerical simulation of turbulent flow in a moderately curved concentric annular pipe. Phys. Fluids 32 (6), 065105.CrossRefGoogle Scholar
Baltzer, J.R., Adrian, R.J. & Wu, X. 2013 Structural organization of large and very large scales in turbulent pipe flow simulation. J. Fluid Mech. 720, 236–279.CrossRefGoogle Scholar
Bartello, P., Métais, O. & Lesieur, M. 1994 Coherent structures in rotating three-dimensional turbulence. J. Fluid Mech. 273, 1–29.CrossRefGoogle Scholar
Blackburn, H.M. & Sherwin, S.J. 2004 Formulation of a Galerkin spectral element – Fourier method for three-dimensional incompressible flows in cylindrical geometries. J. Comput. Phys. 197, 759–778.CrossRefGoogle Scholar
Buzzicotti, M., Aluie, H., Biferale, L. & Linkmann, M. 2018 Energy transfer in turbulence under rotation. Phys. Rev. Fluids 3, 034802.CrossRefGoogle Scholar
Chin, C., Ooi, A.S.H., Marusic, I. & Blackburn, H.M. 2010 The influence of pipe length on turbulence statistics computed from direct numerical simulation data. Phys. Fluids 22 (11), 115107.CrossRefGoogle Scholar
Ebstein, D. 1998 Reynolds Stresses and Kinetic Energy Budgets in the Flow through an Axially Rotating Pipe, PhD thesis, Sapienza University of Rome, Italy.Google Scholar
Eggels, J.G.M., Unger, F., Weiss, M.H., Westerweel, J., Adrian, R.J., Friedrich, R. & Nieuwstadt, F.T.M. 1994 Fully developed turbulent pipe flow: a comparison between direct numerical simulation and experiment. J. Fluid Mech. 268, 175–210.CrossRefGoogle Scholar
Facciolo, L., Tillmark, N., Talamelli, A. & Alfredsson, P.H. 2007 A study of swirling turbulent pipe and jet flows. Phys. Fluids 19, 035105.CrossRefGoogle Scholar
Fang, X.-J. & Wang, B.-C. 2018 On the turbulent heat transfer in a square duct subjected to spanwise system rotation. Intl J. Heat Fluid Flow 71, 220–230.CrossRefGoogle Scholar
Fang, X.-J., Yang, Z.-X., Wang, B.-C. & Bergstrom, D.J. 2017 Direct numerical simulation of turbulent flow in a spanwise rotating square duct at high rotation numbers. Intl J. Heat Fluid Flow 63, 88–98.CrossRefGoogle Scholar
Feiz, A.A., Ould-Rouis, M. & Lauriat, G. 2003 Large eddy simulation of turbulent flow in a rotating pipe. Intl J. Heat Fluid Flow 24 (3), 412–420.CrossRefGoogle Scholar
Gallet, B. 2015 Exact two-dimensionalization of rapidly rotating large-Reynolds-number flows. J. Fluid Mech. 783, 412–447.CrossRefGoogle Scholar
Grundestam, O., Wallin, S. & Johansson, A.V. 2008 Direct numerical simulations of rotating turbulent channel flow. J. Fluid Mech. 598, 177–199.CrossRefGoogle Scholar
Hirai, S., Takagi, T. & Matsumoto, M. 1988 Predictions of the laminarization phenomena in an axially rotating pipe flow. Trans. ASME J. Fluids Engng 110 (4), 424–430.CrossRefGoogle Scholar
Hoyas, S. & Jiménez, J. 2006 Scaling of the velocity fluctuations in turbulent channels up to $Re_{\tau } = 2003$. Phys. Fluids 18, 011702.CrossRefGoogle Scholar
Imao, S., Itoh, M. & Harada, T. 1996 Turbulent characteristics of the flow in an axially rotating pipe. Intl J. Heat Fluid Flow 17, 444–451.CrossRefGoogle Scholar
Jakirlić, S., Hanjalić, K. & Tropea, C. 2002 Modeling rotating and swirling turbulent flows: a perpetual challenge. AIAA J. 40 (10), 1984–1996.CrossRefGoogle Scholar
Jiménez, J. & Moin, P. 1991 The minimal flow unit in near-wall turbulence. J. Fluid Mech. 225, 213–240.CrossRefGoogle Scholar
van Kan, A. & Alexakis, A. 2020 Critical transition in fast-rotating turbulence within highly elongated domains. J. Fluid Mech. 899, A33.CrossRefGoogle Scholar
Karniadakis, G.E., Israeli, M. & Orszag, S.A. 1991 High-order splitting methods for the incompressible Navier–Stokes equations. J. Comput. Phys. 97 (2), 414–443.CrossRefGoogle Scholar
Karniadakis, G.E. & Sherwin, S.J. 2005 Spectral/hp Element Methods for Computational Fluid Dynamics, 2nd edn. Oxford University Press.CrossRefGoogle Scholar
Kikuyama, K., Murakami, M. & Nishibori, K. 1983 a Development of three-dimensional turbulent boundary layer in an axially rotating pipe. Trans. ASME J. Fluids Engng 105 (2), 154–160.CrossRefGoogle Scholar
Kikuyama, K., Murakami, M., Nishibori, K. & Maeda, K. 1983 b Flow in an axially rotating pipe: a calculation of flow in the saturated region. Bull. JSME 26 (214), 506–513.CrossRefGoogle Scholar
Kristoffersen, R. & Andersson, H.I. 1993 Direct simulations of low-Reynolds-number turbulent flow in a rotating channel. J. Fluid Mech. 256, 163–197.CrossRefGoogle Scholar
Murakami, M. & Kikuyama, K. 1980 Turbulent flow in axially rotating pipes. Trans. ASME J. Fluids Engng 102 (1), 97–103.CrossRefGoogle Scholar
Oberlack, M. 1999 Similarity in non-rotating and rotating turbulent pipe flows. J. Fluid Mech. 379, 1–22.CrossRefGoogle Scholar
Oberlack, M., Cabot, W., Pettersson Reif, B.A. & Weller, T. 2006 Group analysis, direct numerical simulation and modelling of a turbulent channel flow with streamwise rotation. J. Fluid Mech. 562, 383–403.CrossRefGoogle Scholar
Orlandi, P. 1997 Helicity fluctuations and turbulent energy production in rotating and non-rotating pipes. Phys. Fluids 9 (7), 2045–2056.CrossRefGoogle Scholar
Orlandi, P. & Ebstein, D. 2000 Turbulent budgets in rotating pipes by DNS. Intl J. Heat Fluid Flow 21 (5), 499–505.CrossRefGoogle Scholar
Orlandi, P. & Fatica, M. 1997 Direct simulations of turbulent flow in a pipe rotating about its axis. J. Fluid Mech. 343, 43–72.CrossRefGoogle Scholar
Pallares, J. & Davidson, L. 2000 Large-eddy simulations of turbulent flow in a rotating square duct. Phys. Fluids 12 (11), 2878–2894.CrossRefGoogle Scholar
Pestana, T. & Hickel, S. 2020 Rossby-number effects on columnar formation and the energy dissipation law in homogeneous rotating turbulence. J. Fluid Mech. 885, A7.CrossRefGoogle Scholar
Recktenwald, I., Alkishriwi, N. & Schröder, W. 2009 PIV-LES analysis of channel flow rotating about the streamwise axis. Eur. J. Mech. (B/Fluids) 28 (5), 677–688.CrossRefGoogle Scholar
Recktenwald, I., Weller, T., Schröder, W. & Oberlack, M. 2007 Comparison of direct numerical simulations and particle-image velocimetry data of turbulent channel flow rotating about the streamwise axis. Phys. Fluids 19 (8), 085114.CrossRefGoogle Scholar
Reich, G. & Beer, H. 1989 Fluid flow and heat transfer in an axially rotating pipe. I. Effect of rotation on turbulent pipe flow. Intl J. Heat Mass Transfer 32 (3), 551–562.CrossRefGoogle Scholar
Robinson, S.K. 1991 Coherent motions in the turbulent boundary layer. Annu. Rev. Fluid Mech. 23 (1), 601–639.CrossRefGoogle Scholar
Rosas, R.H. & Wang, B.C. 2022 DNS study of turbulent heat transfer in an elliptical pipe flow subjected to system rotation about the major axis. Intl J. Heat Mass Transfer 184, 122230.CrossRefGoogle Scholar
Rosas, R.H., Zhang, Z.P. & Wang, B.C. 2021 Direct numerical simulation of turbulent elliptical pipe flow under system rotation about the major axis. Phys. Rev. Fluids 6 (8), 084609.CrossRefGoogle Scholar
Smith, L.M. & Waleffe, F. 1999 Transfer of energy to two-dimensional large scales in forced, rotating three-dimensional turbulence. Phys. Fluids 11 (6), 1608–1622.CrossRefGoogle Scholar
Speziale, C.G., Younis, B.A. & Berger, S.A. 2000 Analysis and modelling of turbulent flow in an axially rotating pipe. J. Fluid Mech. 407, 1–26.CrossRefGoogle Scholar
Staplehurst, P.J. & Davidson, P.A. 2008 Structure formation in homogeneous freely decaying rotating turbulence. J. Fluid Mech. 598, 81–105.CrossRefGoogle Scholar
Thiele, M. & Müller, W.-C. 2009 Structure and decay of rotating homogeneous turbulence. J. Fluid Mech. 637, 425–442.CrossRefGoogle Scholar
Wallin, S., Grundestam, O. & Johansson, A.V. 2013 Laminarization mechanisms and extreme-amplitude states in rapidly rotating plane channel flow. J. Fluid Mech. 730, 193–219.CrossRefGoogle Scholar
Weller, T. & Oberlack, M. 2006 a DNS of a turbulent channel flow with streamwise rotation – investigation on the cross flow phenomena. In Direct and Large-Eddy Simulation VI (ed. E. Lamballais, R. Friedrich, B.J. Geurts & O. Métais), pp. 241–248. Springer.CrossRefGoogle Scholar
Weller, T. & Oberlack, M. 2006 b DNS of a turbulent channel flow with streamwise rotation – study of the reverse effect of the cross flow. Proc. Appl. Maths. Mech., 6(1):553–554.Google Scholar
Wu, H. & Kasagi, N. 2004 Effects of arbitrary directional system rotation on turbulent channel flow. Phys. Fluids 16 (4), 979–990.CrossRefGoogle Scholar
Wu, X., Baltzer, J.R. & Adrian, R.J. 2012 Direct numerical simulation of a 30$R$ long turbulent pipe flow at $R^+ = 685$: large- and very large-scale motions. J. Fluid Mech. 698, 235–281.CrossRefGoogle Scholar
Wu, X. & Moin, P. 2008 A direct numerical simulation study on the mean velocity characteristics in turbulent pipe flow. J. Fluid Mech. 608, 81–112.CrossRefGoogle Scholar
Wu, X. & Moin, P. 2009 Direct numerical simulation of turbulence in a nominally zero-pressure-gradient flat-plate boundary layer. J. Fluid Mech. 630, 5–41.CrossRefGoogle Scholar
Wu, X., Moin, P., Adrian, R.J. & Baltzer, J.R. 2015 Osborne Reynolds pipe flow: direct simulation from laminar through gradual transition to fully developed turbulence. Proc. Natl Acad. Sci. 112 (26), 7920–7924.CrossRefGoogle ScholarPubMed
Xia, Z.-H., Shi, Y.-P. & Chen, S.-Y. 2016 Direct numerical simulation of turbulent channel flow with spanwise rotation. J. Fluid Mech. 788, 42–56.CrossRefGoogle Scholar
Yang, Y.-T., Su, W.-D. & Wu, J.-Z. 2010 Helical-wave decomposition and applications to channel turbulence with streamwise rotation. J. Fluid Mech. 662, 91–122.CrossRefGoogle Scholar
Yang, Z., Deng, B.-D., Wang, B.-C. & Shen, L. 2018 Letter: the effects of streamwise system rotation on pressure fluctuations in a turbulent channel flow. Phys. Fluids 30, 091701.CrossRefGoogle Scholar
Yang, Z. & Wang, B.-C. 2018 Capturing Taylor–Görtler vortices in a streamwise-rotating channel at very high rotation numbers. J. Fluid Mech. 838, 658–689.CrossRefGoogle Scholar
Yoshimatsu, K., Midorikawa, M. & Kaneda, Y. 2011 Columnar eddy formation in freely decaying homogeneous rotating turbulence. J. Fluid Mech. 677, 154–178.CrossRefGoogle Scholar
Yu, C., Hu, R., Yan, Z. & Li, X. 2022 Helicity distributions and transfer in turbulent channel flows with streamwise rotation. J. Fluid Mech. 940, A18.CrossRefGoogle Scholar
Zhang, Z.-P. & Wang, B.-C. 2019 Direct numerical simulation of turbulent flow in a circular pipe subjected to radial system rotation. Flow Turbul. Combust. 103 (4), 1057–1079.CrossRefGoogle Scholar
Zhou, J., Adrian, R.J., Balachandar, S. & Kendall, T.M. 1999 Mechanisms for generating coherent packets of hairpin vortices in channel flow. J. Fluid Mech. 387, 353–396.CrossRefGoogle Scholar