Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Coleman, G. N.
Garbaruk, A.
and
Spalart, P. R.
2015.
Direct Numerical Simulation, Theories and Modelling of Wall Turbulence with a Range of Pressure Gradients.
Flow, Turbulence and Combustion,
Vol. 95,
Issue. 2-3,
p.
261.
Phillips, W. R. C.
2015.
Drift and pseudomomentum in bounded turbulent shear flows.
Physical Review E,
Vol. 92,
Issue. 4,
Seo, J.
García-Mayoral, R.
and
Mani, A.
2015.
Pressure fluctuations and interfacial robustness in turbulent flows over superhydrophobic surfaces.
Journal of Fluid Mechanics,
Vol. 783,
Issue. ,
p.
448.
Alizard, Frédéric
2015.
Linear stability of optimal streaks in the log-layer of turbulent channel flows.
Physics of Fluids,
Vol. 27,
Issue. 10,
de Silva, C. M.
Marusic, I.
Woodcock, J. D.
and
Meneveau, C.
2015.
Scaling of second- and higher-order structure functions in turbulent boundary layers.
Journal of Fluid Mechanics,
Vol. 769,
Issue. ,
p.
654.
Vinuesa, R.
Hites, M. H.
Wark, C. E.
and
Nagib, H. M.
2015.
Documentation of the role of large-scale structures in the bursting process in turbulent boundary layers.
Physics of Fluids,
Vol. 27,
Issue. 10,
de Kat, Roeland
and
Ganapathisubramani, Bharathram
2015.
Frequency–wavenumber mapping in turbulent shear flows.
Journal of Fluid Mechanics,
Vol. 783,
Issue. ,
p.
166.
Angeli, D
Stalio, E
Corticelli, M A
and
Barozzi, G S
2015.
A fast algorithm for Direct Numerical Simulation of natural convection flows in arbitrarily-shaped periodic domains.
Journal of Physics: Conference Series,
Vol. 655,
Issue. ,
p.
012054.
Klewicki, J.
and
Oberlack, M.
2015.
Finite Reynolds number properties of a turbulent channel flow similarity solution.
Physics of Fluids,
Vol. 27,
Issue. 9,
Ahn, Junsun
Lee, Jae Hwa
Lee, Jin
Kang, Ji-hoon
and
Sung, Hyung Jin
2015.
Direct numerical simulation of a 30R long turbulent pipe flow at Re
τ = 3008.
Physics of Fluids,
Vol. 27,
Issue. 6,
Marusic, I.
Chauhan, K. A.
Kulandaivelu, V.
and
Hutchins, N.
2015.
Evolution of zero-pressure-gradient boundary layers from different tripping conditions.
Journal of Fluid Mechanics,
Vol. 783,
Issue. ,
p.
379.
Katul, Gabriel G.
Banerjee, Tirtha
Cava, Daniela
Germano, Massimo
and
Porporato, Amilcare
2016.
Generalized logarithmic scaling for high-order moments of the longitudinal velocity component explained by the random sweeping decorrelation hypothesis.
Physics of Fluids,
Vol. 28,
Issue. 9,
Alfonsi, Giancarlo
Ciliberti, Stefania
Mancini, Marco
and
Primavera, Leonardo
2016.
Direct Numerical Simulation of Turbulent Channel Flow on High-Performance GPU Computing System.
Computation,
Vol. 4,
Issue. 1,
p.
13.
Chen, Xi
Hussain, Fazle
and
She, Zhen-Su
2016.
Bulk flow scaling for turbulent channel and pipe flows.
EPL (Europhysics Letters),
Vol. 115,
Issue. 3,
p.
34001.
Mizuno, Yoshinori
2016.
Spectra of energy transport in turbulent channel flows for moderate Reynolds numbers.
Journal of Fluid Mechanics,
Vol. 805,
Issue. ,
p.
171.
Ostilla-Mónico, Rodolfo
Verzicco, Roberto
Grossmann, Siegfried
and
Lohse, Detlef
2016.
The near-wall region of highly turbulent Taylor–Couette flow.
Journal of Fluid Mechanics,
Vol. 788,
Issue. ,
p.
95.
Hwang, Jinyul
Lee, Jin
and
Sung, Hyung Jin
2016.
Influence of large-scale accelerating motions on turbulent pipe and channel flows.
Journal of Fluid Mechanics,
Vol. 804,
Issue. ,
p.
420.
Pirozzoli, Sergio
Bernardini, Matteo
and
Orlandi, Paolo
2016.
Passive scalars in turbulent channel flow at high Reynolds number.
Journal of Fluid Mechanics,
Vol. 788,
Issue. ,
p.
614.
Gerolymos, G. A.
and
Vallet, I.
2016.
The dissipation tensor in wall turbulence.
Journal of Fluid Mechanics,
Vol. 807,
Issue. ,
p.
386.
Örlü, Ramis
Segalini, Antonio
Klewicki, Joseph
and
Alfredsson, P. Henrik
2016.
High-order generalisation of the diagnostic scaling for turbulent boundary layers.
Journal of Turbulence,
Vol. 17,
Issue. 7,
p.
664.
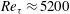 $\mathit{Re}_{{\it\tau}}\approx 5200$
$\mathit{Re}_{{\it\tau}}\approx 5200$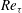 $\mathit{Re}_{{\it\tau}}$) of 5186 has been performed, and the flow exhibits a number of the characteristics of high-Reynolds-number wall-bounded turbulent flows. For example, a region where the mean velocity has a logarithmic variation is observed, with von Kármán constant
$\mathit{Re}_{{\it\tau}}$) of 5186 has been performed, and the flow exhibits a number of the characteristics of high-Reynolds-number wall-bounded turbulent flows. For example, a region where the mean velocity has a logarithmic variation is observed, with von Kármán constant 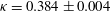 ${\it\kappa}=0.384\pm 0.004$. There is also a logarithmic dependence of the variance of the spanwise velocity component, though not the streamwise component. A distinct separation of scales exists between the large outer-layer structures and small inner-layer structures. At intermediate distances from the wall, the one-dimensional spectrum of the streamwise velocity fluctuation in both the streamwise and spanwise directions exhibits
${\it\kappa}=0.384\pm 0.004$. There is also a logarithmic dependence of the variance of the spanwise velocity component, though not the streamwise component. A distinct separation of scales exists between the large outer-layer structures and small inner-layer structures. At intermediate distances from the wall, the one-dimensional spectrum of the streamwise velocity fluctuation in both the streamwise and spanwise directions exhibits 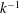 $k^{-1}$ dependence over a short range in wavenumber
$k^{-1}$ dependence over a short range in wavenumber  $(k)$. Further, consistent with previous experimental observations, when these spectra are multiplied by
$(k)$. Further, consistent with previous experimental observations, when these spectra are multiplied by 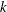 $k$ (premultiplied spectra), they have a bimodal structure with local peaks located at wavenumbers on either side of the
$k$ (premultiplied spectra), they have a bimodal structure with local peaks located at wavenumbers on either side of the 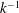 $k^{-1}$ range.
$k^{-1}$ range.


