Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-27T11:19:37.992Z Has data issue: false hasContentIssue false
  • English
  • Français

Direct numerical simulation of a turbulent core-annular flow with water-lubricated high viscosity oil in a vertical pipe

Published online by Cambridge University Press:  20 June 2018

Kiyoung Kim  and
Haecheon Choi Open the ORCID record for Haecheon Choi [Opens in a new window]
Kiyoung Kim
Affiliation:
Department of Mechanical & Aerospace Engineering, Seoul National University, Seoul 08826, Korea
Haecheon Choi*
Affiliation:
Department of Mechanical & Aerospace Engineering, Seoul National University, Seoul 08826, Korea Institute of Advanced Machines and Design, Seoul National University, Seoul 08826, Korea
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The characteristics of a turbulent core-annular flow with water-lubricated high viscosity oil in a vertical pipe are investigated using direct numerical simulation, in conjunction with a level-set method to track the phase interface between oil and water. At a given mean wall friction (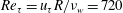$Re_{\unicode[STIX]{x1D70F}}=u_{\unicode[STIX]{x1D70F}}R/\unicode[STIX]{x1D708}_{w}=720$, where 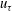$u_{\unicode[STIX]{x1D70F}}$ is the friction velocity, $R$ is the pipe radius and 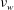$\unicode[STIX]{x1D708}_{w}$ is the kinematic viscosity of water), the total volume flow rate of a core-annular flow is similar to that of a turbulent single-phase pipe flow of water, indicating that water lubrication is an effective tool to transport high viscosity oil in a pipe. The high viscosity oil flow in the core region is almost a plug flow due to its high viscosity, and the water flow in the annular region is turbulent except for the case of large oil volume fraction (e.g. 0.91 in the present study). With decreasing oil volume fraction, the mean velocity profile in the annulus becomes more like that of turbulent pipe flow, but the streamwise evolution of vortical structures is obstructed by the phase interface wave. In a reference frame moving with the core velocity, water is observed to be trapped inside the wave valley in the annulus, and only a small amount of water runs through the wave crest. The phase interface of the core-annular flow consists of different streamwise and azimuthal wavenumber components for different oil holdups. The azimuthal wavenumber spectra of the phase interface amplitude have largest power at the smallest wavenumber whose corresponding wavelength is the pipe circumference, while the streamwise wavenumber having the largest power decreases with decreasing oil volume fraction. The overall convection velocity of the phase interface is slightly lower than the core velocity. Finally, we suggest a predictive oil holdup model by defining the displacement thickness in the annulus and considering the boundary layer characteristics of water flow. This model predicts the variation of the oil holdup with the superficial velocity ratio very well.

JFM classification

Multiphase and Particle-laden Flows: Core-annular flow Flow Control: Drag reduction Turbulent Flows: Turbulent Flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 849 , 25 August 2018 , pp. 419 - 447
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arney, M. S., Bai, R., Guevara, E., Joseph, D. D. & Liu, K. 1993 Friction factor and holdup studies for lubricated pipelining – 1. Experiments and correlations. Intl J. Multiphase Flow 19, 1061–1076.Google Scholar
Arney, M. S., Ribeiro, G. S., Guevara, E., Bai, R. & Joseph, D. D. 1996 Cement-lined pipes for water lubricated transport of heavy oil. Intl J. Multiphase Flow 22, 207–221.Google Scholar
Bai, R.1995 Traveling waves in a high viscosity ratio and axisymmetric core annular flow. PhD thesis, University of Minnesota.Google Scholar
Bai, R., Chen, K. & Joseph, D. D. 1992 Lubricated pipelining: stability of core-annular flow. Part 5. Experiments and comparison with theory. J. Fluid Mech. 240, 97–132.Google Scholar
Bai, R., Kelkar, K. & Joseph, D. D. 1996 Direct simulation of interfacial waves in a high-viscosity-ratio and axisymmetric core-annular flow. J. Fluid Mech. 327, 1–34.Google Scholar
Bannwart, A. C. 2001 Modeling aspects of oil–water core-annular flows. J. Petrol. Sci. Engng 32, 127–143.Google Scholar
Bannwart, A. C., Rodriguez, O. M. H., De Carvalho, C. H. M., Wang, I. S. & Vara, R. M. O. 2004 Flow patterns in heavy crude oil–water flow. J. Energy Resour. Technol. 126, 184–189.Google Scholar
Brackbill, J. U., Kothe, D. B. & Zemach, C. 1992 A continuum method for modeling surface tension. J. Comput. Phys. 100, 335–354.Google Scholar
Brauner, N. 1991 Two-phase liquid-liquid annular flow. Intl J. Multiphase Flow 17, 59–76.Google Scholar
Choi, H. & Moin, P. 1990 On the space–time characteristics of wall-pressure fluctuations. Phys. Fluids 2, 1450–1460.Google Scholar
Choi, H. & Moin, P. 1994 Effects of the computational time step on numerical solutions of turbulent flow. J. Comput. Phys. 113, 1–4.Google Scholar
Choi, H., Moin, P. & Kim, J.1992 Turbulent drag reduction: studies of feedback control and flow over riblets. Rep. TF-55. Department of Mechanical Engineering, Stanford University, Stanford, CA.Google Scholar
Dusseault, M. B.2001 Comparing Venezuelan and Canadian heavy oil and tar sands. In Paper 2001-061 in the Petroleum Society’s Canadian International Petroleum Conference, pp. 1–20. Calgary, AB, Canada.Google Scholar
Eggels, J. G. M., Unger, F., Weiss, M. H., Westerweel, J., Adrian, R. J., Friedrich, R. & Nieuwstadt, F. T. M. 1994 Fully developed turbulent pipe flow: a comparison between direct numerical simulation and experiment. J. Fluid Mech. 268, 175–209.Google Scholar
Feng, J., Huang, P. Y. & Joseph, D. D. 1995 Dynamic simulation of the motion of capsules in pipelines. J. Fluid Mech. 286, 201–227.Google Scholar
Francois, M. M., Cummins, S. J., Dendy, E. D., Kothe, D. B., Sicilian, J. M. & Williams, M. W. 2006 A balance-force algorithm for continuous and sharp interfacial surface tension models within a volume tracking framework. J. Comput. Phys. 213, 141–173.Google Scholar
Ghosh, S., Das, G. & Das, P. K. 2010 Simulation of core annular downflow through CFD – a comprehensive study. Chem. Engng Process. 49, 1222–1228.Google Scholar
Ghosh, S., Mandal, T. K., Das, G. & Das, P. K. 2009 Review of oil water core annular flow. Renew. Sust. Energy Rev. 13, 1957–1965.Google Scholar
Gottlieb, S. & Shu, C.-W. 1998 Total variation diminishing Runge–Kutta schemes. Math. Comput. 67, 73–85.Google Scholar
Herrmann, M. 2008 A balanced force refined level set grid method for two-phase flows on unstructured flow solver grids. J. Comput. Phys. 227, 2674–2706.Google Scholar
Hu, H. H. & Joseph, D. D. 1989 Lubricated pipelining: stability of core-annular flow. Part 2. J. Fluid Mech. 205, 359–396.Google Scholar
Huang, A., Christodoulou, C. & Joseph, D. D. 1994 Friction factor and holdup studies for lubricated pipelining – 2. Laminar and k–𝜀 models of eccentric core flow. Intl J. Multiphase Flow 20, 481–491.Google Scholar
Ingen Housz, E. M. R. M., Ooms, G., Henkes, R. A. W. M., Pourquie, M. J. B. M., Kidess, A. & Radhakrishnan, R. 2017 A comparison between numerical predictions and experimental results for core-annular flow with a turbulent annulus. Intl J. Multiphase Flow 95, 271–282.Google Scholar
Irons, B. M. & Tuck, R. C. 1969 A version of the Aitken accelerator for computer iteration. Intl J. Numer. Meth. Engng 1, 275–277.Google Scholar
Ismail, A. S. I., Ismail, I., Zoveidavianpoor, M., Mohsin, R., Piroozian, A., Misnan, M. S. & Sariman, M. Z. 2015 Review of oil–water through pipes. Flow Meas. Instrum. 45, 357–374.Google Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.Google Scholar
Jiang, G.-S. & Peng, D. 2000 Weighted ENO schemes for Hamilton–Jacobi equations. SIAM J. Sci. Comput. 21, 2126–2143.Google Scholar
Joseph, D. D., Renardy, M. & Renardy, Y. 1984 Instability of the flow of two immiscible liquids with different viscosities in a pipe. J. Fluid Mech. 141, 309–317.Google Scholar
Kang, M., Fedkiw, R. P. & Liu, X.-D. 2000 A boundary condition capturing method for multiphase incompressible flow. J. Sci. Comput. 15, 323–360.Google Scholar
Kang, S., Moin, P. & Iaccarino, G.2008 An improved immersed boundary method for computation of turbulent flows with heat transfer. Rep. TF-108. Department of Mechanical Engineering, Stanford University, Stanford, CA.Google Scholar
Kim, D. & Moin, P.2011 Direct numerical simulation of two-phase flows with application to air layer drag reduction. Rep. TF-125. Department of Mechanical Engineering, Stanford University, Stanford, CA.Google Scholar
Ko, T., Choi, H. G., Bai, R. & Joseph, D. D. 2002 Finite element method simulation of turbulent wavy core-annular flows using a k-𝜔 turbulence model method. Intl J. Multiphase Flow 28, 1205–1222.Google Scholar
Kouris, C. & Tsamopoulos, J. 2001 Dynamics of axisymmetric core-annular flow in a straight tube. 1. The more viscous fluid in the core, bamboo waves. Phys. Fluids 13, 1352623.Google Scholar
Kouris, C. & Tsamopoulos, J. 2002 Core-annular flow in a periodically constricted circular tube. Part 2. Nonlinear dynamics. J. Fluid Mech. 470, 181–222.Google Scholar
Li, J. & Renardy, Y. 1999 Direct simulation of unsteady axisymmetric core-annular flow with high viscosity ratio. J. Fluid Mech. 391, 123–149.Google Scholar
Oliemans, R. V. A.1986 The lubricating-film model for core-annular flow. PhD thesis, Delft University of Technology.Google Scholar
Ooms, G., Pourquie, M. J. B. M. & Beerens, J. C. 2013 On the levitation force in horizontal core-annular flow with a large viscosity ratio and small density ratio. Phys. Fluids 25, 032102.Google Scholar
Ooms, G., Segal, A., van der Wees, A. J., Meerhoff, R. & Oliemans, R. V. A. 1984 A theoretical model for core-annular flow of a very viscous oil core and a water annulus through a horizontal pipe. Intl J. Multiphase Flow 10, 41–60.Google Scholar
Orazzo, A., Coppola, G. & De Luca, L. 2014 Disturbance energy growth in core-annular flow. J. Fluid Mech. 747, 44–72.Google Scholar
Peng, D., Merriman, B., Osher, S., Zhao, H. & Kang, M. 1999 A PDE-based fast local level set method. J. Comput. Phys. 155, 410–438.Google Scholar
Polderman, H. G., Velraeds, G. & Knol, W. 1986 Turbulent lubrication flow in an annular channel. Trans. ASME J. Fluids Engng 108, 185–192.Google Scholar
Preziosi, L., Chen, K. & Joseph, D. D. 1989 Lubricated pipelining: stability of core-annular flow. J. Fluid Mech. 201, 323–356.Google Scholar
Raessi, M. & Pitsch, H. 2012 Consistent mass and momentum transport for simulating incompressible interfacial flows with large density ratios using the level set method. Comput. Fluids 63, 70–81.Google Scholar
Rodriguez, O. M. H. & Bannwart, A. C. 2006 Experimental study on interfacial waves in vertical core flow. J. Petrol. Sci. Engng 54, 140–148.Google Scholar
Shi, J., Gourma, M. & Yeung, H. 2017 CFD simulation of horizontal oil–water flow with matched density and medium viscosity ratio in different flow regimes. J. Petrol. Sci. Engng 151, 373–383.Google Scholar
Son, G. 2001 A numerical method for bubble motion with phase change. Numer. Heat Transfer B 39, 509–523.Google Scholar
Sotgia, G., Tartarini, P. & Stalio, E. 2008 Experimental analysis of flow regimes and pressure drop reduction in oil–water mixtures. Intl J. Multiphase Flow 34, 1161–1174.Google Scholar
Spalding, D. B. 1961 A single formula for the ‘law of the wall’. Trans. ASME J. Appl. Mech. 28, 455–458.Google Scholar
Sussman, M., Smereka, P. & Osher, S. 1994 A level set approach for computing solutions to incompressible two-phase flow. J. Comput. Phys. 114, 146–159.Google Scholar
Tripathi, S., Tabor, R. F., Singh, R. & Bhattacharya, A. 2017 Characterization of interfacial waves and pressure drop in horizontal oil–water core-annular flows. Phys. Fluids 29, 082109.Google Scholar
van der Pijl, S. P., Segal, A., Vuik, C. & Wesseling, P. 2005 A mass-conserving level-set method for modelling of multi-phase flows. Intl J. Numer. Meth. Fluids 47, 339–361.Google Scholar
Vanegas Prada, J. W.1999 Estudo experimental do escoamento anular óleo-água (‘core flow’) na elevaçáo de óleos ultraviscosos. PhD thesis, Universidade Estadual de Campinas.Google Scholar
Vanegas Prada, J. W. & Bannwart, A. C. 2001 Modeling of vertical core-annular flows and application to heavy oil production. J. Energy Resour. Technol. 123, 194–199.Google Scholar
Vuong, D. H.2009 Experimental study on high viscosity oil–water flows in horizontal and vertical pipes. PhD thesis, The University of Tulsa.Google Scholar
Wills, J. A. B. 1970 Measurements of the wave-number/phase velocity spectrum of wall pressure beneath a turbulent boundary layer. J. Fluid Mech. 45, 65–90.Google Scholar
Wu, X., Baltzer, J. R. & Adrian, R. J. 2012 Direct numerical simulation of a 30R long turbulent pipe flow at R + = 685: large- and very large-scale motions. J. Fluid Mech. 698, 235–281.Google Scholar
Zhang, Y., Zou, Q. & Greaves, D. 2010 Numerical simulation of free-surface flow using the level-set method with global mass correction. Intl J. Numer. Meth. Fluids 63, 651–680.Google Scholar