Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-25T14:08:13.508Z Has data issue: false hasContentIssue false
  • English
  • Français

A direct comparison of particle-resolved and point-particle methods in decaying turbulence

Published online by Cambridge University Press:  04 July 2018

M. Mehrabadi ,
J. A. K. Horwitz ,
S. Subramaniam Open the ORCID record for S. Subramaniam [Opens in a new window]  and
A. Mani Open the ORCID record for A. Mani [Opens in a new window]
M. Mehrabadi
Affiliation:
University of Illinois at Urbana-Champaign, Department of Aerospace Engineering, Urbana, IL 61820, USA
J. A. K. Horwitz
Affiliation:
Stanford University, Department of Mechanical Engineering, Stanford, CA 94305, USA
S. Subramaniam*
Affiliation:
Iowa State University, Department of Mechanical Engineering, Ames, IA 50010, USA
A. Mani
Affiliation:
Stanford University, Department of Mechanical Engineering, Stanford, CA 94305, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We use particle-resolved direct numerical simulation (PR-DNS) as a model-free physics-based numerical approach to validate particle acceleration modelling in gas-solid suspensions. To isolate the effect of the particle acceleration model, we focus on point-particle direct numerical simulation (PP-DNS) of a collision-free dilute suspension with solid-phase volume fraction $\unicode[STIX]{x1D719}=0.001$ in a decaying isotropic turbulent particle-laden flow. The particle diameter 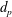$d_{p}$ in the suspension is chosen to be the same as the initial Kolmogorov length scale 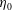$\unicode[STIX]{x1D702}_{0}$ (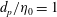$d_{p}/\unicode[STIX]{x1D702}_{0}=1$) in order to overlap with the regime where PP-DNS is valid. We assess the point-particle acceleration model for two different particle Stokes numbers, 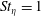$St_{\unicode[STIX]{x1D702}}=1$ and 100. For the high Stokes number case, the Stokes drag model for particle acceleration under-predicts the true particle acceleration. In addition, second moment quantities which play key roles in the physical evolution of the gas–solid suspension are not correctly captured. Considering finite Reynolds number corrections to the acceleration model improves the prediction of the particle acceleration probability density function and second moment statistics of the point-particle model compared with the particle-resolved simulation. We also find that accounting for the undisturbed fluid velocity in the acceleration model can be of greater importance than using the most appropriate acceleration model for a given physical problem.

JFM classification

Turbulent Flows: Isotropic turbulence Complex Fluids: Suspensions
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 850 , 10 September 2018 , pp. 336 - 369
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

‡

Equally contributing first authors.

References

Akiki, G., Jackson, T. L. & Balachandar, S. 2017a Pairwise interaction extended point-particle model for a random array of monodisperse spheres. J. Fluid Mech. 813, 882–928.Google Scholar
Akiki, G., Moore, W. C. & Balachandar, S. 2017b Pairwise-interaction extended point-particle model for particle-laden flows. J. Comput. Phys. 351, 329–357.Google Scholar
Bagchi, P. & Balachandar, S. 2003 Effect of turbulence on the drag and lift of a particle. Phys. Fluids 15 (11), 3496–3513.Google Scholar
Balachandar, S. & Eaton, J. K. 2010 Turbulent dispersed multiphase flow. Annu. Rev. Fluid Mech. 42, 111–133.Google Scholar
Balachandar, S. & Maxey, M. R. 1989 Methods for evaluating fluid velocities in spectral simulations of turbulence. J. Comput. Phys. 83, 96–125.Google Scholar
Basset, A. B. 1888 A Treatise on Hydrodynamics: With Numerous Examples, vol. 2. Cambridge University Press.Google Scholar
Batchelor, G. K. 1967 An Introduction to Fluid Dynamics. Cambridge University Press.Google Scholar
Batchelor, G. K. 1972 Sedimentation in a dilute dispersion of spheres. J. Fluid Mech. 52, 245–268.Google Scholar
Batchelor, G. K. & Green, J. T. 1972 The hydrodynamic interaction of two small freely-moving spheres in a linear flow field. J. Fluid Mech. 56 (2), 375–400.Google Scholar
Boivin, M., Simonin, O. & Squires, K. D. 1998 Direct numerical simulation of turbulence modulation by particles in isotropic turbulence. J. Fluid Mech. 275, 235–263.Google Scholar
Bokkers, G. A., Annaland, M. V. S. & Kuipers, J. A. M. 2004 Mixing and segregation in a bidisperse gas–solid fluidised bed: a numerical and experimental study. Powder Technol. 140, 176–186.Google Scholar
Boussinesq, J. 1885 Sur la résistance qu’oppose un liquide indéfini en repos, sans pesanteur, au mouvement varié d’une sphère solide qu’il mouille sur toute sa surface, quand les vitesses restent bien continues et assez faibles pour que leurs carrés et produits soient négligeables. C. R. Acad. Sci. Paris 100, 935–937.Google Scholar
Burton, T. M. & Eaton, J. K. 2005 Fully resolved simulations of particle-turbulence interaction. J. Fluid Mech. 545, 67–111.Google Scholar
Calzavarini, E., Volk, R., Bourgoin, M., Leveque, E., Pinton, J. F. & Toschi, F. 2009 Acceleration statistics of finite-sized particles in turbulent flow: the role of Faxén forces. J. Fluid Mech. 630, 179–189.Google Scholar
Cate, A. T., Derksen, J. J., Portela, L. M. & van den Akker, H. E. A. 2004 Fully resolved simulations of colliding monodisperse spheres in forced isotropic turbulence. J. Fluid Mech. 519, 233–271.Google Scholar
Chouippe, A. & Uhlmann, M. 2015 Forcing homogeneous turbulence in direct numerical simulation of particulate flow with interface resolution and gravity. Phys. Fluids 27 (12), 123301.Google Scholar
Clift, R., Grace, J. R. & Weber, M. E. 1978 Bubbles, Drops and Particles. Academic.Google Scholar
Coimbra, C. F. M. & Rangel, R. H. 1998 General solution of the particle momentum equation in unsteady Stokes flows. J. Fluid Mech. 370, 53–72.Google Scholar
Corrsin, S. & Lumley, J. 1956 On the equation of motion for a particle in turbulent fluid. Appl. Sci. Res. A 6, 114–116.Google Scholar
Cundall, P. A. & Strack, O. D. L. 1979 A discrete numerical model for granular assemblies. Geotechnique 29, 47–65.Google Scholar
Daitche, A. 2015 On the role of the history force for inertial particles in turbulence. J. Fluid Mech. 782, 567–593.Google Scholar
Drew, D. A. & Passman, S. L. 1998 Theory of Multicomponent Fluids. Springer.Google Scholar
Elghobashi, S. & Truesdell, G. C. 1992 Direct simulation of particle dispersion in a decaying isotropic turbulence. J. Fluid Mech. 242, 655–700.Google Scholar
Elghobashi, S. E. & Truesdell, G. C. 1993 On the two-way interaction between homogeneous turbulence and dispersed solid particles. I: Turbulence modification. Phys. Fluids A 5, 1790–1801.Google Scholar
Fan, R., Marchisio, D. L. & Fox, R. O. 2004 Application of the direct quadrature method of moments to polydisperse gas–solid fluidized beds. Powder Technol. 139 (1), 7–20.Google Scholar
Faxén, V. H. 1922 Der widerstand gegen die bewegung einer starren kugel in einer zahen flussigkeit, die zwischen zwei parallelen ebenen wanden eingeschlossen ist. Ann. Phys. 373 (10), 89–119.Google Scholar
Ferrante, A. & Elghobashi, S. 2003 On the physical mechanisms of two-way coupling in particle-laden isotropic turbulence. Phys. Fluids 15 (2), 315–329.Google Scholar
Frankel, A., Pouransari, H., Coletti, F. & Mani, A. 2016 Settling of heated particles in homogeneous turbulence. J. Fluid Mech. 792, 869–893.Google Scholar
Ganguli, S. & Lele, S. 2017 Importance of variable density and non-Boussinesq effects on the drag of spherical particles. In 70th Annual Meeting of the APS Division of Fluid Dynamics, Denver, CO. American Physical Society.Google Scholar
Gao, H., Li, H. & Wang, L.-P. 2013 Lattice Boltzmann simulation of turbulent flow laden with finite-size particles. Comput. Math. Appl. 65 (2), 194–210.Google Scholar
Garg, R., Tenneti, S., Mohd-Yusof, J. & Subramaniam, S. 2011 Direct numerical simulation of gas–solids flow based on the immersed boundary method. In Computational Gas-Solids Flows and Reacting Systems: Theory, Methods and Practice (ed. Pannala, S., Syamlal, M. & O’Brien, T. J.), pp. 245–276. IGI Global.Google Scholar
Gatignol, R. 1983 The Faxén formulae for a rigid sphere in an unsteady non-uniform stokes flow. Journal de mécanique théorique et appliquée 1, 143–160.Google Scholar
Girifalco, L. A. 2000 Statistical Mechanics of Solids. Oxford University Press.Google Scholar
Goldschmidt, M. J. V., Link, J. M., Mellema, S. & Kuipers, J. A. M. 2003 Digital image analysis measurements of bed expansion and segregation dynamics in dense gas–solid fluidized beds. Powder Technol. 138, 135–159.Google Scholar
Gualtieri, P., Picano, F., Sardina, G. & Casciola, C. M. 2015 Exact regularized point particle method for multiphase flows in the two-way coupling regime. J. Fluid Mech. 773, 520–561.Google Scholar
Homann, H. & Bec, J. 2010 Finite-size effects in the dynamics of neutrally buoyant particles in turbulent flow. J. Fluid Mech. 651, 81–91.Google Scholar
Horwitz, J. & Mani, A. 2015 Simulations of decaying turbulence laden with particles: how are statistics affected by two-way coupling numerical scheme? In 68th Annual Meeting of the APS Division of Fluid Dynamics, Boston, Massachusetts. American Physical Society.Google Scholar
Horwitz, J. A. K. & Mani, A. 2016 Accurate calculation of stokes drag for point-particle tracking in two-way coupled flows. J. Comput. Phys. 318, 85–109.Google Scholar
Horwitz, J. A. K. & Mani, A. 2018 Correction scheme for point-particle models applied to a nonlinear drag law in simulations of particle-fluid interaction. Intl J. Multiphase Flow 101, 74–84.Google Scholar
Horwitz, J. A. K., Rahmani, M., Geraci, G., Banko, A. J. & Mani, A. 2016 Two-way coupling effects in particle-laden turbulence: how particle-tracking scheme affects particle and fluid statistics. In 9th International Conference on Multiphase Flow, Firenze.Google Scholar
Ireland, P. J. & Desjardins, O. 2017 Improving particle drag predictions in euler-lagrange simulations with two-way coupling. J. Comput. Phys. 338, 405–430.Google Scholar
Kidanemariam, A. G., Chan-Braun, C., Doychev, T. & Uhlmann, M. 2013 Direct numerical simulation of horizontal open channel flow with finite-size, heavy particles at low solid volume fraction. New J. Phys. 15 (2), 025031.Google Scholar
Kiger, K. T. & Pan, C. 2000 PIV technique for the simultaneous measurement of dilute two-phase flows. Trans. ASME J. Fluids Engng 122 (4), 811–818.Google Scholar
Kim, J. & Moin, P. 1985 Application of a fractional-step method to incompressible Navier–Stokes equations. J. Comput. Phys. 59, 308–323.Google Scholar
Kim, S. & Karrila, S. J. 2005 Microhydrodynamics: Principles and Selected Applications. Butterworth-Heinemann.Google Scholar
Koch, D. L. 1990 Kinetic theory for a monodisperse gas–solid suspension. Phys. Fluids A 2, 1711–1723.Google Scholar
Lee, S. L. & Durst, F. 1982 On the motion of particles in turbulent duct flows. Intl J. Multiphase Flow 8 (2), 125–146.Google Scholar
Ling, Y., Parmar, M. & Balachandar, S. 2013 A scaling analysis of added-mass and history forces and their coupling in dispersed multiphase flows. Intl J. Multiphase Flow 57, 102–114.Google Scholar
Lovalenti, P. M. & Brady, J. F. 1993 The hydrodynamic force on a rigid particle undergoing arbitrary time-dependent motion at small Reynolds number. J. Fluid Mech. 256, 561–605.Google Scholar
Lucci, F., Ferrante, A. & Elghobashi, S. 2011 Is Stokes number an appropriate indicator for turbulence modulation by particles of Taylor-length-scale size? Phys. Fluids 23 (2), 025101.Google Scholar
Maxey, M. R. & Riley, J. J. 1983 Equation of motion for a small rigid sphere in a uniform flow. Phys. Fluids 26 (4), 883–889.Google Scholar
Mehrabadi, M., Murphy, E. & Subramaniam, S. 2016a Development of a gas–solid drag law for clustered particles using particle-resolved direct numerical simulation. Chem. Engng Sci. 152, 199–212.Google Scholar
Mehrabadi, M. & Subramaniam, S. 2017 Mechanism of kinetic energy transfer in homogeneous bidisperse gas–solid flow and its implications for segregation. Phys. Fluids 29, 020714.Google Scholar
Mehrabadi, M., Tenneti, S., Garg, R. & Subramaniam, S. 2015 Pseudo-turbulent gas-phase velocity fluctuations in homogeneous gas–solid flow: fixed particle assemblies and freely evolving suspensions. J. Fluid Mech. 770, 210–246.Google Scholar
Mehrabadi, M., Tenneti, S. & Subramaniam, S. 2016b Importance of the fluid-particle drag model in predicting segregation in bidisperse gas–solid flow. Intl J. Multiphase Flow 86, 99–114.Google Scholar
Mohd-Yusof, J.1996 Interaction of massive particles with turbulence. PhD thesis, Cornell University.Google Scholar
Naso, A. & Prosperetti, A. 2010 The interaction between a solid particle and a turbulent flow. New J. Phys. 12 (3), 033040.Google Scholar
Oakley, T. R., Loth, E. & Adrian, R. J. 1997 A two-phase cinematic PIV method for bubbly flows. Trans. ASME J. Fluids Engng 119 (3), 707–712.Google Scholar
Olivieri, S., Picano, F., Sardina, G., Iudicone, D. & Brandt, L. 2014 The effect of the basset history force on particle clustering in homogeneous and isotropic turbulence. Phys. Fluids 26, 041704.Google Scholar
Ozel, A., de Mottaa, J. C. B., Abbas, M., Fedea, P., Masbernat, O., Vincent, S., Estivalezes, J. L. & Simonin, O. 2017 Particle resolved direct numerical simulation of a liquid–solid fluidized bed: comparison with experimental data. Intl J. Multiphase Flow 89, 228–240.Google Scholar
Pai, M. G. & Subramaniam, S. 2009 A comprehensive probability density function formalism for multiphase flows. J. Fluid Mech. 628, 181–228.Google Scholar
Parmar, M., Haselbacher, A. & Balachandar, S. 2012 Equation of motion for a sphere in non-uniform compressible flows. J. Fluid Mech. 699, 352–375.Google Scholar
Pope, S. B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Pouransari, H., Mortazavi, M. & Mani, A. 2015 Parallel variable-density particle-laden turbulence simulation. Annu. Res. Briefs 2015, 43–54.Google Scholar
Reade, W. C. & Collins, L. R. 2000 Effect of preferential concentration on turbulent collision rates. Phys. Fluids 12 (10), 2530–2540.Google Scholar
Riley, J. J. & Patterson, G. S. 1974 Diffusion experiments with numerically integrated isotropic turbulence. Phys. Fluids 17 (2), 292–297.Google Scholar
Rogallo, R. S.1981 Numerical experiments in homogeneous turbulence. NASA Tech. Rep. 81835.Google Scholar
Rogers, C. B. & Eaton, J. K. 1991 The effect of small particles on fluid turbulence in a flat plate, turbulent boundary layer in air. Phys. Fluids 3 (5), 928–937.Google Scholar
Sato, Y., Hishida, K. & Maeda, M. 1996 Effect of dispersed phase on modification of turbulent flow in a wall jet. Trans. ASME J. Fluids Engng 118 (2), 307–315.Google Scholar
Schneiders, L., Meinke, M. & Schroder, W. 2016 On the accuracy of Lagrangian point-mass models for heavy nonspherical particles in isotropic turbulence. Fuel 201, 2–14.Google Scholar
Squires, K. D. & Eaton, J. K. 1990 Particle response and turbulence modification in isotropic turbulence. Phys. Fluids A 2 (7), 292–297.Google Scholar
Squires, K. D. & Eaton, J. K. 1991a Measurements of particle dispersion obtained from direct numerical simulations of isotropic turbulence. J. Fluid Mech. 226, 1–35.Google Scholar
Squires, K. D. & Eaton, J. K. 1991b Preferential concentration of particles by turbulence. Phys. Fluids A 3, 1169–1178.Google Scholar
Stimson, M. & Jeffrey, G. G. 1926 The motion of two spheres in a viscous fluid. Proc. R. Soc. Lond. A 111 (757), 110–116.Google Scholar
Stokes, G. G. 1850 On the effect of the inertial friction of fluids on the motion of pendulums. Trans. Camb. Phil. Soc. 9, 1–86.Google Scholar
Subramaniam, S., Mehrabadi, M., Horwitz, J. & Mani, A. 2014 Developing improved lagrangian point particle models of gas–solid flow from particle-resolved direct numerical simulation. In Studying Turbulence Using Numerical Simulation Databases–XV, Proceedings of the CTR 2014 Summer Program, pp. 5–14. Center for Turbulence Research, Stanford University.Google Scholar
Sun, B., Tenneti, S. & Subramaniam, S. 2015 Modeling average gas–solid heat transfer using particle-resolved direct numerical simulation. Intl J. Heat Mass Transfer 86, 898–913.Google Scholar
Sun, B., Tenneti, S., Subramaniam, S. & Koch, D. L. 2016 Pseudo-turbulent heat flux and average gas-phase conduction during gas–solid heat transfer: flow past random fixed particle assemblies. J. Fluid Mech. 798, 299–349.Google Scholar
Sundaram, S. & Collins, L. R. 1996 Numerical considerations in simulating a turbulent suspension of finite-volume particles. J. Comput. Phys. 124, 337–350.Google Scholar
Sundaram, S. & Collins, L. R. 1997 Collision statistics in an isotropic particle-laden turbulent suspension. Part 1. Direct numerical simulations. J. Fluid Mech. 335, 75–109.Google Scholar
Sundaram, S. & Collins, L. R. 1999 A numerical study of the modulation of isotropic turbulence by suspended particles. J. Fluid Mech. 379, 105–143.Google Scholar
Taneda, S. 1956 Experimental investigation of the wake behind a sphere at low Reynolds numbers. J. Phys. Soc. Japan 11 (10), 1104–1108.Google Scholar
Tenneti, S., Garg, R., Hrenya, C. M., Fox, R. O. & Subramaniam, S. 2010 Direct numerical simulation of gas–solid suspensions at moderate Reynolds number: quantifying the coupling between hydrodynamic forces and particle velocity fluctuations. Powder Technol. 203 (1), 57–69.Google Scholar
Tenneti, S., Garg, R. & Subramaniam, S. 2011 Drag law for monodisperse gas–solid systems using particle-resolved direct numerical simulation of flow past fixed assemblies of spheres. Intl J. Multiphase Flow 37, 1072–1092.Google Scholar
Tenneti, S., Mehrabadi, M. & Subramaniam, S. 2016 Stochastic Lagrangian model for hydrodynamic acceleration of inertial particles in gas–solid suspensions. J. Fluid Mech. 788, 695–729.Google Scholar
Tenneti, S. & Subramaniam, S. 2014 Particle-resolved direct numerical simulation for gas–solid flow model development. Annu. Rev. Fluid Mech. 46 (1), 199–230.Google Scholar
Tenneti, S., Sun, B., Garg, R. & Subramaniam, S. 2013 Role of fluid heating in dense gas–solid flow as revealed by particle-resolved direct numerical simulation. Intl J. Heat Mass Transfer 58 (1), 471–479.Google Scholar
Truesdell, G. C. & Elghobashi, S. 1994 On the two-way interaction between homogeneous turbulence and dispersed solid particles. II. Particle dispersion. Phys. Fluids 6 (3), 1405–1407.Google Scholar
Uhlmann, M. 2008 Interface-resolved direct numerical simulation of vertical particulate channel flow in the turbulent regime. Phys. Fluids 20 (5), 053305.Google Scholar
Wang, L.-P., Ayala, O., Gao, H., Andersen, C. & Mathews, K. L. 2014 Study of forced turbulence and its modulation by finite-size solid particles using the lattice Boltzmann approach. Comput. Maths Applics. 67 (2), 363–380.Google Scholar
Wang, L.-P. & Maxey, M. R. 1993 Settling velocity and concentration distribution of heavy particles in homogeneous isotropic turbulence. Phys. Fluids 256, 27–68.Google Scholar
Xu, Y. & Subramaniam, S. 2007 Consistent modeling of interphase turbulent kinetic energy transfer in particle-laden turbulent flows. Phys. Fluids 19 (8), 085101.Google Scholar
Xu, Y. & Subramaniam, S. 2010 Effect of particle clusters on carrier flow turbulence: A direct numerical simulation study. Flow Turbul. Combust. 85, 735–761.Google Scholar
Yeung, P. K. & Pope, S. B. 1988 An algorithm for tracking fluid particles in numerical simulations of homogeneous turbulence. J. Comput. Phys. 79 (2), 373–416.Google Scholar
Zhang, Z. & Prosperetti, A. 2005 A second–order method for three–dimensional particle simulation. J. Comput. Phys. 210 (1), 292–324.Google Scholar
Zick, A. A. & Homsy, G. M. 1982 Stokes flow through periodic arrays of spheres. J. Fluid Mech. 115, 13–26.Google Scholar