Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-26T04:51:00.189Z Has data issue: false hasContentIssue false
  • English
  • Français

Destratification of thermally stratified turbulent open-channel flow by surface cooling

Published online by Cambridge University Press:  28 July 2020

Michael P. Kirkpatrick Open the ORCID record for Michael P. Kirkpatrick [Opens in a new window] ,
N. Williamson Open the ORCID record for N. Williamson [Opens in a new window] ,
S. W. Armfield  and
V. Zecevic
Michael P. Kirkpatrick*
Affiliation:
School of Aerospace, Mechanical and Mechatronic Engineering, The University of Sydney, Sydney, NSW2006, Australia
N. Williamson
Affiliation:
School of Aerospace, Mechanical and Mechatronic Engineering, The University of Sydney, Sydney, NSW2006, Australia
S. W. Armfield
Affiliation:
School of Aerospace, Mechanical and Mechatronic Engineering, The University of Sydney, Sydney, NSW2006, Australia
V. Zecevic
Affiliation:
School of Aerospace, Mechanical and Mechatronic Engineering, The University of Sydney, Sydney, NSW2006, Australia
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

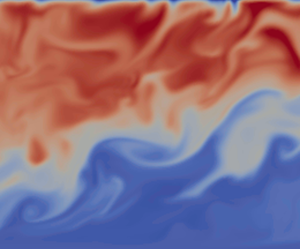
Destratification of thermally stratified open-channel flow by surface cooling is investigated using direct numerical simulation. The initial states are the equilibrium states resulting from radiative heating. Using these states as initial conditions, a series of direct numerical simulations was run with radiative heating removed and a constant, uniform cooling flux applied at the upper surface. The flow evolves until the initial stable stratification is broken down and replaced by unstable stratification driven by surface cooling. The destratification process is described with reference to the evolution of the internal structure of the turbulent flow field. Based on these observations, we conclude that the dominant time scales in the flow from the perspective of destratification are the time scales associated with shear ${t}_{\tau }$, convection ${t}_*$ and stable density stratification ${t}_N$. Scaling arguments are then used to derive a scaling relationship for destratification rate as a function of a friction Richardson number $Ri_{\tau } = ( {t}_{\tau }/ {t}_N)^2$ and a convection Richardson number $Ri_* = ( {t}_*/ {t}_N)^2$. The relationship takes the form ${\mathcal {D}}_N = C_1Ri_{\tau }^{-1} + C_2Ri_*^{-1}$, where ${\mathcal {D}}_N$ is the destratification rate non-dimensionalised with respect to $ {t}_N$ and $C_1$ and $C_2$ are model coefficients. The relationship is compared with simulation results and is shown to accurately predict the destratification rate in the simulations across a range of parameters. This relationship is then integrated to give a formula for the time taken for the flow to destratify.

JFM classification

Geophysical and Geological Flows: Stratified flows Turbulent Flows: Turbulent convection Geophysical and Geological Flows: River dynamics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 899 , 25 September 2020 , A29
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Angevine, W. M., Grimsdell, A. W., Hartten, L. M. & Delany, A. C. 1998 The Flatland boundary layer experiments. Bull. Am. Meteorol. Soc. 79 (3), 419–432.2.0.CO;2>CrossRefGoogle Scholar
Armenio, V. & Sarkar, S. 2002 An investigation of stably stratified turbulent channel flow using large-eddy simulation. J. Fluid Mech. 459, 1–42.CrossRefGoogle Scholar
Arya, S. P. S. 1975 Buoyancy effects in a horizontal flat-plate boundary layer. J. Fluid Mech. 68 (2), 321–343.CrossRefGoogle Scholar
Basu, S. & Porté-Agel, F. 2006 Large-eddy simulation of stably stratified atmospheric boundary layer turbulence: a scale-dependent dynamic modeling approach. J. Atmos. Sci. 63 (8), 2074–2091.CrossRefGoogle Scholar
Batchelor, G. K. 1959 Small-scale variation of convected quantities like temperature in turbulent fluid Part 1. General discussion and the case of small conductivity. J. Fluid Mech. 5 (1), 113–133.CrossRefGoogle Scholar
Betts, A. K. & Ball, J. H. 1994 Budget analysis of FIFE 1987 sonde data. J. Geophys. Res. 99 (D2), 3655–3666.CrossRefGoogle Scholar
Bjerklie, D. M., Dingman, S. L. & Bolster, C. H. 2005 Comparison of constitutive flow resistance equations based on the Manning and Chezy equations applied to natural rivers. Water Resour. Res. 41 (11).CrossRefGoogle Scholar
Bormans, M., Maier, H., Burch, M. & Baker, P. 1997 Temperature stratification in the lower River Murray, Australia: implication for cyanobacterial bloom development. Mar. Freshwat. Res. 48 (7), 647–654.CrossRefGoogle Scholar
Bormans, M. & Webster, I. T. 1997 A mixing criterion for turbid rivers. Environ. Model. Softw. 12 (4), 329–333.CrossRefGoogle Scholar
Bormans, M. & Webster, I. T. 1998 Dynamics of temperature stratification in lowland rivers. J.Hydraul. Engng ASCE 124 (10), 1059–1063.CrossRefGoogle Scholar
Bouffard, D. & Wüest, A. 2019 Convection in lakes. Annu. Rev. Fluid Mech. 51 (1), 189–215.CrossRefGoogle Scholar
Bouffard, D., Zdorovennova, G., Bogdanov, S., Efremova, T., Lavanchy, S., Palshin, N., Terzhevik, A., Vinnå, L. R., Volkov, S., Wüest, A., et al. 2019 Under-ice convection dynamics in a boreal lake. Inland Waters 9 (2), 142–161.CrossRefGoogle Scholar
Bretherton, C. S., Macvean, M. K., Bechtold, P., Chlond, A., Cotton, W. R., Cuxart, J., Cuijpers, H., Mhairoutdinov, M., Kosovic, B., Lewellen, D., et al. 1999 An intercomparison of radiatively driven entrainment and turbulence in a smoke cloud, as simulated by different numerical models. Q. J. R. Meteorol. Soc. 125 (554), 391–423.CrossRefGoogle Scholar
Brethouwer, G., Billant, P., Lindborg, E & Chomaz, J.-M. 2007 Scaling analysis and simulation of strongly stratified turbulent flows. J. Fluid Mech. 585, 343–368.CrossRefGoogle Scholar
Chung, D. & Matheou, G. 2012 Direct numerical simulation of stationary homogeneous stratified sheared turbulence. J. Fluid Mech. 696, 434–467.CrossRefGoogle Scholar
D'Asaro, E. A., Winters, K. B & Lien, R.-C. 2002 Lagrangian analysis of a convective mixed layer. J. Geophys. Res. 107 (C5), 8-1–8-17.CrossRefGoogle Scholar
Deardorff, J. W., Willis, G. E. & Stockton, B. H. 1980 Laboratory studies of the entrainment zone of a convectively mixed layer. J. Fluid Mech. 100 (1), 41–64.CrossRefGoogle Scholar
Deardorff, W. 1970 Convective velocity and temperature scales for the unstable planetary boundary layer and for Rayleigh convection. J. Atmos. Sci. 27, 1211–1213.2.0.CO;2>CrossRefGoogle Scholar
Dyer, A. J. 1974 A review of flux-profile relationships. Boundary Layer Meteorol. 7 (3), 363–372.CrossRefGoogle Scholar
Fedorovich, E., Conzemius, R. & Mironov, D. 2004 Convective entrainment into a shear-free, linearly stratified atmosphere: bulk models re-evaluated through large eddy simulations. J. Atmos. Sci. 61, 281–295.2.0.CO;2>CrossRefGoogle Scholar
Fernando, H. J. S. 1991 Turbulent mixing in stratified fluids. Annu. Rev. Fluid Mech. 23 (1), 455–493.CrossRefGoogle Scholar
Fernando, H. J. S. & Little, L. J. 1990 Molecular-diffusive effects in penetrative convection. Phys. Fluids A 2 (9), 1592–1596.CrossRefGoogle Scholar
Garcia-Villalba, M. & del Alamo, J. C. 2011 Turbulence modification by stable stratification in channel flow. Phys. Fluids 23 (4), 045104.CrossRefGoogle Scholar
Hägeli, P., Steyn, D. G. & Strawbridge, K. B. 2000 Spatial and temporal variability of mixed-layer depth and entrainment zone thickness. Boundary Layer Meteorol. 97 (1), 47–71.CrossRefGoogle Scholar
Ihle, C. F. & Niño, Y. 2012 The onset of natural convection in lakes and reservoirs due to night time cooling. Environ. Fluid Mech. 12 (2), 133–144.CrossRefGoogle Scholar
Ivey, G. N., Bluteau, C. E. & Jones, N. L. 2018 Quantifying diapycnal mixing in an energetic ocean. J. Geophys. Res. 123 (1), 346–357.CrossRefGoogle Scholar
Jonker, H. J. J. & Jiménez, M. A. 2014 Laboratory experiments on convective entrainment using a saline water tank. Boundary Layer Meteorol. 151 (3), 479–500.CrossRefGoogle Scholar
Kirkpatrick, M. P. 2002 A large eddy simulation code for industrial and environmental flows. PhD thesis, School of Aerospace, Mechanical and Mechatronic Engineering, Faculty of Engineering, University of Sydney.Google Scholar
Kirkpatrick, M. P., Williamson, N., Armfield, S. W. & Zecevic, V. 2019 Evolution of thermally stratified turbulent open channel flow after removal of the heat source. J. Fluid Mech. 876, 356–412.CrossRefGoogle Scholar
Koren, I., Kaufman, Y. J., Remer, L. A. & Martins, J. V. 2004 Measurement of the effect of Amazon smoke on inhibition of cloud formation. Science 303 (5662), 1342–1345.CrossRefGoogle ScholarPubMed
de Lozar, A. & Mellado, J. P. 2013 Direct numerical simulations of a smoke cloud–top mixing layer as a model for stratocumuli. J. Atmos. Sci. 70 (8), 2356–2375.CrossRefGoogle Scholar
Marshall, J. & Schott, F. 1999 Open-ocean convection: observations, theory, and models. Rev. Geophys. 37 (1), 1–64.CrossRefGoogle Scholar
Mater, B. D. & Venayagamoorthy, S. K. 2014 The quest for an unambiguous parameterization of mixing efficiency in stably stratified geophysical flows. Geophys. Res. Lett. 41 (13), 4646–4653.CrossRefGoogle Scholar
Mellado, J. P. 2017 Cloud-top entrainment in stratocumulus clouds. Annu. Rev. Fluid Mech. 49 (1), 145–169.CrossRefGoogle Scholar
Mellado, J. P., Stevens, B., Schmidt, H. & Peters, N. 2009 Buoyancy reversal in cloud-top mixing layers. Q. J. R. Meteorol. Soc. 135 (641), 963–978.CrossRefGoogle Scholar
Mitrovic, S. M., Oliver, R. L., Rees, C., Bowling, L. C. & Buckney, R. T. 2003 Critical flow velocities for the growth and dominance of Anabaena circinalis in some turbid freshwater rivers. Freshwat. Biol. 48 (1), 164–174.CrossRefGoogle Scholar
Moritz, C., Blackall, L., Davis, J., Flannery, T., Godden, L., Head, L., Jackson, S., Kingsford, R., Wheeler, S & Williams, J. 2019 Investigation of the causes of mass fish kills in the Menindee Region NSW over the summer of 2018–2019. Tech. Rep. Australian Academy of Science.Google Scholar
Osborn, T. R. 1980 Estimates of the local state of vertical diffusion from dissipation measurements. J.Phys. Oceanogr. 10 (1), 83–89.2.0.CO;2>CrossRefGoogle Scholar
Osborn, T. R. & Cox, C. S. 1972 Oceanic fine structure. Geophys. Fluid Dyn. 3 (1), 321–345.CrossRefGoogle Scholar
Paluch, I. R. & Lenschow, D. H. 1991 Stratiform cloud formation in the marine boundary layer. J.Atmos. Sci. 48 (19), 2141–2158.2.0.CO;2>CrossRefGoogle Scholar
Reinfelds, I. & Williams, S. 2012 Threshold flows for the breakdown of seasonally persistent thermal stratification: Shoalhaven River below Tallowa Dam, New South Wales, Australia. River Res. Appl. 28 (7), 893–907.CrossRefGoogle Scholar
Sayler, B. J. & Breidenthal, R. E. 1998 Laboratory simulations of radiatively induced entrainment in stratiform clouds. J. Geophys. Res. 103 (D8), 8827–8837.CrossRefGoogle Scholar
Schumm, S. A. 1968 River Adjustment to Altered Hydrologic Regimen, Murrumbidgee River and Paleochannels, Australia. U.S. Government Printing Office.CrossRefGoogle Scholar
Scotti, A. & White, B. 2016 The mixing efficiency of stratified turbulent boundary layers. J. Phys. Oceanogr. 46 (10), 3181–3191.CrossRefGoogle Scholar
Sherman, B. S., Webster, I. T., Jones, G. J. & Oliver, R. L. 1998 Transitions between Auhcoseira and Anabaena dominance in a turbid river weir pool. Limnol. Oceanogr. 43, 1902–1915.CrossRefGoogle Scholar
Shih, L. H., Koseff, J. R., Ferziger, J. H. & Rehmann, C. R. 2000 Scaling and parameterization of stratified homogeneous turbulent shear flow. J. Fluid Mech. 412, 1–20.CrossRefGoogle Scholar
Shih, L. H., Koseff, J. R., Ivey, G. N. & Ferziger, J. H. 2005 Parameterization of turbulent fluxes and scales using homogeneous sheared stably stratified turbulence simulations. J. Fluid Mech. 525, 193–214.CrossRefGoogle Scholar
Simpson, J. H. & Hunter, J. R. 1974 Fronts in the Irish Sea. Nature 250 (5465), 404–406.CrossRefGoogle Scholar
Strang, E. J. & Fernando, H. J. S. 2001 Entrainment and mixing in stratified shear flows. J. Fluid Mech. 428, 349–386.CrossRefGoogle Scholar
Taylor, J. R., Sarkar, S. & Armenio, V. 2005 Large eddy simulation of stably stratified open channel flow. Phys. Fluids 17 (11), 116602.CrossRefGoogle Scholar
Ulloa, H. N., Winters, K. B., Wüest, A. & Bouffard, D. 2019 Differential heating drives downslope flows that accelerate mixed-layer warming in ice-covered waters. Geophys. Res. Lett. 46 (23), 13872–13882.CrossRefGoogle Scholar
Walker, R., Tejada-Martínez, A. E., Martinat, G. & Grosch, C. E. 2014 Large-eddy simulation of open channel flow with surface cooling. Intl J. Heat Fluid Flow 50, 209–224.CrossRefGoogle Scholar
Walter, R. K., Squibb, M. E., Woodson, C. B., Koseff, J. R. & Monismith, S. G. 2014 Stratified turbulence in the nearshore coastal ocean: dynamics and evolution in the presence of internal bores. J. Geophys. Res. 119 (12), 8709–8730.CrossRefGoogle Scholar
Webster, I. T., Sherman, B. S., Bormans, M. & Jones, G. 2000 Management strategies for cyanobacterial blooms in an impounded lowland river. Regul. Rivers: Res. Manage. 16 (5), 513–525.3.0.CO;2-B>CrossRefGoogle Scholar
Williamson, N., Armfield, S. W., Kirkpatrick, M. P. & Norris, S. E. 2015 Transition to stably stratified states in open channel flow with radiative surface heating. J. Fluid Mech. 766, 528–555.CrossRefGoogle Scholar
Williamson, N., Kirkpatrick, M. P. & Armfield, S. W. 2018 Entrainment across a sheared density interface in a cavity flow. J. Fluid Mech. 835, 999–1021.CrossRefGoogle Scholar
Wood, R. 2012 Stratocumulus clouds. Mon. Weath. Rev. 140 (8), 2373–2423.CrossRefGoogle Scholar
Zhou, Q., Taylor, J. R. & Caulfield, C. P. 2017 Self-similar mixing in stratified plane Couette flow for varying Prandtl number. J. Fluid Mech. 820, 86–120.CrossRefGoogle Scholar
Zikanov, O., Slinn, D. N. & Dhanak, M. R. 2002 Turbulent convection driven by surface cooling in shallow water. J. Fluid Mech. 464, 81–111.CrossRefGoogle Scholar

Kirkpatrick et al. supplementary movie 2

Evolution of temperature and vorticity fields for time, t = 1 to 2. Top panel temperature. Bottom panel vorticity. Temperature range: -18 to 45. Vorticity range: 0 to 300.

Download Kirkpatrick et al. supplementary movie 2(Video)
Video 9.5 MB

Kirkpatrick et al. supplementary movie 3

Evolution of temperature and vorticity fields for time, t = 2 to 3. Top panel temperature. Bottom panel vorticity. Temperature range: -15 to 20. Vorticity range: 0 to 300.

Download Kirkpatrick et al. supplementary movie 3(Video)
Video 9.6 MB

Kirkpatrick et al. supplementary movie 4

Evolution of temperature and vorticity fields for time, t = 3 to 4. Top panel temperature. Bottom panel vorticity. Temperature range: -25 to 8. Vorticity range: 0 to 300.

Download Kirkpatrick et al. supplementary movie 4(Video)
Video 9.2 MB

Kirkpatrick et al. supplementary movie 5

Evolution of temperature and vorticity fields for time, t = 4 to 5. Top panel temperature. Bottom panel vorticity. Temperature range: -25 to 3. Vorticity range: 0 to 300.

Download Kirkpatrick et al. supplementary movie 5(Video)
Video 9.7 MB