Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Hearst, R. Jason
and
Lavoie, Philippe
2014.
Scale-by-scale energy budget in fractal element grid-generated turbulence.
Journal of Turbulence,
Vol. 15,
Issue. 8,
p.
540.
Isaza, Juan C.
Salazar, Ricardo
and
Warhaft, Zellman
2014.
On grid-generated turbulence in the near- and far field regions.
Journal of Fluid Mechanics,
Vol. 753,
Issue. ,
p.
402.
Meldi, Marcello
Lejemble, Hugo
and
Sagaut, Pierre
2014.
On the emergence of non-classical decay regimes in multiscale/fractal generated isotropic turbulence.
Journal of Fluid Mechanics,
Vol. 756,
Issue. ,
p.
816.
Vassilicos, J. Christos
2015.
Dissipation in Turbulent Flows.
Annual Review of Fluid Mechanics,
Vol. 47,
Issue. 1,
p.
95.
Laizet, S.
and
Vassilicos, J. C.
2015.
Stirring and scalar transfer by grid-generated turbulence in the presence of a mean scalar gradient.
Journal of Fluid Mechanics,
Vol. 764,
Issue. ,
p.
52.
Hearst, R. J.
and
Lavoie, P.
2015.
The effect of active grid initial conditions on high Reynolds number turbulence.
Experiments in Fluids,
Vol. 56,
Issue. 10,
Zhou, Yi
Nagata, Koji
Sakai, Yasuhiko
Ito, Yasumasa
and
Hayase, Toshiyuki
2015.
On the evolution of the invariants of the velocity gradient tensor in single-square-grid-generated turbulence.
Physics of Fluids,
Vol. 27,
Issue. 7,
Hearst, R. J.
and
Lavoie, P.
2015.
Velocity derivative skewness in fractal-generated, non-equilibrium grid turbulence.
Physics of Fluids,
Vol. 27,
Issue. 7,
Fragner, Romain
Mazellier, Nicolas
Halter, Fabien
Chauveau, Christian
and
Gökalp, Iskender
2015.
Multi-Scale High Intensity Turbulence Generator Applied to a High Pressure Turbulent Burner.
Flow, Turbulence and Combustion,
Vol. 94,
Issue. 1,
p.
263.
Laizet, S.
Nedić, J.
and
Vassilicos, J. C.
2015.
The spatial origin of −5/3 spectra in grid-generated turbulence.
Physics of Fluids,
Vol. 27,
Issue. 6,
Djenidi, L.
Kamruzzaman, Md.
and
Antonia, R. A.
2015.
Power-law exponent in the transition period of decay in grid turbulence.
Journal of Fluid Mechanics,
Vol. 779,
Issue. ,
p.
544.
Tang, S. L.
Antonia, R. A.
Djenidi, L.
Abe, H.
Zhou, T.
Danaila, L.
and
Zhou, Y.
2015.
Transport equation for the mean turbulent energy dissipation rate on the centreline of a fully developed channel flow.
Journal of Fluid Mechanics,
Vol. 777,
Issue. ,
p.
151.
Valente, P. C.
and
Vassilicos, J. C.
2015.
The energy cascade in grid-generated non-equilibrium decaying turbulence.
Physics of Fluids,
Vol. 27,
Issue. 4,
Hearst, R. J.
and
Lavoie, P.
2016.
Progress in Turbulence VI.
Vol. 165,
Issue. ,
p.
229.
Nedić, J.
and
Tavoularis, S.
2016.
Measurements of passive scalar diffusion downstream of regular and fractal grids.
Journal of Fluid Mechanics,
Vol. 800,
Issue. ,
p.
358.
Meldi, M.
2016.
The signature of initial production mechanisms in isotropic turbulence decay.
Physics of Fluids,
Vol. 28,
Issue. 3,
Hearst, R. Jason
Gomit, Guillaume
and
Ganapathisubramani, Bharathram
2016.
Effect of turbulence on the wake of a wall-mounted cube.
Journal of Fluid Mechanics,
Vol. 804,
Issue. ,
p.
513.
Nedić, Jovan
and
Tavoularis, Stavros
2016.
Energy dissipation scaling in uniformly sheared turbulence.
Physical Review E,
Vol. 93,
Issue. 3,
TAKAMURE, Kotaro
and
OZONO, Shigehira
2016.
Effects of Inflow Conditions on the Turbulence Generated by Multiple Fans.
Journal of Wind Engineering,
Vol. 41,
Issue. 4,
p.
148.
Vergine, Fabrizio
Ground, Cody
and
Maddalena, Luca
2016.
Turbulent kinetic energy decay in supersonic streamwise interacting vortices.
Journal of Fluid Mechanics,
Vol. 807,
Issue. ,
p.
353.
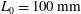 $L_0 = 100\, {\rm mm}$. Measurements were performed in the region
$L_0 = 100\, {\rm mm}$. Measurements were performed in the region  $3.5 \le x/L_0 \le 48.5$, which represents a significant extension to the
$3.5 \le x/L_0 \le 48.5$, which represents a significant extension to the 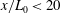 $x/L_0 < 20$ of previously reported square fractal grid measurements. For the region
$x/L_0 < 20$ of previously reported square fractal grid measurements. For the region 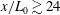 $x/L_0 \gtrsim 24$ it was found that a power-law decay region following
$x/L_0 \gtrsim 24$ it was found that a power-law decay region following 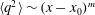 $\langle {q}^2 \rangle \sim (x - x_0)^m$ exists with decay exponents of
$\langle {q}^2 \rangle \sim (x - x_0)^m$ exists with decay exponents of 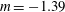 $m = -1.39$ and
$m = -1.39$ and 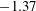 $-1.37$ at
$-1.37$ at  $\mathit{Re}_{L_0} = 57\, 000$ and
$\mathit{Re}_{L_0} = 57\, 000$ and 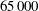 $65\, 000$, respectively. This agrees with decay values previously measured for regular grids (
$65\, 000$, respectively. This agrees with decay values previously measured for regular grids ( $-1 \gtrsim m \gtrsim -1.4$). The turbulence in the near-grid region,
$-1 \gtrsim m \gtrsim -1.4$). The turbulence in the near-grid region, 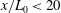 $x/L_0 < 20$, is shown to be inhomogeneous and anisotropic, in apparent contrast with previous fractal grid measurements. Nonetheless, power-law fits to the decay of turbulent kinetic energy in this region result in
$x/L_0 < 20$, is shown to be inhomogeneous and anisotropic, in apparent contrast with previous fractal grid measurements. Nonetheless, power-law fits to the decay of turbulent kinetic energy in this region result in  $m = -2.79$, similar to
$m = -2.79$, similar to 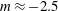 $m \approx -2.5$ recently reported by Valente & Vassilicos (J. Fluid Mech., vol. 687, 2011, pp. 300–340) for space-filling square fractals. It was also found that
$m \approx -2.5$ recently reported by Valente & Vassilicos (J. Fluid Mech., vol. 687, 2011, pp. 300–340) for space-filling square fractals. It was also found that 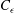 $C_\epsilon $ is approximately constant for
$C_\epsilon $ is approximately constant for 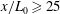 $x/L_0 \ge 25$, while it grows rapidly for
$x/L_0 \ge 25$, while it grows rapidly for 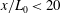 $x/L_0 < 20$. These results reconcile previous fractal-generated turbulence measurements with classical grid turbulence measurements.
$x/L_0 < 20$. These results reconcile previous fractal-generated turbulence measurements with classical grid turbulence measurements.