Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-26T16:37:02.208Z Has data issue: false hasContentIssue false
  • English
  • Français

Computational modelling of flow through prosthetic heart valves using the entropic lattice-Boltzmann method

Published online by Cambridge University Press:  03 March 2014

B. Min Yun ,
L. P. Dasi ,
C. K. Aidun  and
A. P. Yoganathan
B. Min Yun
Affiliation:
G. W. Woodruff School of Mechanical Engineering, Georgia Institute of Technology, 500 10th Street NW, Atlanta, GA 30318, USA
L. P. Dasi
Affiliation:
Department of Mechanical Engineering, Colorado State University, Campus Delivery 1374, Fort Collins, CO 80523, USA
C. K. Aidun
Affiliation:
G. W. Woodruff School of Mechanical Engineering, Georgia Institute of Technology, 500 10th Street NW, Atlanta, GA 30318, USA Parker H. Petit Institute for Bioengineering and Bioscience, Georgia Institute of Technology, 315 Ferst Drive, Atlanta, GA 30332, USA
A. P. Yoganathan*
Affiliation:
G. W. Woodruff School of Mechanical Engineering, Georgia Institute of Technology, 500 10th Street NW, Atlanta, GA 30318, USA Wallace H. Coulter Department of Biomedical Engineering, Georgia Institute of Technology and Emory University, 313 Ferst Drive, Atlanta, GA 30332, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Previous clinical, in vitro experimental and in silico simulation studies have shown the complex dynamics of flow through prosthetic heart valves. In the case of bileaflet mechanical heart valves (BMHVs), complex flow phenomena are observed due to the presence of two rigid leaflets. A numerical method for this type of study must be able to accurately simulate pulsatile flow through BMHVs with the inclusion of leaflet motion and high-Reynolds-number flow modelling. Consequently, this study aims at validating a numerical method that captures the flow dynamics for pulsatile flow through a BMHV. 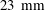A $23~ \mbox{mm}$ St. Jude Medical (SJM) Regent™ valve is selected for use in both the experiments and numerical simulations. The entropic lattice-Boltzmann method is used to simulate pulsatile flow through the valve with the inclusion of reverse leakage flow, while prescribing the flowrate and leaflet motion from experimental data. The numerical simulations are compared against experimental digital particle image velocimetry (DPIV) results from a previous study for validation. The numerical method is shown to match well with the experimental results quantitatively as well as qualitatively. Simulations are performed with efficient parallel processing at very high spatiotemporal resolution that can capture the finest details in the pulsatile BMHV flow field. This study validates the lattice-Boltzmann method as suitable for simulating pulsatile, high-Reynolds-number flows through prosthetic devices for use in future research.

JFM classification

Biological Fluid Dynamics: Biomedical flows Mathematical Foundations: Computational methods Vortex Flows: Vortex dynamics
Type
Papers
Information
Journal of Fluid Mechanics , Volume 743 , 25 March 2014 , pp. 170 - 201
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Aidun, C. K. & Clausen, J. R. 2010 Lattice Boltzmann method for complex flows. Annu. Rev. Fluid Mech. 42, 439–472.CrossRefGoogle Scholar
Aidun, C. K. & Lu, Y. 1995 Lattice Boltzmann simulation of solid particles suspended in fluid. J. Stat. Phys. 81 (1), 49–61.Google Scholar
Aidun, C. K., Lu, Y. & Ding, E. J. 1998 Direct analysis of particulate suspensions with inertia using the discrete Boltzmann equation. J. Fluid Mech. 373, 287–311.Google Scholar
Black, M. M. & Drury, P. J. 1994 Mechanical and other problems of artificial valves. The Pathology of Devices 86, 127–159.Google Scholar
Borazjani, I., Ge, L. & Sotiropoulos, F. 2008 Curvilinear immersed boundary method for simulating fluid structure interaction with complex 3D rigid bodies. J. Comput. Phys. 227 (16), 7587–7620.Google Scholar
Borazjani, I. & Sotiropoulos, F. 2010 The effect of implantation orientation of a bileaflet mechanical heart valve on kinematics and hemodynamics in an anatomic aorta. J. Biomech. Engng 132, 111005.CrossRefGoogle Scholar
Chikatamarla, S. S., Frouzakis, C. E., Karlin, I. V., Tomboulides, A. G. & Boulouchos, K. B. 2010 Lattice Boltzmann method for direct numerical simulation of turbulent flows. J. Fluid Mech. 656, 298–308.Google Scholar
Clausen, J. R., Reasor, D. A. Jr & Aidun, C. K. 2010 Parallel performance of a lattice-Boltzmann/finite element cellular blood flow solver on the IBM Blue Gene/P architecture. Comput. Phys. Commun. 181 (6), 1013–1020.Google Scholar
Dasi, L. P., Ge, L., Simon, H. A., Sotiropoulos, F. & Yoganathan, A. P. 2007 Vorticity dynamics of a bileaflet mechanical heart valve in an axisymmetric aorta. Phys. Fluids 19, 067105.Google Scholar
De Tullio, M. D., Cristallo, A., Balaras, E. & Verzicco, R. 2010 Direct numerical simulation of the pulsatile flow through an aortic bileaflet mechanical heart valve. J. Fluid Mech. 622, 259–290.Google Scholar
Ellis, J. T., Healy, T. M., Fontaine, A. A., Saxena, R. & Yoganathan, A. P. 1996 Velocity measurements and flow patterns within the hinge region of a medtronic parallel bileaflet mechanical valve with clear housing. J. Heart Valve Dis. 5 (6), 591–599.Google Scholar
Ellis, J. T. & Yoganathan, A. P. 2000 A comparison of the hinge and near-hinge flow fields of the st jude medical hemodynamic plus and regent bileaflet mechanical heart valves. J. Thorac. Cardiovasc. Surg. 119 (1), 83–93.Google Scholar
Fallon, A. M., Dasi, L. P., Marzec, U. M., Hanson, S. R. & Yoganathan, A. P. 2008 Procoagulant properties of flow fields in stenotic and expansive orifices. Ann. Biomed. Engng 36 (1), 1–13.CrossRefGoogle ScholarPubMed
Ge, L., Leo, H. L., Sotiropoulos, F. & Yoganathan, A. P. 2005 Flow in a mechanical bileaflet heart valve at laminar and near-peak systole flow rates: CFD simulations and experiments. J. Biomech. Engng 127, 782–797.Google Scholar
Giersiepen, M., Wurzinger, L. J., Opitz, R. & Reul, H. 1990 Estimation of shear stress-related blood damage in heart valve prostheses–in vitro comparison of 25 aortic valves. Intl J. Artif. Organs 13 (5), 300–306.CrossRefGoogle ScholarPubMed
Grunkemeier, G. L. & Anderson, W. N. Jr 1998 Clinical evaluation and analysis of heart valve substitutes. J. Heart Valve Dis. 7 (2), 163–169.Google Scholar
Keating, B., Vahala, G., Yepez, J., Soe, M. & Vahala, L. 2007 Entropic lattice Boltzmann representations required to recover Navier–Stokes flows. Phys. Rev. E 75 (3), 36712.Google Scholar
Lim, W. L., Chew, Y. T., Chew, T. C. & Low, H. T. 1994 Particle image velocimetry in the investigation of flow past artificial heart valves. Ann. Biomed. Engng 22 (3), 307–318.Google Scholar
Macmeccan, R. M., Clausen, J. R., Neitzel, G. P. & Aidun, C. K. 2008 Simulating deformable particle suspensions using a coupled lattice-Boltzmann and finite-element method. J. Fluid Mech. 618, 13–58.Google Scholar
Manning, K. B., Kini, V., Fontaine, A. A., Deutsch, S. & Tarbell, J. M. 2003 Regurgitant flow field characteristics of the st. jude bileaflet mechanical heart valve under physiologic pulsatile flow using particle image velocimetry. Artif. Organs 27 (9), 840–846.Google Scholar
Simon, H. A., Ge, L., Sotiropoulos, F. & Yoganathan, A. P. 2009 Simulation of the three-dimensional hinge flow fields of a bileaflet mechanical heart valve under aortic conditions. Ann. Biomed. Engng 38 (3), 841–853.Google Scholar
Simon, H. A., Ge, L., Sotiropoulos, F. & Yoganathan, A. P. 2010 Numerical investigation of the performance of three hinge designs of bileaflet mechanical heart valves. Ann. Biomed. Engng 38 (11), 3295–3310.Google Scholar
Vahala, G., Keating, B., Soe, M., Yepez, J., Vahala, L., Carter, J. & Ziegeler, S. 2008 MHD turbulence studies using lattice Boltzmann algorithms. Commun. Comput. Phys. 4, 624–646.Google Scholar
Vahala, G., Keating, B., Soe, M., Yepez, J., Vahala, L. & Ziegeler, S. 2009 Entropic, les and boundary conditions in lattice Boltzmann simulations of turbulence. Eur. Phys. J.-Spec. Top. 171 (1), 167–171.Google Scholar
Wu, J., Yun, B. M., Fallon, A. M., Hanson, S. R., Aidun, C. K. & Yoganathan, A. P. 2011 Numerical investigation of the effects of channel geometry on platelet activation and blood damage. Ann. Biomed. Engng 39 (2), 897–910.Google Scholar
Xenos, M., Girdhar, G., Alemu, Y., Jesty, J., Slepian, M., Einav, S. & Bluestein, D. 2010 Device Thrombogenicity Emulator (DTE)-design optimization methodology for cardiovascular devices: a study in two bileaflet MHV designs. J. Biomech. 43 (12), 2400–2409.Google Scholar
Yeung, P. K. & Pope, S. B. 1989 Lagrangian statistics from direct numerical simulations of isotropic turbulence. J. Fluid Mech. 207 (1), 531–586.Google Scholar
Yoganathan, A., Leo, H., Travis, B. & Teoh, S. 2003 Heart valve bioengineering. Encyclopedia of Comprehensive Structural Integrity (CSI). pp. 795–796. Elsevier Science.Google Scholar
Yun, B. M.2014 Simulations of pulsatile flow through bileaflet mechanical heart valves using a suspension flow model: to assess blood damage. PhD thesis, Georgia Institute of Technology.Google Scholar
Yun, B. M., Wu, J., Simon, H. A., Arjunon, S., Sotiropoulos, F., Aidun, C. K. & Yoganathan, A. P. 2012 A numerical investigation of blood damage in the hinge area of aortic bileaflet mechanical heart valves during the leakage phase. Ann. Biomed. Engng 40 (7), 1468–1485.Google Scholar
Zou, Q. & He, X.1996 On pressure and velocity flow boundary conditions and bounceback for the lattice Boltzmann BGK model. arXiv preprint comp-gas/9611001.Google Scholar