Article contents
Coherent noise sources of a subsonic round jet investigated using hydrodynamic and acoustic phased-microphone arrays
Published online by Cambridge University Press: 02 August 2013
Abstract
Based on phased-microphone array data acquired in the past, properties of coherent noise sources in a subsonic round jet are investigated at low frequencies (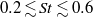 $0. 2\lesssim \mathit{St}\lesssim 0. 6$) via two approaches: (i) by extracting hydrodynamic fluctuations from the near-field array, instability-wave components are projected to the acoustic field using a boundary-value problem technique; (ii) by post-processing mid-field array data in an acoustic field, noise sources are decomposed into multipole distributions using a generalized-inverse beam-forming technique. Comparison between the projected acoustic fields from the hydrodynamic array and the sound pressure levels at the acoustic array implies that the near-field pressure fluctuations beyond the end of the potential core primarily contribute to the downstream sound, as mentioned by many previous studies. However, the jet-spreading effect, which creates the streamwise growth and decay of the eigenfunctions in linear stability analysis, is insufficient to generate the sound pressure levels measured in the acoustic array. In the actual hydrodynamic data, the streamwise decay is much slower and the phase velocity is faster than those of the corresponding eigenfunction beyond the peak of the wave-packet, and these factors govern the downstream sound. Results from the acoustic array demonstrate that free-space multipole distributions detected by generalized-inverse beam-forming can reproduce primary coherent modes, the first one predominantly propagating downstream and the second one typically being more omni-directional. In particular, the detected phase relation of the first coherent mode shows nearly a constant slope, indicating a wavy-type source structure and the relation of downstream sound with instability waves.
$0. 2\lesssim \mathit{St}\lesssim 0. 6$) via two approaches: (i) by extracting hydrodynamic fluctuations from the near-field array, instability-wave components are projected to the acoustic field using a boundary-value problem technique; (ii) by post-processing mid-field array data in an acoustic field, noise sources are decomposed into multipole distributions using a generalized-inverse beam-forming technique. Comparison between the projected acoustic fields from the hydrodynamic array and the sound pressure levels at the acoustic array implies that the near-field pressure fluctuations beyond the end of the potential core primarily contribute to the downstream sound, as mentioned by many previous studies. However, the jet-spreading effect, which creates the streamwise growth and decay of the eigenfunctions in linear stability analysis, is insufficient to generate the sound pressure levels measured in the acoustic array. In the actual hydrodynamic data, the streamwise decay is much slower and the phase velocity is faster than those of the corresponding eigenfunction beyond the peak of the wave-packet, and these factors govern the downstream sound. Results from the acoustic array demonstrate that free-space multipole distributions detected by generalized-inverse beam-forming can reproduce primary coherent modes, the first one predominantly propagating downstream and the second one typically being more omni-directional. In particular, the detected phase relation of the first coherent mode shows nearly a constant slope, indicating a wavy-type source structure and the relation of downstream sound with instability waves.
JFM classification
- Type
- Papers
- Information
- Copyright
- ©2013 Cambridge University Press
References
- 35
- Cited by


