Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Hearst, R. Jason
and
Ganapathisubramani, Bharathram
2017.
Tailoring incoming shear and turbulence profiles for lab‐scale wind turbines.
Wind Energy,
Vol. 20,
Issue. 12,
p.
2021.
Cummins, Cathal
Viola, Ignazio Maria
Mastropaolo, Enrico
and
Nakayama, Naomi
2017.
The effect of permeability on the flow past permeable disks at low Reynolds numbers.
Physics of Fluids,
Vol. 29,
Issue. 9,
Hu, Zhenghong
Lei, Jiarui
Liu, Chao
and
Nepf, Heidi
2018.
Wake structure and sediment deposition behind models of submerged vegetation with and without flexible leaves.
Advances in Water Resources,
Vol. 118,
Issue. ,
p.
28.
Bossuyt, Juliaan
Meneveau, Charles
and
Meyers, Johan
2018.
Effect of layout on asymptotic boundary layer regime in deep wind farms.
Physical Review Fluids,
Vol. 3,
Issue. 12,
Kurth, Sebastian
Hamann, Christian
Seume, Jörg R.
and
Mulleners, Karen
2018.
Experimental Investigation of the Influence of Anisotropic Surface Structures on the Boundary Layer Flow.
Hamed, A. M.
and
Chamorro, L. P.
2018.
Turbulent boundary layer around 2D permeable and impermeable obstacles.
Experiments in Fluids,
Vol. 59,
Issue. 9,
Tang, Tingting
Yu, Peng
Shan, Xiaowen
Chen, Huisu
and
Su, Jian
2019.
Investigation of drag properties for flow through and around square arrays of cylinders at low Reynolds numbers.
Chemical Engineering Science,
Vol. 199,
Issue. ,
p.
285.
Biggs, H. J.
Nikora, V. I.
Gibbins, C. N.
Cameron, S. M.
Papadopoulos, K.
Stewart, M.
Fraser, S.
Vettori, D.
Savio, M.
O’Hare, M. T.
Kucher, M.
and
Hicks, D. M.
2019.
Flow interactions with an aquatic macrophyte: a field study using stereoscopic particle image velocimetry.
Journal of Ecohydraulics,
Vol. 4,
Issue. 2,
p.
113.
Kosutova, Katarina
van Hooff, Twan
Vanderwel, Christina
Blocken, Bert
and
Hensen, Jan
2019.
Cross-ventilation in a generic isolated building equipped with louvers: Wind-tunnel experiments and CFD simulations.
Building and Environment,
Vol. 154,
Issue. ,
p.
263.
Klettner, C. A.
Eames, I.
and
Hunt, J. C. R.
2019.
The effect of an unsteady flow incident on an array of circular cylinders.
Journal of Fluid Mechanics,
Vol. 872,
Issue. ,
p.
560.
Zhdanov, Oleksandr
and
Busse, Angela
2019.
Angle of attack dependence of flow past cactus-inspired cylinders with a low number of ribs.
European Journal of Mechanics - B/Fluids,
Vol. 75,
Issue. ,
p.
244.
Zhou, Jian
and
Venayagamoorthy, Subhas K.
2019.
Near-field mean flow dynamics of a cylindrical canopy patch suspended in deep water.
Journal of Fluid Mechanics,
Vol. 858,
Issue. ,
p.
634.
Tang, Tingting
Yu, Peng
Shan, Xiaowen
and
Chen, Huisu
2019.
The formation mechanism of recirculating wake for steady flow through and around arrays of cylinders.
Physics of Fluids,
Vol. 31,
Issue. 4,
Klettner, Christian
2020.
The Transfer and Transport of a Passive Scalar Within an Isolated Array of Circular Cylinders in a Uniform Flow.
Water Resources Research,
Vol. 56,
Issue. 9,
Tang, Tingting
Yu, Peng
Yu, Shimin
Shan, Xiaowen
and
Chen, Huisu
2020.
Connection between pore-scale and macroscopic flow characteristics of recirculating wake behind a porous cylinder.
Physics of Fluids,
Vol. 32,
Issue. 8,
Tang, Tingting
Yu, Peng
Shan, Xiaowen
Li, Jianhui
and
Yu, Shimin
2020.
On the transition behavior of laminar flow through and around a multi-cylinder array.
Physics of Fluids,
Vol. 32,
Issue. 1,
Nicolai, C.
Taddei, S.
Manes, C.
and
Ganapathisubramani, B.
2020.
Wakes of wall-bounded turbulent flows past patches of circular cylinders.
Journal of Fluid Mechanics,
Vol. 892,
Issue. ,
Steiros, K.
Kokmanian, K.
Bempedelis, N.
and
Hultmark, M.
2020.
The effect of porosity on the drag of cylinders.
Journal of Fluid Mechanics,
Vol. 901,
Issue. ,
Montali-Ashworth, Daniella
Vowles, Andrew S.
de Almeida, Gustavo
and
Kemp, Paul S.
2020.
Use of Cylindrical Bristle Clusters as a novel multispecies fish pass to facilitate upstream movement at gauging weirs.
Ecological Engineering,
Vol. 143,
Issue. ,
p.
105634.
Huai, Wen-xin
Li, Shuolin
Katul, Gabriel G.
Liu, Meng-yang
and
Yang, Zhong-hua
2021.
Flow dynamics and sediment transport in vegetated rivers: A review.
Journal of Hydrodynamics,
Vol. 33,
Issue. 3,
p.
400.
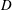 $D$) and are made of several identical circular cylinders (height,
$D$) and are made of several identical circular cylinders (height, 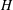 $H$, and diameter,
$H$, and diameter, 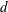 $d$). The bulk aspect ratio of all of the patches (
$d$). The bulk aspect ratio of all of the patches (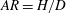 $AR=H/D$) was fixed at 1 and the patch density (
$AR=H/D$) was fixed at 1 and the patch density (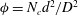 ${\it\phi}=N_{c}d^{2}/D^{2}$, also referred to as the solidity) is altered by varying the number of cylinders (
${\it\phi}=N_{c}d^{2}/D^{2}$, also referred to as the solidity) is altered by varying the number of cylinders ( $N_{c}$) in the patch. Drag measurements show that the patch drag coefficient increases with increasing density. However, the drag coefficient of the highest investigated density (
$N_{c}$) in the patch. Drag measurements show that the patch drag coefficient increases with increasing density. However, the drag coefficient of the highest investigated density (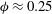 ${\it\phi}\approx 0.25$) is greater than the drag coefficient of a solid patch (i.e.
${\it\phi}\approx 0.25$) is greater than the drag coefficient of a solid patch (i.e. 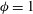 ${\it\phi}=1$, which is a solid cylinder with
${\it\phi}=1$, which is a solid cylinder with 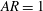 $AR=1$). Particle image velocimetry (PIV) measurements were carried out along the streamwise–wall-normal (
$AR=1$). Particle image velocimetry (PIV) measurements were carried out along the streamwise–wall-normal ( $x$–
$x$–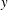 $y$) plane along the centreline of patch and in the streamwise–spanwise (
$y$) plane along the centreline of patch and in the streamwise–spanwise ( $x$–
$x$– $z$) plane at its mid height (i.e.
$z$) plane at its mid height (i.e.  $y=H/2$). Mean velocity fields show that the porosity of the patch promotes bleeding along all directions. It was observed that bleeding along the vertical/horizontal direction increases/decreases with increasing
$y=H/2$). Mean velocity fields show that the porosity of the patch promotes bleeding along all directions. It was observed that bleeding along the vertical/horizontal direction increases/decreases with increasing 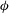 ${\it\phi}$. Furthermore, it was also observed that bleeding along the lateral direction dictates the point of flow separation along the sides of the patch and makes it independent of
${\it\phi}$. Furthermore, it was also observed that bleeding along the lateral direction dictates the point of flow separation along the sides of the patch and makes it independent of  ${\it\phi}$. All of these aspects make wakes for porous patches markedly different from their solid counterpart and provide a rational basis to explain the observed trends in the drag coefficient.
${\it\phi}$. All of these aspects make wakes for porous patches markedly different from their solid counterpart and provide a rational basis to explain the observed trends in the drag coefficient.