Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-17T11:24:00.286Z Has data issue: false hasContentIssue false
  • English
  • Français

Bulk temperature and heat transport in turbulent Rayleigh–Bénard convection of fluids with temperature-dependent properties

Published online by Cambridge University Press:  20 July 2018

Stephan Weiss Open the ORCID record for Stephan Weiss [Opens in a new window] ,
Xiaozhou He ,
Guenter Ahlers ,
Eberhard Bodenschatz  and
Olga Shishkina Open the ORCID record for Olga Shishkina [Opens in a new window]
Stephan Weiss
Affiliation:
Max Planck Institute for Dynamics and Self-Organization, Am Fassberg 17, 37077 Göttingen, Germany
Xiaozhou He
Affiliation:
Max Planck Institute for Dynamics and Self-Organization, Am Fassberg 17, 37077 Göttingen, Germany School of Mechanical Engineering and Automation, Harbin Institute of Technology, Shenzhen, China
Guenter Ahlers
Affiliation:
Max Planck Institute for Dynamics and Self-Organization, Am Fassberg 17, 37077 Göttingen, Germany Department of Physics, University of California, Santa Barbara, CA 93106, USA
Eberhard Bodenschatz
Affiliation:
Max Planck Institute for Dynamics and Self-Organization, Am Fassberg 17, 37077 Göttingen, Germany Institute for Nonlinear Dynamics, Georg-August-University Göttingen, 37073 Göttingen, Germany Laboratory of Atomic and Solid-State Physics and Sibley School of Mechanical and Aerospace Engineering, Cornell University, Ithaca, NY 14853, USA
Olga Shishkina*
Affiliation:
Max Planck Institute for Dynamics and Self-Organization, Am Fassberg 17, 37077 Göttingen, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

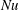We critically analyse the different ways to evaluate the dependence of the Nusselt number ($\mathit{Nu}$) on the Rayleigh number (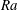$\mathit{Ra}$) in measurements of the heat transport in turbulent Rayleigh–Bénard convection under general non-Oberbeck–Boussinesq conditions and show the sensitivity of this dependence to the choice of the reference temperature at which the fluid properties are evaluated. For the case when the fluid properties depend significantly on the temperature and any pressure dependence is insignificant we propose a method to estimate the centre temperature. The theoretical predictions show very good agreement with the Göttingen measurements by He et al. (New J. Phys., vol. 14, 2012, 063030). We further show too the values of the normalized heat transport 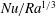$\mathit{Nu}/\mathit{Ra}^{1/3}$ are independent of whether they are evaluated in the whole convection cell or in the lower or upper part of the cell if the correct reference temperatures are used.

JFM classification

Convection: Convection
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 851 , 25 September 2018 , pp. 374 - 390
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlers, G., Araujo, F. F., Funfschilling, D., Grossmann, S. & Lohse, D. 2007 Non-Oberbeck–Boussinesq effects in gaseous Rayleigh–Bénard convection. Phys. Rev. Lett. 98, 054501.Google Scholar
Ahlers, G., Bodenschatz, E., Funfschilling, D., Grossmann, S., He, X., Lohse, D., Stevens, R. J. A. M. & Verzicco, R. 2012a Logarithmic temperature profiles in turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 109, 114501.Google Scholar
Ahlers, G., Bodenschatz, E. & He, X. 2014 Logarithmic temperature profiles of turbulent Rayleigh–Bénard convection in the classical and ultimate state for a Prandtl number of 0.8. J. Fluid Mech. 758, 436–467.Google Scholar
Ahlers, G., Brown, E., Araujo, F. F., Funfschilling, D., Grossmann, S. & Lohse, D. 2006 Non-Oberbeck–Boussinesq effects in strongly turbulent Rayleigh–Bénard convection. J. Fluid Mech. 569, 409–446.Google Scholar
Ahlers, G., Calzavarini, E., Araujo, F. F., Funfschilling, D., Grossmann, S., Lohse, D. & Sugiyama, K. 2008 Non-Oberbeck–Boussinesq effects in turbulent thermal convection in ethane close to the critical point. Phys. Rev. E 77, 046302.Google Scholar
Ahlers, G., Grossmann, S. & Lohse, D. 2009 Heat transfer and large scale dynamics in turbulent Rayleigh–Bénard convection. Rev. Mod. Phys. 81, 503–537.Google Scholar
Ahlers, G., He, X., Funfschilling, D. & Bodenschatz, E. 2012b Heat transport by turbulent Rayleigh–Bénard convection for Pr⋍0. 8 and 3 × 1012 ≲Ra ≲ 1015 : aspect ratio 𝛤 = 0. 50. New J. Phys. 14, 103012.Google Scholar
Ashkenazi, S. & Steinberg, V. 1999 High Rayleigh number turbulent convection in a gas near the gas–liquid critical point. Phys. Rev. Lett. 83, 3641–3644.Google Scholar
Bodenschatz, E., Pesch, W. & Ahlers, G. 2000 Recent developments in Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 32, 709–778.Google Scholar
Boussinesq, J. 1903 Théorie Analytique de la Chaleur. Gauthier-Villars.Google Scholar
Burnishev, Y., Segre, E. & Steinberg, V. 2010 Strong symmetrical non-Oberbeck–Boussinesq turbulent convection and the role of compressibility. Phys. Fluids 22, 035108.Google Scholar
Busse, F. H. 1967 The stability of finite amplitude cellular convection and its relation to an extremum principle. J. Fluid Mech. 30, 625–649.Google Scholar
Castaing, B., Gunaratne, G., Heslot, F., Kadanoff, L., Libchaber, A., Thomae, S., Wu, X.-Z., Zaleski, S. & Zanetti, G. 1989 Scaling of hard thermal turbulence in Rayleigh–Bénard convection. J. Fluid Mech. 204, 1–30.Google Scholar
Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. Eur. Phys. J. E 35, 58.Google Scholar
Ching, E. S. C., Dung, O.-Y. & Shishkina, O. 2017 Fluctuating thermal boundary layers and heat transfer in turbulent Rayleigh–Bénard convection. J. Stat. Phys. 167, 626–635.Google Scholar
Chung, M. K., Yun, H. C. & Adrian, R. J. 1992 Scale analysis and wall-layer model for the temperature profile in a turbulent thermal convection. Intl J. Heat Mass Transfer 35, 43–51.Google Scholar
Gray, D. D. & Giorgini, A. 1976 The validity of the Boussinesq approximation for liquids and gases. Intl J. Heat Mass Transfer 19, 545–551.Google Scholar
Grossmann, S. & Lohse, D. 2000 Scaling in thermal convection: a unifying theory. J. Fluid Mech. 407, 27–56.Google Scholar
Grossmann, S. & Lohse, D. 2001 Thermal convection for large Prandtl numbers. Phys. Rev. Lett. 86, 3316–3319.Google Scholar
Grossmann, S. & Lohse, D. 2004 Fluctuations in turbulent Rayleigh–Bénard convection: the role of plumes. Phys. Fluids 16, 4462–4472.Google Scholar
Grossmann, S. & Lohse, D. 2011 Multiple scaling in the ultimate regime of thermal convection. Phys. Fluids 23, 045108.Google Scholar
He, X., Bodenschatz, E. & Ahlers, G. 2016 Azimuthal diffusion of the large-scale-circulation plane, and absence of significant non-Boussinesq effects, in turbulent convection near the ultimate-state transition. J. Fluid Mech. 791, R3.Google Scholar
He, X., Funfschilling, D., Bodenschatz, E. & Ahlers, G. 2012 Heat transport by turbulent Rayleigh–Bénard convection for Pr⋍0. 8 and 4 × 1011 ≲Ra ≲ 2 × 1014 : ultimate-state transition for aspect ratio 𝛤 = 1. 00. New J. Phys. 14, 063030.Google Scholar
Horn, S. & Shishkina, O. 2014 Rotating non-Oberbeck–Boussinesq Rayleigh-Bénard convection in water. Phys. Fluids 26, 055111.Google Scholar
Horn, S., Shishkina, O. & Wagner, C. 2013 On non-Oberbeck–Boussinesq effects in three-dimensional Rayleigh–Bénard convection in glycerol. J. Fluid Mech. 724, 175–202.Google Scholar
Howard, L. N. 1966 Convection at high Rayleigh number. In Applied Mechanics (ed. Görtler, H.), pp. 1109–1115. Springer.Google Scholar
Kraichnan, R. 1962 Turbulent thermal convection at arbitrary Prandtl number. Phys. Fluids 5, 1374–1389.Google Scholar
Lohse, D. & Xia, K.-Q. 2010 Small-scale properties of turbulent Rayleigh–Bénard convection. Annu. Rev. Fluid Mech. 42, 335–364.Google Scholar
Malkus, M. V. R. 1954 The heat transport and spectrum of thermal turbulence. Proc. R. Soc. Lond. A 225, 196–212.Google Scholar
Manga, M. & Weeraratne, D. 1999 Experimental study of non-Boussinesq Rayleigh–Bénard convection at high Rayleigh and Prandtl numbers. Phys. Fluids 11 (10), 2969–2976.Google Scholar
Niemela, J. J., Skrbek, L., Sreenivasan, K. R. & Donnely, R. J. 2000 Turbulent convection at very high Rayleigh numbers. Nature 404, 837–841.Google Scholar
Niemela, J. J. & Sreenivasan, K. R. 2003 Confined turbulent convection. J. Fluid Mech. 481, 355–384.Google Scholar
Oberbeck, A. 1879 Über die Wärmeleitung der Flüssigkeiten bei Berücksichtigung der Strömungen in Folge von Temperaturdifferenzen. Ann. Phys. (Berlin) 243 (6), 271–292.Google Scholar
du Puits, R., Resagk, C. & Thess, A. 2013 Thermal boundary layers in turbulent Rayleigh–Bénard convection at aspect ratios between 1 and 9. New J. Phys. 15, 013040.Google Scholar
du Puits, R., Resagk, C., Tilgner, A., Busse, F. H. & Thess, A. 2007 Structure of thermal boundary layers in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 572, 231–254.Google Scholar
Roche, P. E., Castaing, B., Chabaud, B. & Hebral, B. 2004 Heat transfer in turbulent Rayleigh–Bénard convection below the ultimate regime. J. Low. Temp. Phys. 134, 1011–1042.Google Scholar
Scheel, J. D., Kim, E. & White, K. R. 2012 Thermal and viscous boundary layers in turbulent Rayleigh–Bénard convection. J. Fluid Mech. 711, 281–305.Google Scholar
Shishkina, O., Emran, M., Grossmann, S. & Lohse, D. 2017a Scaling relations in large-Prandtl-number natural thermal convection. Phys. Rev. Fluids 2, 103502.Google Scholar
Shishkina, O., Horn, S., Emran, M. & Ching, E. S. C. 2017b Mean temperature profiles in turbulent thermal convection. Phys. Rev. Fluids 2, 113502.Google Scholar
Shishkina, O., Horn, S. & Wagner, S. 2013 Falkner–Skan boundary layer approximation in Rayleigh–Bénard convection. J. Fluid Mech. 730, 442–463.Google Scholar
Shishkina, O., Horn, S., Wagner, S. & Ching, E. S. C. 2015 Thermal boundary layer equation for turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 114, 114302.Google Scholar
Shishkina, O., Wagner, S. & Horn, S. 2014 Influence of the angle between the wind and the isothermal surfaces on the boundary layer structures in turbulent thermal convection. Phys. Rev. E 89, 033014.Google Scholar
Shishkina, O., Weiss, S. & Bodenschatz, E. 2016 Conductive heat flux in measurements of the Nusselt number in turbulent Rayleigh–Bénard convection. Phys. Rev. Fluids 1, 062301(R).Google Scholar
Spiegel, E. A. & Veronis, G. 1960 On the Boussinesq approximation for a compressible fluid. Astrophys. J. 131, 442–447.Google Scholar
Stevens, R. J. A. M., Zhou, Q., Grossmann, S., Verzicco, R., Xia, K.-Q. & Lohse, D. 2012 Thermal boundary layer profiles in turbulent Rayleigh–Bénard convection in a cylindrical sample. Phys. Rev. E 85, 027301.Google Scholar
Sugiyama, K., Calzavarini, E., Grossmann, S. & Lohse, D. 2007 Non-Oberbeck–Boussinesq effects in two-dimensional Rayleigh–Bénard convection in glycerol. Europhys. Lett. 80, 34002.Google Scholar
Sugiyama, K., Calzavarini, E., Grossmann, S. & Lohse, D. 2009 Flow organization in two-dimensional non-Oberbeck–Boussinesq Rayleigh–Bénard convection in water. J. Fluid Mech. 637, 105–135.Google Scholar
Urban, P., Hanzelka, P., Musilova, V., Kralik, T., Mantia, M. L., Srnka, A. & Skrbek, L. 2014 Heat transfer in cryogenic helium gas by turbulent Rayleigh–Bénard convection in a cylindrical cell of aspect ratio 1. New J. Phys. 16, 053042.Google Scholar
Wei, P. & Ahlers, G. 2014 Logarithmic temperature profiles in the bulk of turbulent Rayleigh–Bénard convection for a Prandtl number of 12.3. J. Fluid Mech. 758, 809–830.Google Scholar
Wu, X. Z. & Libchaber, A. 1991 Non-Boussinesq effects in free thermal convection. Phys. Rev. A 43 (6), 2833–2839.Google Scholar
Xia, K.-Q., Lam, S. & Zhou, S. Q. 2002 Heat-flux measurement in high-Prandtl-number turbulent Rayleigh–Bénard convection. Phys. Rev. Lett. 88, 064501.Google Scholar
Zhang, J., Childress, S. & Libchaber, A. 1997 Non-Boussinesq effect: thermal convection with broken symmetry. Phys. Fluids 9, 1034–1042.Google Scholar