Article contents
Bifurcation of nonlinear Tollmien–Schlichting waves in a high-speed channel flow
Published online by Cambridge University Press: 16 March 2018
Abstract
Plane Poiseuille flow has long served as the simplest testing ground for Tollmien–Schlichting wave instability. In this paper, we provide a comprehensive comparison of equilibrium Tollmien–Schlichting wave solutions arising from new high-resolution Navier–Stokes calculations and the corresponding predictions of various large-Reynolds-number asymptotic theories developed in the last century, such as double-deck theory, viscous nonlinear critical layer theory and strongly nonlinear critical layer theory. In the relatively small to moderate amplitude regime, the theories excellently predict the behaviour of the numerical solutions at Reynolds numbers of order 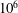 $10^{6}$ and above, whilst for larger amplitudes our computations suggest the need for further asymptotic theories to be developed.
$10^{6}$ and above, whilst for larger amplitudes our computations suggest the need for further asymptotic theories to be developed.
- Type
- JFM Papers
- Information
- Copyright
- © 2018 Cambridge University Press
References
- 9
- Cited by


