Published online by Cambridge University Press: 12 April 2011
We consider theoretically the long-time evolution of axisymmetric, high Reynolds number, Boussinesq gravity currents supplied by a constant, small-area source of mass and radial momentum in a deep, quiescent ambient. We describe the gravity currents using a shallow-water model with a Froude number closure condition to incorporate ambient form drag at the front and present numerical and asymptotic solutions. The predicted profile consists of an expanding, radially decaying, steady interior that connects via a shock to a deeper, self-similar frontal boundary layer. Controlled by the balance of interior momentum flux and frontal buoyancy across the shock, the front advances as (g′sQ/r1/4s)4/15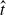 4/5, where g′s is the reduced gravity of the source fluid, Q is the total volume flux, rs is the source radius and
4/5, where g′s is the reduced gravity of the source fluid, Q is the total volume flux, rs is the source radius and 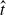 is time. A radial momentum source has no effect on this solution below a non-zero threshold value. Above this value, the (virtual) radius over which the flow becomes critical can be used to collapse the solution onto the subthreshold one. We also use a simple parameterization to incorporate the effect of interfacial entrainment, and show that the profile can be substantially modified, although the buoyancy profile and radial extent are less significantly impacted. Our predicted profiles and extents are in reasonable agreement with existing experiments.
is time. A radial momentum source has no effect on this solution below a non-zero threshold value. Above this value, the (virtual) radius over which the flow becomes critical can be used to collapse the solution onto the subthreshold one. We also use a simple parameterization to incorporate the effect of interfacial entrainment, and show that the profile can be substantially modified, although the buoyancy profile and radial extent are less significantly impacted. Our predicted profiles and extents are in reasonable agreement with existing experiments.