Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-17T11:23:32.328Z Has data issue: false hasContentIssue false
  • English
  • Français

An experimental study of the motion of a light sphere in a rotating viscous fluid

Published online by Cambridge University Press:  21 May 2018

T. Sauma-Pérez ,
C. G. Johnson ,
L. Yang  and
T. Mullin Open the ORCID record for T. Mullin [Opens in a new window]
T. Sauma-Pérez
Affiliation:
Manchester Centre for Nonlinear Dynamics, School of Physics and Astronomy, University of Manchester, Oxford Road, Manchester M13 9PL, UK
C. G. Johnson
Affiliation:
Manchester Centre for Nonlinear Dynamics and School of Mathematics, University of Manchester, Oxford Road, Manchester M13 9PL, UK
L. Yang
Affiliation:
Manchester Centre for Nonlinear Dynamics, School of Physics and Astronomy, University of Manchester, Oxford Road, Manchester M13 9PL, UK
T. Mullin*
Affiliation:
Mathematical Institute, University of Oxford, Woodstock Road, Oxford OX2 6GG, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We present the results of an experimental investigation of the motion of a light, solid sphere in a horizontal rotating cylinder filled with viscous fluid. At high rotation rates, the sphere sits near the axis of the cylinder. At lower rotation rates, a set of off-axis fixed points are observed for a range of sphere radii. The locations of these fixed points are in quantitative agreement with the predictions of a model based on available theory. The fixed points are observed to become unstable to periodic orbits below a critical Reynolds number 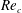$Re_{c}$. The radius of the observed orbits increases with Reynolds number more slowly than a typical Hopf bifurcation, in this case, growing as $1/Re^{2}$.

JFM classification

Low-Reynolds-number flows: Low-Reynolds-number flows Multiphase and Particle-laden Flows: Particle/fluid flow Low-Reynolds-number flows: Stokesian dynamics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 847 , 25 July 2018 , pp. 119 - 133
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ashmore, J., Del Pino, C. & Mullin, T. 2005 Cavitation in a lubrication flow between a moving sphere and a boundary. Phys. Rev. Lett. 94 (12), 124501.CrossRefGoogle Scholar
Basset, A. B. 1888 A Treatise on Hydrodynamics: With Numerous Examples, Vol. 2. Deighton, Bell and Company.Google Scholar
Batchelor, G. K. 1967 An Introduction to Fluid Mechanics. Cambridge University Press.Google Scholar
Bluemink, J. J., Lohse, D., Prosperetti, A. & Van Wijngaarden, L. 2010 Drag and lift forces on particles in a rotating flow. J. Fluid Mech. 643, 1–31.CrossRefGoogle Scholar
Bretherton, F. P. 1962 The motion of rigid particles in a shear flow at low Reynolds number. J. Fluid Mech. 14, 284–304.CrossRefGoogle Scholar
Candelier, F. 2008 Time-dependent force acting on a particle moving arbitrarily in a rotating flow, at small Reynolds and Taylor numbers. J. Fluid Mech. 608, 319–336.Google Scholar
Childress, S. 1964 The slow motion of a sphere in a rotating, viscous fluid. J. Fluid Mech. 20, 305–314.CrossRefGoogle Scholar
Coimbra, C. F. M. & Kobayashi, M. H. 2002 Particle dynamics and pattern formation in a rotating suspension. J. Fluid Mech. 469, 257–286.Google Scholar
Dandy, D. S. & Dwyer, H. A. 1990 A sphere in shear flow at finite Reynolds number: effect of shear on particle lift, drag, and heat transfer. J. Fluid Mech. 216, 381–410.Google Scholar
Davidheiser, J. E., Syers, P., Segre, P. N. & Weeks, E. R. 2010 Complex dynamics of three interacting spheres in a rotating drum. Phys. Fluids 22, 033305.Google Scholar
Ecke, R. E., Zhong, F. & Knobloch, E. 1992 Hopf bifurcation with broken reflection symmetry in rotating Rayleigh–Bénard convection. Eur. Phys. Lett. 19 (3), 177–182.Google Scholar
Gao, H., Ayyaswamy, P. S. & Ducheyne, P. 1996 Dynamics of a microcarrier particle in the simulated microgravity environment of a rotating-wall vessel. Microgravity Sci. Technol. 10 (3), 154–165.Google Scholar
Gotoh, T. 1990 Brownian motion in a rotating flow. J. Stat. Phys. 59 (1–2), 371–402.Google Scholar
Greenspan, H. P. & Howard, L. N. 1963 On a time-dependent motion of a rotating fluid. J. Fluid Mech. 17 (3), 385–404.Google Scholar
Herron, I. H., Davis, S. H. & Bretherton, F. P. 1975 On the sedimentation of a sphere in a centrifuge. J. Fluid Mech. 68 (2), 209–234.Google Scholar
Karanfilian, S. K. & Kotas, T. J. 1981 Motion of a spherical particle in a liquid rotating as a solid body. Proc. R. Soc. Lond. A 376 (1767), 525–544.Google Scholar
Leal, G. 1980 Particle motions in a viscous fluid. Annu. Rev. Fluid Mech. 12, 435–476.Google Scholar
Lee, J. & Ladd, A. J. C. 2005 Particle dynamics and pattern formation in a rotating suspension. J. Fluid Mech. 577, 183–209.CrossRefGoogle Scholar
Magnaudet, J. J. M. 1997 The forces acting on bubbles and rigid particles. In ASME Fluids Engineering Division Summer Meeting, FEDSM, pp. 22–26.Google Scholar
Maxey, M. R. & Riley, J. J. 1983 Equation of motion for a small rigid sphere in a nonuniform flow. Phys. Fluids 26 (4), 883–889.Google Scholar
McLaughlin, J. B. 1991 Inertial migration of a small sphere in linear shear flows. J. Fluid Mech. 224, 261–274.Google Scholar
Mei, R. 1992 An approximate expression for the shear lift force on a spherical particle at finite Reynolds number. Intl J. Multiphase Flow 18 (1), 145–147.Google Scholar
Mukundakrishnan, K., Hu, H. H. & Ayyaswamy, P. S. 2008 The dynamics of two spherical particles in a confined rotating flow: pedalling motion. J. Fluid Mech. 599, 169–204.Google Scholar
Mullin, T., Li, Y., Del Pino, C. & Ashmore, J. 2005 An experimental study of fixed points and chaos in the motion of spheres in a Stokes flow. IMA J. Appl. Maths 70 (5), 666–676.Google Scholar
Pfister, G. & Gerdts, U. 1981 Dynamics of Taylor wavy vortex flow. Phys. Lett. 83A (1), 23–25.Google Scholar
Ramirez, L. E. S., Lim, E. A., Coimbra, C. F. M. & Kobayashi, M. H. 2003 On the dynamics of a spherical scaffold in rotating bioreactors. Biotechnol. Bioengng 84 (3), 382–389.Google Scholar
Rastello, M., Marié, J.-L., Grosjean, N. & Lance, M. 2009 Select drag and lift forces on interface-contaminated bubbles spinning in a rotating flow. J. Fluid Mech. 624, 159–178.Google Scholar
Roberts, G. O., Kornfeld, D. M. & Fowlis, W. W. 1991 Particle orbits in a rotating liquid. J. Fluid Mech. 229, 555–567.Google Scholar
Saffman, P. G. 1965 The lift on a small sphere in a slow shear flow. J. Fluid Mech. 22, 385–400.Google Scholar
Segré, G. & Silberberg, A. 1962 Behaviour of macroscopic rigid spheres in Poiseuille flow. Part 2. Experimental results and interpretation. J. Fluid Mech. 14 (1), 136–157.CrossRefGoogle Scholar
Seiden, G. & Thomas, P. J. 2011 Complexity, segregation, and pattern formation in rotating-drum flows. Rev. Mod. Phys. 83 (4), 1323–1365.Google Scholar
Sreenivasan, K., Strykowski, P. & Olinger, D. 1987 Hopf bifurcation, Landau equation, and vortex shedding behind circular cylinders. In Forum on Unsteady Flow Separation (ed. Ghia, K. N.), vol. 52, pp. 1–13. ASME, Fluids Engineering Division.Google Scholar
Thomson, W. (Lord Kelvin) 1873 On the ultramundane corpuscles of Le Sage. Phil. Mag. 45, 321–332.Google Scholar
Van Nierop, E. A., Luther, S., Bluemink, J. J., Magnaudet, J., Prosperetti, A. & Lohse, D. 2007 Drag and lift forces on bubbles in a rotating flow. J. Fluid Mech. 571, 439–454.Google Scholar
Yang, L., Seddon, J. R. T., Mullin, T., Del Pino, C. & Ashmore, J. 2006 The motion of a rough particle in a Stokes flow adjacent to a boundary. J. Fluid Mech. 557, 337–346.CrossRefGoogle Scholar