Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Giorgio-Serchi, Francesco
and
Weymouth, Gabriel D.
2017.
Can added-mass variation act as a thrust force?.
p.
1.
Bi, Xiaobo
and
Zhu, Qiang
2018.
Numerical investigation of cephalopod-inspired locomotion with intermittent bursts.
Bioinspiration & Biomimetics,
Vol. 13,
Issue. 5,
p.
056005.
Gao, Amy
and
Triantafyllou, Michael S.
2018.
Independent caudal fin actuation enables high energy extraction and control in two-dimensional fish-like group swimming.
Journal of Fluid Mechanics,
Vol. 850,
Issue. ,
p.
304.
Bi, Xiaobo
and
Zhu, Qiang
2019.
Dynamics of a squid-inspired swimmer in free swimming.
Bioinspiration & Biomimetics,
Vol. 15,
Issue. 1,
p.
016005.
Bi, Xiaobo
and
Zhu, Qiang
2019.
Fluid-structure investigation of a squid-inspired swimmer.
Physics of Fluids,
Vol. 31,
Issue. 10,
Bi, Xiaobo
and
Zhu, Qiang
2020.
Pulsed-jet propulsion via shape deformation of an axisymmetric swimmer.
Physics of Fluids,
Vol. 32,
Issue. 8,
Christianson, Caleb
Cui, Yi
Ishida, Michael
Bi, Xiaobo
Zhu, Qiang
Pawlak, Geno
and
Tolley, Michael T
2020.
Cephalopod-inspired robot capable of cyclic jet propulsion through shape change.
Bioinspiration & Biomimetics,
Vol. 16,
Issue. 1,
p.
016014.
Luo, Yang
Xiao, Qing
Zhu, Qiang
and
Pan, Guang
2020.
Pulsed-jet propulsion of a squid-inspired swimmer at high Reynolds number.
Physics of Fluids,
Vol. 32,
Issue. 11,
Hou, T G
Yang, X B
Wang, T M
Liang, J H
Li, S W
and
Fan, Y B
2020.
Locomotor transition: how squid jet from water to air.
Bioinspiration & Biomimetics,
Vol. 15,
Issue. 3,
p.
036014.
Schwab, Fabian
Lunsford, Elias T
Hong, Taehwa
Wiesemüller, Fabian
Kovac, Mirko
Park, Yong-Lae
Akanyeti, Otar
Liao, James C
and
Jusufi, Ardian
2021.
Body Caudal Undulation Measured by Soft Sensors and Emulated by Soft Artificial Muscles.
Integrative and Comparative Biology,
Vol. 61,
Issue. 5,
p.
1955.
Yang, Zhiyuan
Chen, Dongsheng
Levine, David J.
and
Sung, Cynthia
2021.
Origami-Inspired Robot That Swims via Jet Propulsion.
IEEE Robotics and Automation Letters,
Vol. 6,
Issue. 4,
p.
7145.
Wang, Tso-Kang
and
Shoele, Kourosh
2021.
Geometrically weighted modal decomposition techniques.
Journal of Fluid Mechanics,
Vol. 911,
Issue. ,
Ruzicka, M.C.
Puncochar, M.
and
Simcik, M.
2021.
From single bubble to global mixing: Added mass, drift, wake.
Chemical Engineering Research and Design,
Vol. 172,
Issue. ,
p.
280.
Luo, Yang
Xiao, Qing
Zhu, Qiang
and
Pan, Guang
2021.
Jet propulsion of a squid-inspired swimmer in the presence of background flow.
Physics of Fluids,
Vol. 33,
Issue. 3,
Bi, Xiaobo
and
Zhu, Qiang
2021.
Efficiency of pulsed-jet propulsion via thrust-drag decomposition.
Physics of Fluids,
Vol. 33,
Issue. 7,
Hermes, Mark
Ishida, Michael
Luhar, Mitul
and
Tolley, Michael T.
2021.
Bioinspired Sensing, Actuation, and Control in Underwater Soft Robotic Systems.
p.
7.
Bi, Xiaobo
and
Zhu, Qiang
2022.
Role of internal flow in squid-inspired jet propulsion.
Physics of Fluids,
Vol. 34,
Issue. 3,
Bi, Xiaobo
Tang, Hui
and
Zhu, Qiang
2022.
Feasibility of hydrodynamically activated valves for salp-like propulsion.
Physics of Fluids,
Vol. 34,
Issue. 10,
Zhu, Qiang
and
Xiao, Qing
2022.
Physics and applications of squid-inspired jetting.
Bioinspiration & Biomimetics,
Vol. 17,
Issue. 4,
p.
041001.
Paniccia, Damiano
Graziani, Giorgio
Lugni, Claudio
and
Piva, Renzo
2022.
The fish ability to accelerate and suddenly turn in fast maneuvers.
Scientific Reports,
Vol. 12,
Issue. 1,
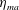 $\unicode[STIX]{x1D702}_{ma}$, which measures momentum recovery in relation to the maximum momentum recovery possible in an ideal flow, increases with increasing the non-dimensional shrinking parameter
$\unicode[STIX]{x1D702}_{ma}$, which measures momentum recovery in relation to the maximum momentum recovery possible in an ideal flow, increases with increasing the non-dimensional shrinking parameter 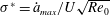 $\unicode[STIX]{x1D70E}^{\ast }={\dot{a}}_{max}/U\sqrt{\mathit{Re }_{0}}$, where
$\unicode[STIX]{x1D70E}^{\ast }={\dot{a}}_{max}/U\sqrt{\mathit{Re }_{0}}$, where 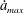 ${\dot{a}}_{max}$ is the maximum shrinking speed,
${\dot{a}}_{max}$ is the maximum shrinking speed, 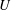 $U$ is the characteristic flow velocity and
$U$ is the characteristic flow velocity and 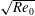 $\sqrt{\mathit{Re }_{0}}$ is the Reynolds number at the beginning of the shrinking motion. An estimated threshold
$\sqrt{\mathit{Re }_{0}}$ is the Reynolds number at the beginning of the shrinking motion. An estimated threshold 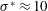 $\unicode[STIX]{x1D70E}^{\ast }\approx 10$ determines whether or not enough energy is recovered to the body to produce net thrust. Since there is a region of high transition for
$\unicode[STIX]{x1D70E}^{\ast }\approx 10$ determines whether or not enough energy is recovered to the body to produce net thrust. Since there is a region of high transition for 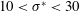 $10<\unicode[STIX]{x1D70E}^{\ast }<30$ where the recovery performance varies widely and for
$10<\unicode[STIX]{x1D70E}^{\ast }<30$ where the recovery performance varies widely and for  $\unicode[STIX]{x1D70E}^{\ast }>100$ added mass energy is recovered at diminishing returns, we propose a design criterion for shrinking bodies to be in the range of
$\unicode[STIX]{x1D70E}^{\ast }>100$ added mass energy is recovered at diminishing returns, we propose a design criterion for shrinking bodies to be in the range of 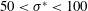 $50<\unicode[STIX]{x1D70E}^{\ast }<100$, resulting in 61–82 % energy recovery.
$50<\unicode[STIX]{x1D70E}^{\ast }<100$, resulting in 61–82 % energy recovery.