Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-23T05:22:47.186Z Has data issue: false hasContentIssue false
  • English
  • Français

A useful ageing property based on the Laplace transform

Published online by Cambridge University Press:  14 July 2016

Bengt Klefsjö
Bengt Klefsjö*
Affiliation:
University of Luleå
*
∗ Postal address: Department of Mathematics, University of Luleå, S-95187 Luleå, Sweden.
Get access Rights & Permissions [Opens in a new window]

Abstract

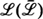The class of life distributions for which , where 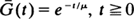, and 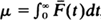, is studied. We prove that this class is larger than the HNBUE (HNWUE) class (consisting of those life distributions for which 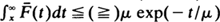for x ≧ 0) and present results concerning closure properties under some usual reliability operations. We also study some shock models and a certain cumulative damage model. The class 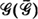of discrete life distributions for which for 0 ≦ p ≦ 1, where 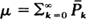, is also studied.

Keywords

LIFE DISTRIBUTIONSURVIVAL FUNCTIONHNBUEHNWUELAPLACE TRANSFORMSHOCK MODELPOISSON PROCESSBIRTH PROCESSDAMAGE MODEL
Type
Research Papers
Information
Journal of Applied Probability , Volume 20 , Issue 3 , September 1983 , pp. 615 - 626
Copyright
Copyright © Applied Probability Trust 1983 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

A-Hameed, M. S. and Proschan, F. (1973) Nonstationary shock models. Stoch. Proc. Appl. 1, 383–404.Google Scholar
A-Hameed, M. S. and Proschan, F. (1975) Shock models with underlying birth processes. J. Appl. Prob. 12, 18–28.Google Scholar
Barlow, R. E. and Proschan, F. (1975) Statistical Theory of Reliability and Life Testing. Probability Models. Holt, Rinehart and Winston, New York.Google Scholar
Block, H. W. and Savits, T. H. (1978) Shock models with NBUE survival. J. Appl. Prob. 15, 621–628.Google Scholar
Block, H. W. and Savits, T. H. (1980) Laplace transforms for classes of life distributions. Ann. Prob. 8, 465–474.CrossRefGoogle Scholar
Bondesson, L. (1981) On preservation of classes of life distributions under reliability operations; some complementary results. Research Report 1981–3, Department of Mathematical Statistics, University of Umeå.Google Scholar
Bryson, M. C. and Siddiqui, M. M. (1969) Some criteria for aging. J. Amer. Statist. Soc. 64, 1472–1483.Google Scholar
Esary, J. D., Marshall, A. W. and Proschan, F. (1973) Shock models and wear processes. Ann. Prob. 1, 627–649.Google Scholar
Feller, W. (1968) An Introduction to Probability Theory and its Applications, Vol. I, 3rd edn. Wiley, New York.Google Scholar
Feller, W. (1971) An Introduction to Probability Theory and its Applications, Vol. II, 2nd edn. Wiley, New York.Google Scholar
Haines, A. (1973) Some Contributions to the Theory of Restricted Classes of Distributions with Applications to Reliability. Ph.D. Dissertation, The George Washington University.Google Scholar
Klefsjö, B. (1981) HNBUE survival under some shock models. Scand. J. Statist. 8, 39–47.Google Scholar
Klefsjö, B. (1982) The HNBUE and HNWUE classes of life distributions. Naval Res. Logist. Quart. 29, 331–344.Google Scholar
Rolski, T. (1975) Mean residual life. Bull. Internat. Statist. Inst. 46, 266–270.Google Scholar
Rolski, T. and Stoyan, D. (1976) On the comparison of waiting times in GI/G/I queues. Operat. Res. 24, 197–200.Google Scholar
Runnenberg, J. T. (1965) On the use of the method of collective marks in queueing theory. In Proc. Symp. Congestion Theory, ed. Smith, W. L. and Wilkinson, W. E., University of North Carolina Press, Chapel Hill, 399–418.Google Scholar
Råde, L. (1972a) On the use of generating functions and Laplace transforms in applied probability theory. Internat. J. Math. Educ. Sci. Technol. 3, 25–33.Google Scholar
Råde, L. (1972b) Thinning of Renewal Point Processes. Matematisk Statistik AB, Gothenburg, Sweden.Google Scholar
Råde, L. (1974) A reliability problem treated by the additional event method. Microelectronics and Reliability 13, 483–485.CrossRefGoogle Scholar