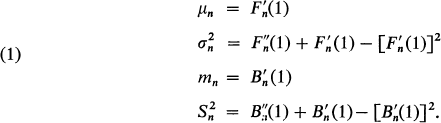We consider a population which evolves at discrete points in time by branching and immigration, and in which each member reproduces independently of all others. Let Fn(x) denote the probability generating function (P.G.F.) of the number of offspring produced by each member of the nth generation, Bn–1(x) the P.G.F. of the number of immigrants joining the nth generation and Zn the population size in the nth generation. We write