Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-23T05:51:58.015Z Has data issue: false hasContentIssue false
  • English
  • Français

The substability and ergodicity of complicated queueing systems

Published online by Cambridge University Press:  14 July 2016

Toshinao Nakatsuka
Toshinao Nakatsuka*
Affiliation:
Tokyo Metropolitan University
*
∗Postal address: Faculty of Economics, Tokyo Metropolitan University, 1–1-1 Yakumo, Meguroku, Tokyo, Japan.
Get access Rights & Permissions [Opens in a new window]

Abstract

The substability and the ergodicity of various queueing models are discussed. This paper considers the vector-valued queueing process Xrn = (xrn,1,xrn,2, · ··) with non-negative components and a constant initial value Xrr= a. For this, the substability is derived under simple conditions by showing the finiteness of 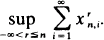With respect to the ergodicity, in order to make use of Borovkov's theorem, we additionally assume that the distribution of the interarrival of customers has non-bounded tail for any given past sequence.

Keywords

DISTRIBUTION WITH LONG TAILLIFOFINITE-SIZE WAITING ROOMRENEGING TIME
Type
Research Papers
Information
Journal of Applied Probability , Volume 23 , Issue 1 , March 1986 , pp. 193 - 200
Copyright
Copyright © Applied Probability Trust 1986 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1] Borovkov, A. A. (1976) Stochastic Processes in Queueing Theory. Springer-Verlag, New York.Google Scholar
[2] Borovkov, A. A. (1978) Ergodicity and stability theorems for a class of stochastic equations and their applications. Theory Prob. Appl. 23, 227–247.CrossRefGoogle Scholar
[3] Franken, F., König, D., Arndt, U. and Schmidt, V. (1982) Queues and Point Processes. Wiley, New York.Google Scholar
[4] Kalähne, U. (1976) Existence, uniqueness and some invariance properties of stationary distributions for general single server queue. Math. Operationsforch. u. Statist. 7, 557–575.Google Scholar
[5] Loynes, R. M. (1962) The stability of a queue with non-independent inter-arrival and service times. Proc. Camb. Phil. Soc. 58, 497–520.CrossRefGoogle Scholar
[6] Miyazawa, M. (1977) Time and customer processes in queues with stationary input. J. Appl. Prob. 14, 349–357.Google Scholar
[7] Nakatsuka, T. (1982) Stability of the critical queueing systems. J. Operat. Res. Soc. Japan 25, 140–150.Google Scholar