Published online by Cambridge University Press: 02 May 2023
We consider a stochastic SIR (susceptible 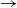 $\rightarrow$ infective
$\rightarrow$ infective 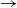 $\rightarrow$ removed) model in which the infectious periods are modulated by a collection of independent and identically distributed Feller processes. Each infected individual is associated with one of these processes, the trajectories of which determine the duration of his infectious period, his contamination rate, and his type of removal (e.g. death or immunization). We use a martingale approach to derive the distribution of the final epidemic size and severity for this model and provide some general examples. Next, we focus on a single infected individual facing a given number of susceptibles, and we determine the distribution of his outcome (number of contaminations, severity, type of removal). Using a discrete-time formulation of the model, we show that this distribution also provides us with an alternative, more stable method to compute the final epidemic outcome distribution.
$\rightarrow$ removed) model in which the infectious periods are modulated by a collection of independent and identically distributed Feller processes. Each infected individual is associated with one of these processes, the trajectories of which determine the duration of his infectious period, his contamination rate, and his type of removal (e.g. death or immunization). We use a martingale approach to derive the distribution of the final epidemic size and severity for this model and provide some general examples. Next, we focus on a single infected individual facing a given number of susceptibles, and we determine the distribution of his outcome (number of contaminations, severity, type of removal). Using a discrete-time formulation of the model, we show that this distribution also provides us with an alternative, more stable method to compute the final epidemic outcome distribution.