Article contents
Scheduled maxima sequences
Published online by Cambridge University Press: 14 July 2016
Abstract
We define a vector-valued scheduled maxima sequence M by considering simultaneously the maxima of several i.i.d. sequences, with the number of observations considered from each sequence at any time determined by a random scheduling sequence J. It is shown that the max-min (vector) sequence 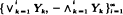 derived from i.i.d.
derived from i.i.d. 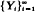 can be represented as a mixture of scheduled maxima sequences, giving results for this sequence and the range
can be represented as a mixture of scheduled maxima sequences, giving results for this sequence and the range 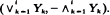 A functional limit theorem for the scheduled maxima sequence shows convergence to independent extremal processes. Embedding in a scheduled extremal process gives strong laws, central limit theorems, and laws of the iterated logarithm for the record time of the scheduled maxima sequence, and hence for the max-min sequence and the range.
A functional limit theorem for the scheduled maxima sequence shows convergence to independent extremal processes. Embedding in a scheduled extremal process gives strong laws, central limit theorems, and laws of the iterated logarithm for the record time of the scheduled maxima sequence, and hence for the max-min sequence and the range.
Keywords
- Type
- Research Papers
- Information
- Copyright
- Copyright © Applied Probability Trust 1978
References
- 6
- Cited by


