Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-22T08:56:48.807Z Has data issue: false hasContentIssue false
  • English
  • Français

Records for time-dependent stationary Gaussian sequences

Part of: Stochastic processes

Published online by Cambridge University Press:  04 May 2020

Michael Falk ,
Amir Khorrami Chokami  and
Simone A. Padoan
Michael Falk*
Affiliation:
University of Würzburg
Amir Khorrami Chokami*
Affiliation:
Polytechnic of Turin
Simone A. Padoan*
Affiliation:
Bocconi University
*
*Postal address: Institute of Mathematics, University of Würzburg, Am Hubland, D-97074, Würzburg, Germany. Email address: [email protected]
**Postal address: Department of Mathematical Sciences, Polytechnic of Turin, Corso Duca degli Abruzzi 24, 10129, Turin, Italy. Email address: [email protected]
***Postal address: Department of Decision Sciences, Bocconi University of Milan, via Roentgen 1, 20136 Milano, Italy. Email address: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

For a zero-mean, unit-variance stationary univariate Gaussian process we derive the probability that a record at the time n, say $X_n$ , takes place, and derive its distribution function. We study the joint distribution of the arrival time process of records and the distribution of the increments between records. We compute the expected number of records. 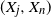We also consider two consecutive and non-consecutive records, one at time j and one at time n, and we derive the probability that the joint records $(X_j,X_n)$ occur, as well as their distribution function. The probability that the records 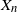$X_n$ and $(X_j,X_n)$ take place and the arrival time of the nth record are independent of the marginal distribution function, provided that it is continuous. These results actually hold for a strictly stationary process with Gaussian copulas.

Keywords

Arrival timeclosed skew-normal distributionGaussian processincrementsjoint recordsrecordstrictly stationary process

MSC classification

Primary: 60G15: Gaussian processes
Secondary: 60G10: Stationary processes 60G40: Stopping times; optimal stopping problems; gambling theory 60G70: Extreme value theory; extremal processes
Type
Research Papers
Information
Journal of Applied Probability , Volume 57 , Issue 1 , March 2020 , pp. 78 - 96
Copyright
© Applied Probability Trust 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anderson, T. W. (1984). An Introduction to Multivariate Statistical Analysis, 2nd edn (Wiley Series in Probability and Mathematical Statistics: Probability and Mathematical Statistics). John Wiley, New York.Google Scholar
Arnold, B. C., Balakrishnan, N. and Nagaraja, H. N. (1998). Records (Wiley Series inProbability and Statistics). John Wiley, New York.Google Scholar
Azzalini, A. (2013). The Skew-Normal and Related Families. Cambridge University Press.CrossRefGoogle Scholar
Azzalini, A. and Bacchieri, A. (2010). A prospective combination of phase ii and phase iii in drug development. Metron 68, 347–369.10.1007/BF03263544CrossRefGoogle Scholar
Azzalini, A. and Valle, A. D. (1996). The multivariate skew-normal distribution. Biometrika 83, 715–726.CrossRefGoogle Scholar
Barakat, H. and Elgawad, M. A. (2017). Asymptotic behavior of the joint record values, with applications. Statist. Prob. Lett. 124, 13–21.10.1016/j.spl.2016.12.020CrossRefGoogle Scholar
Basrak, B., Planini, H. and Soulier, P. (2018). An invariance principle for sums and record times of regularly varying stationary sequences. Prob. Theory Relat. Fields 172, 869–914.10.1007/s00440-017-0822-9CrossRefGoogle Scholar
Brockwell, P. J. and Davis, R. A. (2013). Time Series: Theory and Methods. Springer, New York.Google Scholar
Falk, M., Khorrami Chokami, A. and Padoan, S. A. (2018a). On multivariate records from random vectors with independent components. J. Appl. Prob. 55, 43–53.10.1017/jpr.2018.4CrossRefGoogle Scholar
Falk, M., Khorrami Chokami, A. and Padoan, S. A. (2018b). Some results on joint record events. Statist. Prob. Lett. 135, 11–19.10.1016/j.spl.2017.11.011CrossRefGoogle Scholar
Galambos, J. (1987). The Asymptotic Theory of Extreme Order Statistics, 2nd edn. Krieger, Malabar.Google Scholar
Genton, M. G. (2004). Skew-Elliptical Distributions and their Applications: A Journey beyond Normality. Wiley CRC Press, Boca Raton.10.1201/9780203492000CrossRefGoogle Scholar
Goldie, C. M. and Resnick, S. I. (1989). Records in a partially ordered set. Ann. Prob. 17, 678–699.10.1214/aop/1176991421CrossRefGoogle Scholar
Haiman, G. (1987). Almost sure asymptotic behavior of the record and record time sequences of a stationary Gaussian process. In Mathematical Statistics and Probability Theory, pp. 105–120. Springer, New York.10.1007/978-94-009-3963-9_10CrossRefGoogle Scholar
Haiman, G., Mayeur, N., Nevzorov, V. and Puri, M. L. (1998). Records and 2-block records of 1-dependent stationary sequences under local dependence. Ann. Inst. H. Poincaré Prob. Statist. 34, 481–503.10.1016/S0246-0203(98)80025-3CrossRefGoogle Scholar
Hsing, T., Hüsler, J. and Reiss, R.-D. (1992). The extremes of a triangular array of normal random variables. Ann. Appl. Prob. 6, 671–686.Google Scholar
Resnick, S. I. (2008). Extreme Values, Regular Variation, and Point Processes (Springer Series in Operations Research and Financial Engineering). Springer, New York.Google Scholar