Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-23T05:10:12.072Z Has data issue: false hasContentIssue false
  • English
  • Français

A rate of convergence result for the super-critical Galton-Watson process

Published online by Cambridge University Press:  14 July 2016

C. C. Heyde
C. C. Heyde*
Affiliation:
Australian National University
Get access Rights & Permissions [Opens in a new window]

Extract

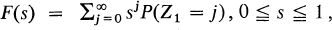Let Z0 = 1, Z1, Z2, ··· denote a super-critical Galton-Watson process whose non-degenerate offspring distribution has probability generating function where 1 < m = EZ1 < ∞. The Galton-Watson process evolves in such a way that the generating function Fn(s) of Znis the nth functional iterate of F(s). The convergence problem for Zn, when appropriately normed, has been studied by quite a number of authors; for an ultimate form see Heyde [2]. However, no information has previously been obtained on the rate of such convergence. We shall here suppose that in which case Wn = m –nZn converges almost surely to a non-degenerate random variable W as n → ∞ (Harris [1], p. 13). It is our object to establish the following result on the rate of convergence of Wn to W.

Type
Short Communications
Information
Journal of Applied Probability , Volume 7 , Issue 2 , August 1970 , pp. 451 - 454
Copyright
Copyright © Applied Probability Trust 1970 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1] Harris, T. E. (1963) The Theory of Branching Processes. Springer-Verlag, Berlin.Google Scholar
[2] Heyde, C. C. (1970) Extension of a result of Seneta for the super-critical Galton-Watson process. Ann. Math. Statist. 41 (in press).CrossRefGoogle Scholar