Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-16T22:23:15.205Z Has data issue: false hasContentIssue false
  • English
  • Français

Oscillations for order statistics of some discrete processes

Part of: Limit theorems Stochastic processes

Published online by Cambridge University Press:  04 September 2020

Andrea Ottolini
Andrea Ottolini*
Affiliation:
Stanford University
*
*Postal address: 450 Jane Stanford Way, Building 380, Stanford, CA94305-2125, USA. Email: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Suppose k balls are dropped into n boxes independently with uniform probability, where n, k are large with ratio approximately equal to some positive real 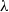$\lambda$ . The maximum box count has a counterintuitive behavior: first of all, with high probability it takes at most two values 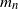$m_n$ or 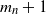$m_n+1$ , where 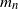$m_n$ is roughly 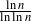$\frac{\ln n}{\ln \ln n}$ . Moreover, it oscillates between these two values with an unusual periodicity. In order to prove this statement and various generalizations, it is first shown that for $X_1,\ldots,X_n$ independent and identically distributed discrete random variables with common distribution F, under mild conditions, the limiting distribution of their maximum oscillates in three possible families, depending on the tail of the distribution. The result stated at the beginning follows from the ensemble equivalence for the order statistics in various allocations problems, obtained via conditioning limit theory. Results about the number of ties for the maximum, as well as applications, are also provided.

Keywords

Extreme value theoryrandom allocationsconditioning limit theory

MSC classification

Primary: 60G70: Extreme value theory; extremal processes
Secondary: 60F99: None of the above, but in this section
Type
Research Papers
Information
Journal of Applied Probability , Volume 57 , Issue 3 , September 2020 , pp. 703 - 719
Check for updates
Copyright
© Applied Probability Trust 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

D’Aristotile, A., Diaconis, P. andFreedman, D. (1988). On merging of probabilities. Sankhā A 50, 363–380.Google Scholar
Aldous, D. J. andDiaconis, P. (1995). Hammersley’s interacting particle process and longest increasing subsequences. Prob. Theory Relat. Fields 103, 199–213.CrossRefGoogle Scholar
Anderson, C. W. (1970). Extreme value theory for a class of discrete distributions with applications. J. Appl. Prob. 7, 99–113.CrossRefGoogle Scholar
Anderson, G. W., Guionnet, A. andZeitouni, O. (2006). An Introduction to Random Matrices. Cambridge University Press.Google Scholar
Arratia, R., Barbour, D. andTavaré, S. (2000). Limits of logarithmic combinatorial structures. Ann. Prob. 28, 1620–1644.10.1214/aop/1019160500CrossRefGoogle Scholar
Athreya, J. S. andSethuraman, S. (2001). On the asymptotics of discrete order statistics. Statist. Prob. Lett. 54, 243–249.Google Scholar
Briggs, K., Song, L. andPrellberg, T. (2003 ). A note on the distribution of the maximum of a set of Poisson random variables. Preprint, .Google Scholar
Campenhout, J. M. V. andCover, T. M. (1981). Maximum entropy and conditional probability. IEEE Trans. Inf. Theory 27, 483–489.CrossRefGoogle Scholar
Chatterjee, S., Diaconis, P. andMeckes, E. (2005). Exchangeable pairs and Poisson approximation. Prob. Surv. 2, 64–106.CrossRefGoogle Scholar
Corless, R. M., Gonnet, G. H., Hare, D. E. G., Jeffrey, D. J. andKnuth, D. E. (1996). On the Lambert W function. Adv. Comp. Math. 5, 329–359.CrossRefGoogle Scholar
de Haan, L. andFerreira, A. F. (2006). Extreme Value Theory: An Introduction. Springer, New York.10.1007/0-387-34471-3CrossRefGoogle Scholar
Diaconis, P. andHolmes, S. (2002). A Bayesian peek into Feller Volume I. Sankhyā A 64, 820–841.Google Scholar
Galambos, J. (1987). The Asymptotic Theory of Extreme Order Statistics. Krieger Publishing Co., Melbourne, FL.Google Scholar
Gnedenko, B. V. (1943). Sur la distribution limite du terme maximum d’une série aléatoire. Ann. Math. 44, 423–453.CrossRefGoogle Scholar
Gnedenko, B. V. andKolmogorov, A. N. (1954). Limit Distributions for Sums of Independent Random Variables. Addison-Wesley, Boston, MA (translated from Russian).Google Scholar
Greenhough, J. andMain, I. G. (2007 ). A Poisson model for earthquake frequency uncertainties in seismic hazard analysis. Preprint, CrossRefGoogle Scholar
Kimber, A. C. (1983). A note on Poisson maxima. Z. Wahrscheinlichkeitsth. 63, 551–552.CrossRefGoogle Scholar
Sethuraman, S. (2003). A clustering law for some discrete order statistics. J. Appl. Prob. 40, 226–241.CrossRefGoogle Scholar
Sheep, L. A. andLloyd, S. P. (1966). Ordered cycle lengths in a random permutation. Trans. Amer. Math. Soc. 121, 340–357.CrossRefGoogle Scholar
Geological Survey, US. (2020). Earthquake hazards program. Available at https://earthquake.usgs.gov/.Google Scholar
Zabell, S. L. (1980). Rates of convergence for conditional expectations. Ann. Prob. 8, 928–941.CrossRefGoogle Scholar