Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-23T18:12:58.716Z Has data issue: false hasContentIssue false
  • English
  • Français

On the continuity of Pickands constants

Part of: Stochastic processes

Published online by Cambridge University Press:  18 January 2022

Krzysztof Dębicki ,
Enkelejd Hashorva  and
Zbigniew Michna
Krzysztof Dębicki*
Affiliation:
University of Wrocław
Enkelejd Hashorva*
Affiliation:
University of Lausanne
Zbigniew Michna*
Affiliation:
Wrocław University of Science and Technology
*
*Postal address: Mathematical Institute, University of Wrocław, pl. Grunwaldzki 2/4, 50-384 Wrocław, Poland. Email address: [email protected]
**Postal address: Department of Actuarial Science, University of Lausanne, UNIL-Dorigny, 1015 Lausanne, Switzerland. Email address: [email protected]
***Postal address: Department of Operations Research and Business Intelligence, Wrocław University of Science and Technology, wybrzeze Stanislawa Wyspianskiego 27, 50-370 Wrocław, Poland. Article partially completed at Wrocław University of Economics and Business. Email address: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

For a non-negative separable random field Z(t), 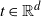$t\in \mathbb{R}^d$ , satisfying some mild assumptions, we show that 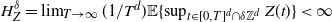$ H_Z^\delta =\lim_{{T} \to \infty} ({1}/{T^d}) \mathbb{E}\{{\sup_{ t\in [0,T]^d \cap \delta \mathbb{Z}^d } Z(t) }\} <\infty$ for 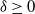$\delta \ge 0$ , where 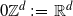$0 \mathbb{Z}^d\,:\!=\,\mathbb{R}^d$ , and prove that $H_Z^0$ can be approximated by 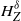$H_Z^\delta$ if 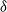$\delta$ tends to 0. These results extend the classical findings for Pickands constants 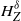$H_{Z}^\delta$ , defined for 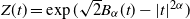$Z(t)= \exp( \sqrt{ 2} B_\alpha (t)- \lvert {t} \rvert^{2\alpha })$ , 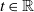$t\in \mathbb{R}$ , with $B_\alpha$ a standard fractional Brownian motion with Hurst parameter $\alpha \in (0,1]$ . The continuity of 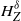$H_{Z}^\delta$ at 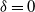$\delta=0$ is additionally shown for two particular extensions of Pickands constants.

Keywords

Pickands constantsdiscrete approximationlocally stationary Gaussian random fieldsmax-stable random fieldsextremal index

MSC classification

Primary: 60G10: Stationary processes
Secondary: 60G70: Extreme value theory; extremal processes
Type
Original Article
Information
Journal of Applied Probability , Volume 59 , Issue 1 , March 2022 , pp. 187 - 201
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Applied Probability Trust

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Basrak, B. and Planinić, H. (2021). Compound Poisson approximation for random fields with application to sequence alignment. Bernoulli 27, 1371–1408.10.3150/20-BEJ1278CrossRefGoogle Scholar
Berman, S. (1992). Sojourns and Extremes of Stochastic Processes (The Wadsworth & Brooks/Cole Statistics/Probability Series). Wadsworth & Brooks/Cole Advanced Books & Software, Pacific Grove, CA.Google Scholar
Bickel, P. J. and Rosenblatt, M. (1973). On some global measures of the deviations of density function estimates. Ann. Statist. 1, 1071–1095.10.1214/aos/1176342558CrossRefGoogle Scholar
Bladt, M., Hashorva, E., and Shevchenko, G. (2021). Tail measures and regular variation. Available at arXiv:2103.04396v2.Google Scholar
Chan, H. P. and Lai, T. L. (2006). Maxima of asymptotically Gaussian random fields and moderate deviation approximations to boundary crossing probabilities of sums of random variables with multidimensional indices. Ann. Prob. 34, 80–121.10.1214/009117905000000378CrossRefGoogle Scholar
Dębicki, K. (2002). Ruin probability for Gaussian integrated processes. Stoch. Process. Appl. 98, 151–174.10.1016/S0304-4149(01)00143-0CrossRefGoogle Scholar
Dębicki, K. (2005). Some properties of generalized Pickands constants. Teor. Veroyatn. Primen. 50, 396–404.CrossRefGoogle Scholar
Dębicki, K. and Hashorva, E. (2017). On extremal index of max-stable processes. Prob. Math. Statist. 27, 299–317.Google Scholar
Dębicki, K. and Hashorva, E. (2020). Approximation of supremum of max-stable stationary processes and Pickands constants. J. Theoret. Prob. 33, 444–464.10.1007/s10959-018-00876-8CrossRefGoogle Scholar
Dębicki, K. and Liu, P. (2018). Extremes of nonstationary Gaussian fluid queues. Adv. Appl. Prob. 50, 887–917.10.1017/apr.2018.40CrossRefGoogle Scholar
Dębicki, K., Engelke, S. and Hashorva, E. (2017). Generalized Pickands constants and stationary max-stable processes. Extremes 20, 493–517.CrossRefGoogle Scholar
Dieker, A. B. and Mikosch, T. (2015). Exact simulation of Brown–Resnick random fields at a finite number of locations. Extremes 18, 301–314.10.1007/s10687-015-0214-4CrossRefGoogle Scholar
Dieker, A. B. and Yakir, B. (2014). On asymptotic constants in the theory of extremes for Gaussian processes. Bernoulli 20, 1600–1619.CrossRefGoogle Scholar
Dombry, C. and Kabluchko, Z. (2017). Ergodic decompositions of stationary max-stable processes in terms of their spectral functions. Stoch. Process. Appl. 127, 1763–1784.10.1016/j.spa.2016.10.001CrossRefGoogle Scholar
Dombry, C, Hashorva, E. and Soulier, P. (2018). Tail measure and spectral tail process of regularly varying time series. Ann. Appl. Prob. 28, 3884–3921.10.1214/18-AAP1410CrossRefGoogle Scholar
Doob, J. L. (1990). Stochastic Processes (Wiley Classics Library). John Wiley.Google Scholar
Ehlert, A. and Schlather, M. (2008). Capturing the multivariate extremal index: bounds and interconnections. Extremes 11, 353–377.10.1007/s10687-008-0062-6CrossRefGoogle Scholar
de Haan, L. (1984). A spectral representation for max-stable processes. Ann. Prob. 12, 1194–1204.Google Scholar
Hashorva, E. (2018). Representations of max-stable processes via exponential tilting. Stoch. Process. Appl. 128, 2952–2978.10.1016/j.spa.2017.10.003CrossRefGoogle Scholar
Hashorva, E. (2021). On extremal index of max-stable random fields. Lith. Math. J. 61, 217–238.CrossRefGoogle Scholar
Janson, S. (2020). The space D in several variables: random variables and higher moments. Available at arXiv:2004.00237.Google Scholar
Kabluchko, Z. (2009). Spectral representations of sum- and max-stable processes. Extremes 12, 401–424.10.1007/s10687-009-0083-9CrossRefGoogle Scholar
Kabluchko, Z. (2010). Stationary systems of Gaussian processes. Ann. Appl. Prob. 20, 2295–2317.10.1214/10-AAP686CrossRefGoogle Scholar
Kabluchko, Z., Schlather, M. and de Haan, L. (2009). Stationary max-stable fields associated to negative definite functions. Ann. Prob. 37, 2042–2065.10.1214/09-AOP455CrossRefGoogle Scholar
Kulik, R. and Soulier, P. (2020). Heavy Tailed Time Series. Springer, Cham.10.1007/978-1-0716-0737-4CrossRefGoogle Scholar
Pickands, J. III (1969). Upcrossing probabilities for stationary Gaussian processes. Trans. Amer. Math. Soc. 145, 51–73.10.1090/S0002-9947-1969-0250367-XCrossRefGoogle Scholar
Pickands, J. III (1969). Asymptotic properties of the maximum in a stationary Gaussian process. Trans. Amer. Math. Soc. 145, 75–86.Google Scholar
Piterbarg, V. I. (1996). Asymptotic Methods in the Theory of Gaussian Processes and Fields (Translations of Mathematical Monographs 148). American Mathematical Society, Providence, RI.Google Scholar
Piterbarg, V. I. (2015). Twenty Lectures About Gaussian Processes. Atlantic Financial Press, London.Google Scholar
Planinić, H. and Soulier, P. (2018). The tail process revisited. Extremes 21, 551–579.10.1007/s10687-018-0312-1CrossRefGoogle Scholar
Potthoff, J. (2009). Sample properties of random fields I: Separability and measurability. Commun. Stoch. Anal. 3, 143–153.Google Scholar
Roy, P. (2010). Ergodic theory, abelian groups and point processes induced by stable random fields. Ann. Prob. 38, 770–793.CrossRefGoogle Scholar
Roy, P. (2010). Nonsingular group actions and stationary $S\alpha S$ random fields. Proc. Amer. Math. Soc. 138, 2195–2202.10.1090/S0002-9939-10-10250-0CrossRefGoogle Scholar
Roy, P. and Samorodnitsky, G. (2008). Stationary symmetric $\alpha$ -stable discrete parameter random fields. J. Theoret. Prob. 21, 212–233.10.1007/s10959-007-0107-9CrossRefGoogle Scholar
Samorodnitsky, G. (2004). Extreme value theory, ergodic theory and the boundary between short memory and long memory for stationary stable processes. Ann. Prob. 32, 1438–1468.10.1214/009117904000000261CrossRefGoogle Scholar
Samorodnitsky, G. (2004). Maxima of continuous-time stationary stable processes. Adv. Appl. Prob. 36, 805–823.CrossRefGoogle Scholar
Soulier, P. (2021). The tail process and tail measure of continuous time regularly varying stochastic processes. Extremes, in press. Available at https://doi.org/10.1007/s10687-021-00417-3.CrossRefGoogle Scholar
Varadarajan, V. S. (1958). On a problem in measure-spaces. Ann. Math. Statist. 29, 1275–1278.10.1214/aoms/1177706461CrossRefGoogle Scholar
Vitale, R. A. (1996). The Wills functional and Gaussian processes. Ann. Prob. 24, 2172–2178.10.1214/aop/1041903224CrossRefGoogle Scholar
Wang, Y., Roy, P. and Stoev, S. A. (2013). Ergodic properties of sum- and max-stable stationary random fields via null and positive group actions. Ann. Prob. 41, 206–228.10.1214/11-AOP732CrossRefGoogle Scholar
Wu, L. and Samorodnitsky, G. (2020). Regularly varying random fields. Stoch. Process. Appl. 130, 4470–4492.10.1016/j.spa.2020.01.005CrossRefGoogle Scholar
Yakir, B. (2013). Extremes in Random Fields: A Theory and its Applications (Wiley Series in Probability and Statistics). John Wiley, Chichester, and Higher Education Press, Beijing.Google Scholar