Article contents
On limiting behavior of ordinary and conditional first-passage times for a class of birth-death processes
Published online by Cambridge University Press: 14 July 2016
Abstract
Let N(t) be a birth-death process on 𝒩= {0, 1, 2, ·· ·} governed by the transition rates λn > 0 (n ≧ 0) and μn > 0 (n ≧ 1) where λn → λ > 0 and μn → μ > 0 as n → ∞ and ρ = λ/μ. Let Tmn be the first-passage time of N(t) from m to n and define  It is shown that, when
It is shown that, when 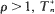 converges in distribution to TBP(μ,λ) as n → ∞ where TΒΡ (μ,λ) is the server busy period of an M/M/1 queueing system with arrival rate μ and service rate λ. Correspondingly T0n/E[T0n] converges to 1 with probability 1 as n →∞. Of related interest is the conditional first-passage time mTrn of N(t) from r to n given no visit to m where m < r < n. As we shall see, the conditional first-passage time of N(t) can be viewed as an ordinary first-passage time of a modified birth-death process M(t) governed by
converges in distribution to TBP(μ,λ) as n → ∞ where TΒΡ (μ,λ) is the server busy period of an M/M/1 queueing system with arrival rate μ and service rate λ. Correspondingly T0n/E[T0n] converges to 1 with probability 1 as n →∞. Of related interest is the conditional first-passage time mTrn of N(t) from r to n given no visit to m where m < r < n. As we shall see, the conditional first-passage time of N(t) can be viewed as an ordinary first-passage time of a modified birth-death process M(t) governed by 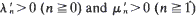 where
where 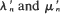 are generated from λn and μn. Furthermore it is shown that for
are generated from λn and μn. Furthermore it is shown that for 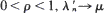 and
and 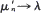 while for
while for 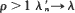 and
and 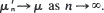 This enables one to establish the relation between the limiting behavior of the ordinary first-passage times and that of the conditional first-passage times.
This enables one to establish the relation between the limiting behavior of the ordinary first-passage times and that of the conditional first-passage times.
Keywords
- Type
- Short Communications
- Information
- Copyright
- Copyright © Applied Probability Trust 1987
References
- 4
- Cited by


