No CrossRef data available.
Published online by Cambridge University Press: 07 December 2022
Let
 $n\geq 2$
random lines intersect a planar convex domain D. Consider the probabilities
$n\geq 2$
random lines intersect a planar convex domain D. Consider the probabilities
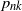 $p_{nk}$
,
$p_{nk}$
,
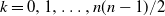 $k=0,1, \ldots, {n(n-1)}/{2}$
that the lines produce exactly k intersection points inside D. The objective is finding
$k=0,1, \ldots, {n(n-1)}/{2}$
that the lines produce exactly k intersection points inside D. The objective is finding
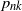 $p_{nk}$
through geometric invariants of D. Using Ambartzumian’s combinatorial algorithm, the known results are instantly reestablished for
$p_{nk}$
through geometric invariants of D. Using Ambartzumian’s combinatorial algorithm, the known results are instantly reestablished for
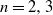 $n=2, 3$
. When
$n=2, 3$
. When
 $n=4$
, these probabilities are expressed by new invariants of D. When D is a disc of radius r, the simplest forms of all invariants are found. The exact values of
$n=4$
, these probabilities are expressed by new invariants of D. When D is a disc of radius r, the simplest forms of all invariants are found. The exact values of
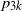 $p_{3k}$
and
$p_{3k}$
and
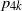 $p_{4k}$
are established.
$p_{4k}$
are established.