Article contents
Limit theorems for general size distributions
Published online by Cambridge University Press: 14 July 2016
Abstract
Let X1, X2, · ··, Xn, · ·· be independent and identically distributed non-negative integer-valued random variables with finite mean and variance. For any positive integer n and m we consider the random vector 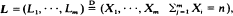 i.e., L has the same distribution as the conditional distribution of (X1, · ··, Xm) given the condition
i.e., L has the same distribution as the conditional distribution of (X1, · ··, Xm) given the condition 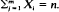 It is easy to see that our model includes the classical urn model, the Bose–Einstein urn model and the Pólya urn model as special cases. For any non-negative integer s define G(s) = the number of Li′s such that Li = s, and U = the number of Li′s such that Li is an even number; in this paper we study the asymptotic behaviour of the random variables considered above. Some central limit theorems and a multinormal local limit theorem are proved.
It is easy to see that our model includes the classical urn model, the Bose–Einstein urn model and the Pólya urn model as special cases. For any non-negative integer s define G(s) = the number of Li′s such that Li = s, and U = the number of Li′s such that Li is an even number; in this paper we study the asymptotic behaviour of the random variables considered above. Some central limit theorems and a multinormal local limit theorem are proved.
Keywords
- Type
- Research Papers
- Information
- Copyright
- Copyright © Applied Probability Trust
References
- 2
- Cited by


