Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-25T03:23:35.288Z Has data issue: false hasContentIssue false
  • English
  • Français

Inequalities for the M/G/∞ queue and related shot noise processes

Published online by Cambridge University Press:  14 July 2016

Fred W. Huffer
Fred W. Huffer*
Affiliation:
Florida State University
*
∗Postal address: Department of Statistics, Florida State University, Tallahassee, Florida 32306-3033, USA.
Get access Rights & Permissions [Opens in a new window]

Abstract

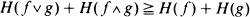Suppose that pulses arrive according to a Poisson process of rate λ with the duration of each pulse independently chosen from a distribution F having finite mean. Let X(t) be the shot noise process formed by the superposition of these pulses. We consider functionals H(X) of the sample path of X(t). H is said to be L-superadditive if for all functions f and g. For any distribution F for the pulse durations, we define H(F) = EH(X). We prove that if H is L-superadditive and 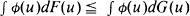for all convex functions ϕ, then 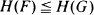. Various consequences of this result are explored.

Keywords

VARIABILITY ORDERINGCONVEX ORDERINGL-SUPERADDITIVE FUNCTIONALS
Type
Research Papers
Information
Journal of Applied Probability , Volume 24 , Issue 4 , December 1987 , pp. 978 - 989
Copyright
Copyright © Applied Probability Trust 1987 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Research supported by the Office of Naval Research under contract N00014-76-C-0475.

References

Brown, L. D. and Rinott, Y. Inequalities for multivariate infinitely divisible processes. (Submitted for publication).Google Scholar
Cox, D. R. and Isham, V. (1980) Point Processes. Chapman and Hall, London.Google Scholar
Hardy, G. H., Littlewood, J. E. and Pólya, G. (1952) Inequalities , 2nd edn. Cambridge University Press, London.Google Scholar
Huffer, F. W. (1984) Inequalities for the M/G/8 queue and related shot noise processes. Technical Report No. 351, Department of Statistics, Stanford University, Stanford, California.Google Scholar
Huffer, F. W. (1986) Variability orderings related to coverage problems on the circle. J. Appl. Prob. 23, 97–106.Google Scholar
Lorentz, G. G. (1953) An inequality for rearrangements. Amer. Math. Monthly. 60, 176–179.Google Scholar
Marshall, A. W. and Olkin, I. (1979) Inequalities: Theory of Majorization and Its Applications. Academic Press, New York.Google Scholar
Rolski, T. (1976) Order relations in the set of probability distribution functions and their applications in queueing theory. Diss. Math. No. 132.Google Scholar
Ross, S. (1983) Stochastic Processes. Wiley, New York.Google Scholar
Schmidt, V. (1985) Qualitative and asymptotic properties of shot-noise fields: A point process approach. Akademie der Wissenschaften der DDR, Institut für Mathematik, Berlin.Google Scholar
Slepian, D. (1962) The one-sided barrier problem for Gaussian noise. Bell System Tech. J. 41, 463–501.Google Scholar
Stoyan, D. (1977) Bounds and approximations in queueing through monotonicity and continuity. Operat. Res. 25, 851–863.Google Scholar
Stoyan, D. and (edited with revisions by) Daley, D. J. (1983) Comparison Methods for Queues and Other Stochastic Models. Wiley, New York.Google Scholar
Topkis, D. M. (1978) Minimizing a submodular function on a lattice. Operat. Res. 26, 305–321.Google Scholar