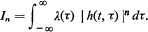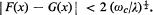Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Papoulis, A.
1972.
Narrow-band systems and Gaussianity.
IEEE Transactions on Information Theory,
Vol. 18,
Issue. 1,
p.
20.
Iglehart, Donald L.
1973.
Weak convergence in queueing theory.
Advances in Applied Probability,
Vol. 5,
Issue. 3,
p.
570.
Marcus, Allan H.
1973.
Traffic noise as a filtered Markov renewal process.
Journal of Applied Probability,
Vol. 10,
Issue. 02,
p.
377.
Andersen, Per K.
Andersen, Søren
and
Lauritzen, Steffen L.
1977.
The average noise from a Poisson stream of vehicles.
Journal of Applied Probability,
Vol. 14,
Issue. 4,
p.
817.
Tautu, P.
1980.
Modelle in der Medizin.
Vol. 22,
Issue. ,
p.
304.
Kotulski, Z.
and
Sobczyk, K.
1981.
Linear systems and normality.
Journal of Statistical Physics,
Vol. 24,
Issue. 2,
p.
359.
Orsingher, Enzo
and
Battaglia, Francesco
1982.
Probability Distributions and Level Crossings of Shot Noise Models.
Stochastics,
Vol. 8,
Issue. 1,
p.
45.
Szajnowski, WJ
1984.
Measurement of ion beam parameters with electrostatic induction electrodes.
Vacuum,
Vol. 34,
Issue. 1-2,
p.
285.
Heinrich, Lothar
and
Schmidt, Volker
1985.
Normal convergence of multidimensional shot noise and rates of this convergence.
Advances in Applied Probability,
Vol. 17,
Issue. 4,
p.
709.
Heinrich, Lothar
and
Schmidt, Volker
1985.
Normal convergence of multidimensional shot noise and rates of this convergence.
Advances in Applied Probability,
Vol. 17,
Issue. 4,
p.
709.
Szajnowski, W.J.
1985.
Extraction of information on a continuous ion beam from beam-induced shot noise.
Nuclear Instruments and Methods in Physics Research Section B: Beam Interactions with Materials and Atoms,
Vol. 6,
Issue. 1-2,
p.
176.
Lane, John A.
1987.
The Berry-Esseen bound for the Poisson shot-noise.
Advances in Applied Probability,
Vol. 19,
Issue. 2,
p.
512.
Schmidt, V.
1987.
Qualitative and asymptotic properties of stochastic integrals related to random marked point processes.
Optimization,
Vol. 18,
Issue. 5,
p.
737.
Snyder, Donald L.
and
Miller, Michael I.
1991.
Random Point Processes in Time and Space.
p.
215.
Sequeira, R.E.
Gubner, J.A.
and
Saleh, B.E.A.
1993.
Image detection under low-level illumination.
IEEE Transactions on Image Processing,
Vol. 2,
Issue. 1,
p.
18.
Ramaswami, Anuradha
and
Small, Mitchell J.
1994.
Modeling the spatial variability of natural trace element concentrations in groundwater.
Water Resources Research,
Vol. 30,
Issue. 2,
p.
269.
Hayat, M.M.
Saleh, B.E.A.
and
Gubner, J.A.
1995.
Bit-error rates for optical receivers using avalanche photodiodes with dead space.
IEEE Transactions on Communications,
Vol. 43,
Issue. 1,
p.
99.
Gubner, John A.
1996.
Computation of Shot-Noise Probability Distributions and Densities.
SIAM Journal on Scientific Computing,
Vol. 17,
Issue. 3,
p.
750.
Kotler, Gregory
Kontorovich, Valery
Lyandres, Vladimir
and
Primak, Sergey
1999.
On the Markov model of shot noise.
Signal Processing,
Vol. 75,
Issue. 1,
p.
79.
Hohn, Nicolas
and
Burkitt, Anthony N.
2001.
Shot noise in the leaky integrate-and-fire neuron.
Physical Review E,
Vol. 63,
Issue. 3,