Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-23T05:15:41.219Z Has data issue: false hasContentIssue false
  • English
  • Français

Some new results on stochastic comparisons of parallel systems

Part of: Nonparametric inference Survival analysis and censored data Distribution theory - Probability Sampling theory, sample surveys

Published online by Cambridge University Press:  14 July 2016

Baha-Eldin Khaledi  and
Subhash Kochar
Baha-Eldin Khaledi*
Affiliation:
Indian Statistical Institute
Subhash Kochar*
Affiliation:
Indian Statistical Institute
*
∗ Postal address: Indian Statistical Institute, 7 S. J. S. Sansanwal Marg, New Delhi 110016, India.
∗ Postal address: Indian Statistical Institute, 7 S. J. S. Sansanwal Marg, New Delhi 110016, India.
Get access Rights & Permissions [Opens in a new window]

Abstract

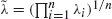Let X1,…,Xn be independent exponential random variables with Xi having hazard rate . Let Y1,…,Yn be a random sample of size n from an exponential distribution with common hazard rate ̃λ = (∏i=1nλi)1/n, the geometric mean of the λis. Let Xn:n = max{X1,…,Xn}. It is shown that Xn:n is greater than Yn:n according to dispersive as well as hazard rate orderings. These results lead to a lower bound for the variance of Xn:n and an upper bound on the hazard rate function of Xn:n in terms of 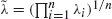. These bounds are sharper than those obtained by Dykstra et al. ((1997), J. Statist. Plann. Inference65, 203–211), which are in terms of the arithmetic mean of the λis. Furthermore, let X1*,…,Xn∗ be another set of independent exponential random variables with Xi∗ having hazard rate λi∗, i = 1,…,n. It is proved that if (logλ1,…,logλn) weakly majorizes (logλ1∗,…,logλn∗, then Xn:n is stochastically greater than Xn:n∗.

Keywords

Exponential distributionhazard rate orderingdispersive orderingSchur functionsmajorizationp-larger ordering

MSC classification

Primary: 60E15: Inequalities; stochastic orderings 62N05: Reliability and life testing 62G30: Order statistics; empirical distribution functions 62D05: Sampling theory, sample surveys
Type
Short Communications
Information
Journal of Applied Probability , Volume 37 , Issue 4 , December 2000 , pp. 1123 - 1128
Copyright
Copyright © by the Applied Probability Trust 2000 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Boland, P. J., El-Neweihi, E., and Proschan, F. (1994). Applications of the hazard rate ordering in reliability and order statistics. J. Appl. Prob. 31, 180–192.Google Scholar
Boland, P. J., Shaked, M., and Shanthikumar, J. G. (1998). Stochastic ordering of order statistics. In Order Statistics: Theory and Method (Handbook of Statistics 16), eds Balakrishnan, N. and Rao, C. R. North-Holland, Amsterdam, pp. 89–103.Google Scholar
Bon, J. L., and Paltanea, E. (1999). Ordering properties of convolutions of exponential random variables. Lifetime Data Anal. 5, 185–192.Google Scholar
Dykstra, R., Kochar, S. C., and Rojo, J. (1997). Stochastic comparisons of parallel systems of heterogeneous exponential components. J. Statist. Plann. Inference 65, 203–211.Google Scholar
Kochar, S. C., and Ma, C. (1999). Dispersive ordering of convolutions of exponential random variables. Statist. Prob. Lett. 43, 321–324 ((1999) Erratum ibid. 45, 283).CrossRefGoogle Scholar
Marshall, A. W., and Olkin, I. (1979). Inequalities : Theory of Majorization and its Applications. Academic Press, New York.Google Scholar
Mitrinović, D. S. (1970). Analytic Inequalities. Springer, Berlin.Google Scholar
Pledger, P., and Proschan, F. (1971). Comparisons of order statistics and of spacings from heterogeneous distributions. In Optimizing Methods in Statistics, ed. Rustagi, J. S. Academic Press, New York, pp. 89–113.Google Scholar
Proschan, F., and Sethuraman, J. (1976). Stochastic comparisons of order statistics from heterogeneous populations, with applications in reliability. J. Multivar. Anal. 6, 608–616.Google Scholar
Shaked, M., and Shanthikumar, J. G. (1994). Stochastic Orders and their Applications. Academic Press, New York.Google Scholar