No CrossRef data available.
Article contents
Skew brownian motion and complexity of the alps algorithm
Published online by Cambridge University Press: 12 July 2022
Abstract
Simulated tempering is a popular method of allowing Markov chain Monte Carlo algorithms to move between modes of a multimodal target density 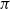 $\pi$
. Tawn, Moores and Roberts (2021) introduces the Annealed Leap-Point Sampler (ALPS) to allow for rapid movement between modes. In this paper we prove that, under appropriate assumptions, a suitably scaled version of the ALPS algorithm converges weakly to skew Brownian motion. Our results show that, under appropriate assumptions, the ALPS algorithm mixes in time
$\pi$
. Tawn, Moores and Roberts (2021) introduces the Annealed Leap-Point Sampler (ALPS) to allow for rapid movement between modes. In this paper we prove that, under appropriate assumptions, a suitably scaled version of the ALPS algorithm converges weakly to skew Brownian motion. Our results show that, under appropriate assumptions, the ALPS algorithm mixes in time  $O(d [\log d]^2)$
or O(d), depending on which version is used.
$O(d [\log d]^2)$
or O(d), depending on which version is used.
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press on behalf of Applied Probability Trust



