Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-16T19:20:54.440Z Has data issue: false hasContentIssue false
  • English
  • Français

Signatures of indirect majority systems

Part of: Survival analysis and censored data Special processes Decision theory

Published online by Cambridge University Press:  14 July 2016

Philip J. Boland
Philip J. Boland*
Affiliation:
National University of Ireland, Dublin
*
∗ Postal address: Department of Statistics, University College Dublin, Belfield, Dublin 4, Ireland. Email address: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

If τ is the lifetime of a coherent system, then the signature of the system is the vector of probabilities that the lifetime coincides with the ith order statistic of the component lifetimes. The signature can be useful in comparing different systems. In this treatment we give a characterization of the signature of a system with independent identically distributed components in terms of the number of path sets in the system as well as in terms of the number of what we call ordered cut sets. We consider, in particular, the signatures of indirect majority systems and compare them with the signatures of simple majority systems of the same size. 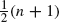We note that the signature of an indirect majority system of size r × s = n is symmetric around , and use this to show that the expected lifetime of an r × s = n indirect majority system exceeds that of a simple (direct) majority system of size n when the components are exponentially distributed with the same parameter.

Keywords

system reliabilitysignature of a coherent systemorder statisticssimple and indirect majority systems

MSC classification

Primary: 62C05: General considerations
Secondary: 60K10: Applications (reliability, demand theory, etc.) 62N05: Reliability and life testing
Type
Short Communications
Information
Journal of Applied Probability , Volume 38 , Issue 2 , June 2001 , pp. 597 - 603
Copyright
Copyright © Applied Probability Trust 2001 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Barlow, R., and Proschan, F. (1966). Inequalities for linear combinations of order statistics from restricted families. Ann. Math. Statist. 37, 1574–1592.Google Scholar
Barlow, R., and Proschan, F. (1981). Statistical Theory of Reliability and Life Testing: Probability Models. To Begin With, Silver Spring, MD.Google Scholar
Boland, P. J. (1989). Majority systems and the Condorcet jury theorem. Statistician 38, 181–189.Google Scholar
Boland, P. J., Proschan, F., and Tong, Y. L. (1989). Modelling dependence in simple and indirect majority systems. J. Appl. Prob. 26, 81–88.Google Scholar
David, H. A., and Groeneveld, R. A. (1982). Measures of local variation in a distribution: expected lengths of spacings and variances of order statistics. Biometrika 69, 227–232.Google Scholar
Kirmani, S., and Kochar, S. (1995). Some new results on spacings from restricted families of distributions. J. Statist. Plann. Inf. 46, 47–57.Google Scholar
Kochar, S., Mukerjee, H., and Samaniego, F. (1999). The signature of a coherent system and its application to comparisons among systems. Naval Res. Logist. 46, 507–523.Google Scholar
Samaniego, F. (1985). On closure of the IFR class under formation of coherent systems. IEEE Trans Rel. 34, 69–72.Google Scholar