Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Tanikawa, Akio
1999.
Geometric ergodicity of nonlinear first order autoregressive models.
Communications in Statistics. Stochastic Models,
Vol. 15,
Issue. 2,
p.
227.
Tanikawa, Akio
2001.
MARKOV CHAINS SATISFYING SIMPLE DRIFT CONDITIONS FOR SUBGEOMETRIC ERGODICITY.
Stochastic Models,
Vol. 17,
Issue. 2,
p.
109.


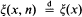 depends only on x. Sufficient conditions on moments of |
depends only on x. Sufficient conditions on moments of |