Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Levikson, Benny
1977.
The age distribution of Markov processes.
Journal of Applied Probability,
Vol. 14,
Issue. 3,
p.
492.
Sawyer, Stanley
1977.
On the past history of an allele now known to have frequencyp.
Journal of Applied Probability,
Vol. 14,
Issue. 3,
p.
439.
Pakes, Anthony G.
1978.
On the maximum and absorption time of left-continuous random walk.
Journal of Applied Probability,
Vol. 15,
Issue. 02,
p.
292.
Pakes, Anthony G.
1979.
The age of a Markov process.
Stochastic Processes and their Applications,
Vol. 8,
Issue. 3,
p.
277.
Cohn, H.
1979.
Harmonic functions for a class of Markov chains.
Journal of the Australian Mathematical Society,
Vol. 28,
Issue. 4,
p.
413.
Tavaré, S.
1979.
Time reversion and age distributions.
Advances in Applied Probability,
Vol. 11,
Issue. 1,
p.
10.
Tavaré, S.
1979.
Dual diffusions, killed diffusions, and the age distribution problem in population genetics.
Theoretical Population Biology,
Vol. 16,
Issue. 3,
p.
253.
Tavaré, S.
1979.
Time reversion and age distributions.
Advances in Applied Probability,
Vol. 11,
Issue. 1,
p.
10.
Tavaré, S.
1980.
Time reversal and age distributions, I. Discrete-time Markov chains.
Journal of Applied Probability,
Vol. 17,
Issue. 1,
p.
33.
Tavaré, S.
1980.
Time reversal and age distributions, I. Discrete-time Markov chains.
Journal of Applied Probability,
Vol. 17,
Issue. 1,
p.
33.
Kulkarni, M. V.
and
Pakes, A. G.
1983.
The total progeny of a simple branching process with state-dependent immigration.
Journal of Applied Probability,
Vol. 20,
Issue. 03,
p.
472.
Mitov, K. V.
Vatutin, V. A.
and
Yanev, N. M.
1984.
Continuous-time branching processes with decreasing state-dependent immigration.
Advances in Applied Probability,
Vol. 16,
Issue. 4,
p.
697.
Mitov, K. V.
and
Yanev, N. M.
1984.
Critical Galton–Watson processes by decreasing state-dependent immigration.
Journal of Applied Probability,
Vol. 21,
Issue. 1,
p.
22.
Mitov, K. V.
and
Yanev, N. M.
1985.
Bellman–Harris branching processes with state-dependent immigration.
Journal of Applied Probability,
Vol. 22,
Issue. 04,
p.
757.
Griffiths, R. C.
and
Pakes, Anthony G.
1988.
An infinite-alleles version of the simple branching process.
Advances in Applied Probability,
Vol. 20,
Issue. 3,
p.
489.
Mitov, K. V.
and
Yanev, N. M.
1989.
Bellman-Harris branching processes with a special type of state-dependent immigration.
Advances in Applied Probability,
Vol. 21,
Issue. 2,
p.
270.
Vatutin, V. A.
and
Zubkov, A. M.
1993.
Branching processes. II.
Journal of Soviet Mathematics,
Vol. 67,
Issue. 6,
p.
3407.
1993.
Correction.
Journal of Applied Probability,
Vol. 30,
Issue. 02,
p.
496.
Chen, Anyue
and
Renshaw, Eric
1995.
Markov branching processes regulated by emigration and large immigration.
Stochastic Processes and their Applications,
Vol. 57,
Issue. 2,
p.
339.
Mitov, Kosto V.
1995.
Branching Processes.
Vol. 99,
Issue. ,
p.
47.
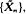 obtained by restarting the original chain at a fixed state after each absorption. The limiting age, A(j), is the weak limit of the time
obtained by restarting the original chain at a fixed state after each absorption. The limiting age, A(j), is the weak limit of the time 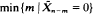 given Xn = j (n → ∞).
given Xn = j (n → ∞).

